Giải phương trình sau: cos 3 x + 3 cos 2 x + 2 cos x = 0
![]()
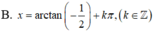
![]()
![]()
Giải phương trình sau: cos(x + 30o) = √3/2
√3/2 = cos30o nên cos(x + 30o )= √3/2
⇔ cos(x + 30o ) = cos 30o
⇔ x + 30o = ±30o + k360o, k ∈ Z
⇔ x = k360o, k ∈ Z và x = -60o + k360o, k ∈ Z
Giải phương trình sau: cos(x - 1) = 2/3
giải phương trình sin^2 x − 4√3 sin x · cos x + cos^2 x = −2.
Với \(cosx=0\) ko phải nghiệm
Với \(cosx\ne0\) chia 2 vế cho \(cos^2x\)
\(\Rightarrow tan^2x-4\sqrt{3}tanx+1=-2\left(1+tan^2x\right)\)
\(\Leftrightarrow3tan^2x-4\sqrt{3}tanx+3=0\)
\(\Rightarrow\left[{}\begin{matrix}tanx=\sqrt{3}\\tanx=\dfrac{\sqrt{3}}{3}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3}+k\pi\\x=\dfrac{\pi}{6}+k\pi\end{matrix}\right.\)
Giải phương trình :
\(3^{\cos x}-2^{\cos x}=\cos x\)
Xét hàm số \(f\left(t\right)=t^{\cos\alpha}-t\cos\alpha\)
Ta có : \(f'\left(x\right)=\left(t^{\cos\alpha}-1\right)\cos\alpha\)
Khi đó \(f\left(3\right)=f\left(2\right)\) và \(f\left(1\right)\) khả vi liên tục trên \(\left[2;3\right]\) Theo định lí Lagrange thì tồn tại \(c\in\left[2;3\right]\) sao cho :
\(f'\left(c\right)=\frac{f\left(3\right)-f\left(2\right)}{3-2}\) hay \(\left(c^{\cos\alpha-1}-1\right)\cos\alpha\)
Từ đó suy ra :
\(\begin{cases}\cos\alpha=0\\\cos\alpha=1\end{cases}\)\(\Leftrightarrow\begin{cases}\alpha=\frac{\pi}{2}+k\pi\\\alpha=k\pi\end{cases}\) \(\left(k\in Z\right)\)
Thử lại ta thấy các giá trị này đều thỏa mãn
Vậy nghiệm của phương trình là \(x=\frac{\pi}{2}+k\pi;x=k\pi\) và \(\left(k\in Z\right)\)
Trong các phương trình sau: cos x = 5 - 3 (1); sin x = 1 - 2 (2); sin x + cos x = 2 (3), phương trình nào vô nghiệm?
A. (2)
B. (1)
C. (3)
D. (1) và (2)
Trong các phương trình sau: cos x = 5 - 3 (1); sin x = 1 - 2 (2); sin x + cos x = 2 (3), phương trình nào vô nghiệm?
A. (2).
B. (1).
C. (3).
D. (1) và (2).
Chọn C
Ta có: ![]() nên (1) và (2) có nghiệm.
nên (1) và (2) có nghiệm.
Cách 1:
Xét: ![]()
![]() nên (3) vô nghiệm.
nên (3) vô nghiệm.
Cách 2:
Điều kiện có nghiệm của phương trình: sin x + cos x = 2 là: 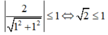
(vô lý) nên (3) vô nghiệm.
Cách 3:
Vì 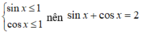
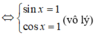
nên (3) vô nghiệm.
Giải phương trình sau:
\(Cos\left(2x+\dfrac{\pi}{3}\right)+Cos\left(x-\dfrac{\pi}{3}\right)=0\)
\(cos\left(2x+\dfrac{\pi}{3}\right)+cos\left(x-\dfrac{\pi}{3}\right)=0\)
\(\Leftrightarrow2cos\dfrac{3x}{2}.cos\left(\dfrac{x}{2}+\dfrac{\pi}{3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos\dfrac{3x}{2}=0\\cos\left(\dfrac{x}{2}+\dfrac{\pi}{3}\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{3x}{2}=\dfrac{\pi}{2}+k\pi\\\dfrac{x}{2}+\dfrac{\pi}{3}=\dfrac{\pi}{2}+k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3}+\dfrac{k2\pi}{3}\\x=\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
Giải các phương trình sau :
a) \(\cos^2x+2\sin x\cos x+5\sin^2x=2\)
b) \(3\cos^2x-2\sin2x+\sin^2x=1\)
c) \(4\cos^2x-3\sin x\cos x+3\sin^2x=1\)
Giải phương trình
(1+cos(x))*(2+4^cos(x)) = 3 * 4^cos(x)
mn giúp mình với !!!! HELP !
đề nè
\(\left(1+cosx\right)\cdot\left(1+4^{cosx}\right)=3\cdot4^{cosx}\)
Giải phương trình sau: \(\sin^22x-2\cos x+\dfrac{3}{4}=0\)