ĐK: \(x\ne\dfrac{\pi}{6}+\dfrac{k\pi}{3}\)

\(tan3x=tanx\)

\(\Leftrightarrow3x=x+k\pi\)

\(\Leftrightarrow x=\dfrac{k\pi}{2}\)

Đối chiếu điều kiện ta được \(x=k\pi\) là nghiệm của phương trình.

Lời giải:

$\tan 3x-\tan x=2$

$\Leftrightarrow \frac{3\tan x-\tan ^3x}{1-3\tan ^2x}-\tan x=2$

Đặt $\tan x=a$ thì:

$\frac{3a-a^3}{1-3a^2}-a=2$
$\Leftrightarrow a^3+3a^2+a-1=0$

$\Leftrihgtarrow a^2(a+1)+2a(a+1)-(a+1)=0$
$\Leftrightarrow (a+1)(a^2+2a-1)=0$

$\Leftrightarrow a=-1$ hoặc $a=-1\pm \sqrt{2}$

Đến đây thì đơn giản rồi.

ĐKXĐ: \(\left\{{}\begin{matrix}x\ne\dfrac{\pi}{2}+k\pi\\x\ne\dfrac{\pi}{6}+\dfrac{k\pi}{3}\end{matrix}\right.\)

\(\dfrac{sin3x}{cos3x}-\dfrac{sinx}{cosx}=2\)

\(\Rightarrow sin3x.cosx-cos3x.sinx=2cos3x.cosx\)

\(\Leftrightarrow sin2x=cos4x-cos2x\)

\(\Leftrightarrow cos^22x-sin^22x-sin2x-cos2x=0\)

\(\Leftrightarrow\left(sin2x+cos2x\right)\left(cos2x-sin2x-1\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}\sqrt{2}sin\left(2x+\dfrac{\pi}{4}\right)=0\\cos\left(2x+\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\end{matrix}\right.\)

\(\Leftrightarrow...\)

Giải theo công thức tan(x+2x)=(tanx+tan2x)/(1-tanx.tan2x) có vẻ nhanh hơn đó. 

Nhưng nhớ phải đặt điều kiện cho 3 cái cos dưới mẫu khác 0 (đk riêng của pt lượng giác)

Giải phương trình : sin5x-sin3x=0

\(tan\left(\dfrac{x}{2}\right)=\sqrt{3}\)

\(\Leftrightarrow\dfrac{x}{2}=\dfrac{\pi}{3}+k\pi\)

\(\Leftrightarrow x=\dfrac{2\pi}{3}+k2\pi\) (\(k\in Z\))

a/ ĐKXĐ: \(sin4x\ne0\)

\(\frac{sinx}{cosx}+\frac{cos2x}{sin2x}=\frac{2cos4x}{sin4x}\)

\(\Leftrightarrow2sin^2x.cos2x+2cos^22x=2cos4x\)

\(\Leftrightarrow\left(1-cos2x\right)cos2x+2cos^22x=4cos^22x-2\)

\(\Leftrightarrow3cos^22x-cos2x-2=0\)

\(\Leftrightarrow\left[{}\begin{matrix}cos2x=1\left(l\right)\\cos2x=-\frac{2}{3}\end{matrix}\right.\)

\(\Leftrightarrow2x=\pm arccos\left(-\frac{2}{3}\right)+k2\pi\)

\(\Leftrightarrow x=\pm\frac{1}{2}arccos\left(-\frac{2}{3}\right)+k\pi\)

b/ ĐKXĐ: \(cosx\ne0\)

\(\Leftrightarrow\left(1-\frac{sinx}{cosx}\right)\left(1+sinx\right)=1+\frac{sinx}{cosx}\)

\(\Leftrightarrow\left(cosx-sinx\right)\left(1+sinx\right)=sinx+cosx\)

\(\Leftrightarrow cosx+sinx.cosx-sinx-sin^2x=sinx+cosx\)

\(\Leftrightarrow sin^2x+2sinx-sinx.cosx=0\)

\(\Leftrightarrow sinx\left(sinx-cosx+2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\Rightarrow x=k\pi\\sinx-cosx=-2\left(1\right)\end{matrix}\right.\)

Xét \(\left(1\right)\Leftrightarrow\sqrt{2}sin\left(x-\frac{\pi}{4}\right)=-2\)

\(\Leftrightarrow sin\left(x-\frac{\pi}{4}\right)=-\sqrt{2}< -1\) (vô nghiệm)

\(tanx=-tan\dfrac{\pi}{5}\)

\(\Leftrightarrow tanx=tan\left(-\dfrac{\pi}{5}\right)\)

\(\Leftrightarrow x=-\dfrac{\pi}{5}+k\pi\)

Mình quên mất, nó nằm trong khoảng (π/2; π) nha, mình xin lỗi

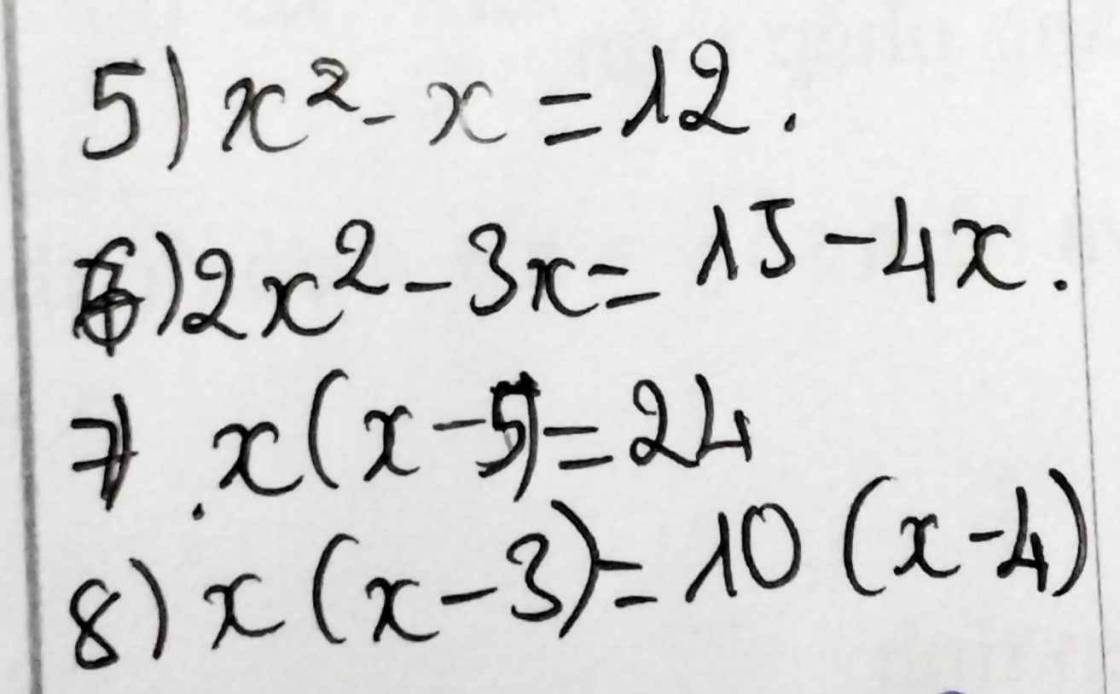
`x^2 -x=12`

`<=>x^2 -x-12=0`

`<=> x^2+3x-4x-12=0`

`<=> x(x+3)-4(x+3)=0`

`<=>(x+3)(x-4)=0`

\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=4\end{matrix}\right.\)

`---`

`2x^2-3x=15-4x`

`<=> 2x^2-3x+4x=15`

`<=>2x^2 +x-15=0`

`<=>2x^2+6x-5x-15=0`

`<=> 2x(x+3)-5(x+3)=0`

`<=>(x+3)(2x-5)=0`

\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\2x-5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=\dfrac{5}{2}\end{matrix}\right.\)

`---`

`x(x-5)=24`

`<=> x^2 -5x-24=0`

`<=>x^2+3x-8x-24=0`

`<=>x(x+3) -8(x+3)=0`

`<=>(x+3)(x-8)=0`

\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-8=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=8\end{matrix}\right.\)

`----`

`x(x-3)=10(x-4)`

`<=> x^2 -3x =10x -40`

`<=>x^2 -3x-10x +40=0`

`<=> x^2 -13x+40=0`

`<=>x^2-5x-8x+40=0`

`<=> x (x-5) - 8(x-5)=0`

`<=>(x-5)(x-8)=0`

\(\Leftrightarrow\left[{}\begin{matrix}x-5=0\\x-8=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=8\end{matrix}\right.\)

5. \(x^2-x=12\Leftrightarrow x^2-x-12=0\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-3\end{matrix}\right.\)

6. \(2x^2-3x=15-4x\Leftrightarrow2x^2+x-15=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-3\end{matrix}\right.\)

7. \(x\left(x-5\right)=24\Leftrightarrow x^2-5x-24=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=8\\x=-3\end{matrix}\right.\)

8. \(x\left(x-3\right)=10\left(x-4\right)\Leftrightarrow x^2-3x=10x-40\)

\(\Leftrightarrow x^2-13x+40=0\Leftrightarrow\left[{}\begin{matrix}x=8\\x=5\end{matrix}\right.\)

\(\Leftrightarrow4x^2=x+2+2\sqrt{x+2}+1\)

\(\Leftrightarrow\left(\sqrt{x+2}+1\right)^2=\left(2x\right)^2\)

\(\Rightarrow\left[{}\begin{matrix}\sqrt{x+2}+1=2x\\\sqrt{x+2}+1=-2x\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}\sqrt{x+2}=2x-1\left(x\ge\dfrac{1}{2}\right)\\\sqrt{x+2}=-2x-1\left(x\le-\dfrac{1}{2}\right)\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x+2=4x^2-4x+1\left(x\ge\dfrac{1}{2}\right)\\x+2=4x^2+4x+1\left(x\le-\dfrac{1}{2}\right)\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{5+\sqrt{41}}{8}\\x=-1\end{matrix}\right.\)