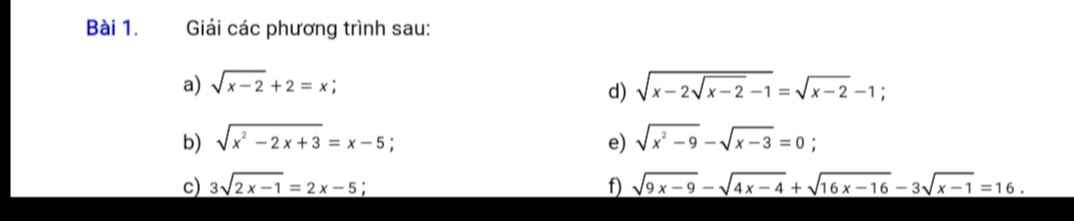
\(a,ĐK:x\ge2\\ PT\Leftrightarrow\sqrt{x-2}=x-2\\ \Leftrightarrow x-2=x^2-4x+4\\ \Leftrightarrow x^2-5x+6=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2\left(tm\right)\\x=3\left(tm\right)\end{matrix}\right.\\ b,ĐK:x\ge5\\ PT\Leftrightarrow x^2-2x+3=x^2-10x+25\\ \Leftrightarrow8x=22\Leftrightarrow x=\dfrac{11}{4}\left(ktm\right)\\ \Leftrightarrow x\in\varnothing\\ c,ĐK:x\ge\dfrac{1}{2}\\ PT\Leftrightarrow9\left(2x-1\right)=\left(2x-5\right)^2\\ \Leftrightarrow18x-9=4x^2-20x+25\\ \Leftrightarrow4x^2-38x+24=0\\ \Leftrightarrow2x^2-19x+12=0\\ \Delta=361-96=265\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{19-\sqrt{265}}{4}\left(tm\right)\\x=\dfrac{19+\sqrt{265}}{4}\left(tm\right)\end{matrix}\right.\)
\(d,ĐK:x\ge2\\ PT\Leftrightarrow\sqrt{\left(\sqrt{x-2}+1\right)^2}=\sqrt{x-2}+1\\ \Leftrightarrow\sqrt{x-2}+1-\sqrt{x-2}-1=0\\ \Leftrightarrow0=0\left(luôn.đúng\right)\Leftrightarrow x\in R;x\ge2\)
\(e,ĐK:x\ge3\\ PT\Leftrightarrow\sqrt{x-3}\left(\sqrt{x+3}-1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-3=0\\x+3=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\left(tm\right)\\x=2\left(ktm\right)\end{matrix}\right.\Leftrightarrow x=3\\ f,ĐK:x\ge1\\ PT\Leftrightarrow3\sqrt{x-1}-2\sqrt{x-1}+4\sqrt{x-1}-3\sqrt{x-1}=16\\ \Leftrightarrow\sqrt{x-1}=8\\ \Leftrightarrow x-1=64\Leftrightarrow x=65\left(tm\right)\)