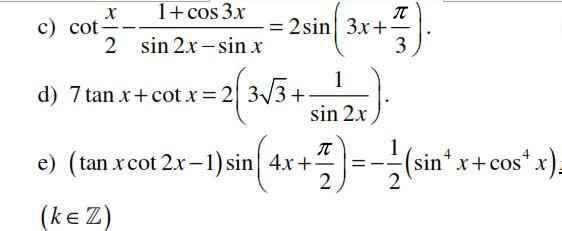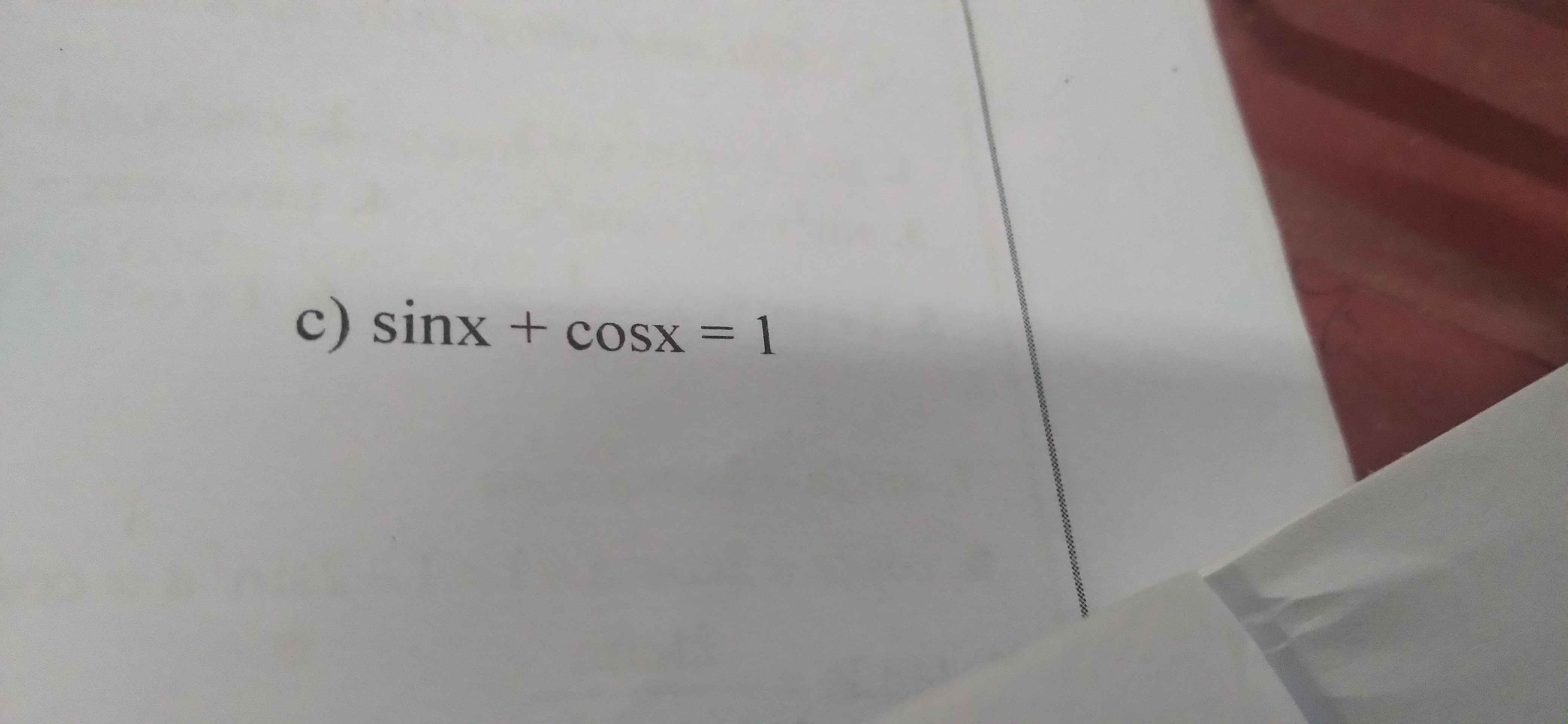a.
Đặt \(sinx+cosx=t\in\left[-\sqrt{2};\sqrt{2}\right]\)
\(\Rightarrow1+2sinx.cosx=t^2\Rightarrow2sinx.cosx=t^2-1\)
Phương trình trở thành:
\(3t=2\left(t^2-1\right)\)
\(\Leftrightarrow2t^2-3t-2=0\)
\(\Rightarrow\left[{}\begin{matrix}t=2>\sqrt{2}\left(loại\right)\\t=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Rightarrow sinx+cosx=-\dfrac{1}{2}\)
\(\Leftrightarrow\sqrt{2}sin\left(x+\dfrac{\pi}{4}\right)=-\dfrac{1}{2}\)
\(\Leftrightarrow sin\left(x+\dfrac{\pi}{4}\right)=-\dfrac{\sqrt{2}}{8}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{\pi}{4}=arcsin\left(-\dfrac{\sqrt{2}}{8}\right)+k2\pi\\x+\dfrac{\pi}{4}=\pi-arcsin\left(-\dfrac{\sqrt{2}}{8}\right)+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{4}+arcsin\left(-\dfrac{\sqrt{2}}{8}\right)+k2\pi\\x=\dfrac{3\pi}{4}-arcsin\left(-\dfrac{\sqrt{2}}{8}\right)+k2\pi\end{matrix}\right.\)
b.
ĐKXĐ: \(x\ne\dfrac{\pi}{2}+k\pi\)
\(1+\dfrac{sinx}{cosx}=2\sqrt{2}sinx\)
\(\Rightarrow sinx+cosx=2\sqrt{2}sinx.cosx\)
\(\Leftrightarrow\sqrt{2}sin\left(x+\dfrac{\pi}{4}\right)=\sqrt{2}sin2x\)
\(\Leftrightarrow sin\left(x+\dfrac{\pi}{4}\right)=sin2x\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=x+\dfrac{\pi}{4}+k2\pi\\2x=\dfrac{3\pi}{4}-x+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+k2\pi\\x=\dfrac{\pi}{4}+\dfrac{k2\pi}{3}\end{matrix}\right.\)
\(\Leftrightarrow x=\dfrac{\pi}{4}+\dfrac{k2\pi}{3}\)
c.
\(\Leftrightarrow1+sinx+cosx+sinx.cosx=2\)
\(\Leftrightarrow sinx+cosx+sinx.cosx=1\)
Đặt \(sinx+cosx=t\in\left[-\sqrt[]{2};\sqrt{2}\right]\)
\(\Rightarrow sinx.cosx=\dfrac{t^2-1}{2}\)
Phương trình trở thành:
\(t+\dfrac{t^2-1}{2}=1\)
\(\Leftrightarrow t^2+2t-3=0\Rightarrow\left[{}\begin{matrix}t=1\\t=-3\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow sinx+cosx=1\)
\(\Leftrightarrow\sqrt{2}sin\left(x+\dfrac{\pi}{4}\right)=1\)
\(\Leftrightarrow sin\left(x+\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow...\)
d.
Đặt \(\left|sinx-cosx\right|=t\in\left[0;\sqrt{2}\right]\)
\(\Rightarrow2sinx.cosx=1-t^2\)
\(\Leftrightarrow sin2x=1-t^2\)(1)
Phương trình trở thành:
\(t+4\left(1-t^2\right)=1\)
\(\Leftrightarrow4t^2-t-3=0\Rightarrow\left[{}\begin{matrix}t=1\\t=-\dfrac{3}{4}< 0\left(loại\right)\end{matrix}\right.\)
Thế vào (1)
\(\Rightarrow sin2x=0\)
\(\Rightarrow...\)
e.
ĐKXĐ: \(x\ne\dfrac{k\pi}{2}\)
\(\left(tan^2x+cot^2x+2\right)+7\left(tanx+cotx\right)+12=0\)
\(\Leftrightarrow\left(tanx+cotx\right)^2+7\left(tanx+cotx\right)+12=0\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx+cotx=-3\\tanx+cotx=-4\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx+\dfrac{1}{tanx}=-3\\tanx+\dfrac{1}{tanx}=-4\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tan^2x+3tanx+1=0\\tanx^2+4tanx+1=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tanx=\dfrac{-3\pm\sqrt{5}}{2}\\tanx=-2\pm\sqrt{3}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=arctan\left(\dfrac{-3\pm\sqrt{5}}{2}\right)+k\pi\\x=-\dfrac{\pi}{12}+k\pi\\x=-\dfrac{5\pi}{12}+k\pi\end{matrix}\right.\)
f.
ĐKXĐ: \(x\ne\dfrac{k\pi}{2}\)
\(\sqrt{3}\left(tan^2x+cot^2x-2\right)+2\left(\sqrt{3}-1\right)\left(tanx-cotx\right)-4=0\)
\(\Leftrightarrow\sqrt{3}\left(tanx-cotx\right)^2+2\left(\sqrt{3}-1\right)\left(tanx-cotx\right)-4=0\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx-cotx=-2\\tanx-cotx=\dfrac{2\sqrt{3}}{3}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx-\dfrac{1}{tanx}=-2\\tanx-\dfrac{1}{tanx}=\dfrac{2\sqrt{3}}{3}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tan^2x+2tanx-1=0\\3tan^2x-2\sqrt{3}tanx-3=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tanx=-1\pm\sqrt{2}\\tanx=\sqrt{3}\\tanx=-\dfrac{1}{\sqrt{3}}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{8}+k\pi\\x=-\dfrac{3\pi}{8}+k\pi\\x=\dfrac{\pi}{3}+k\pi\\x=-\dfrac{\pi}{6}+k\pi\end{matrix}\right.\)