Tập xác định: \(D = \mathbb{R}\backslash \{ 1\} \)
- Chiều biến thiên:
\(y' = \frac{{{x^2} - 2x - 3}}{{{{(x - 1)}^2}}} = 0 \Leftrightarrow \left[ \begin{array}{l}x = - 1\\x = 3\end{array} \right.\)
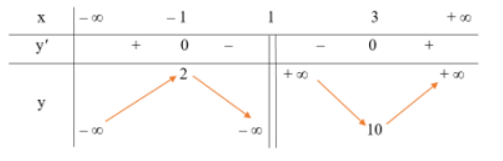
Trên các khoảng (\( - \infty \); -1), (3; \( + \infty \)) thì y' < 0 nên hàm số nghịch biến trên mỗi khoảng đó. Trên khoảng (-1; 3) thì y' > 0 nên hàm số đồng biến trên khoảng đó.
- Giới hạn và tiệm cận:
\(\mathop {\lim }\limits_{x \to + \infty } y = \mathop {\lim }\limits_{x \to + \infty } \frac{{{x^2} + 4x - 1}}{{x - 1}} = + \infty ;\mathop {\lim }\limits_{x \to - \infty } y = \mathop {\lim }\limits_{x \to - \infty } \frac{{{x^2} + 4x - 1}}{{x - 1}} = - \infty \)
\(a = \mathop {\lim }\limits_{x \to + \infty } \frac{{{x^2} + 4x - 1}}{{{x^2} - x}} = 1;b = \mathop {\lim }\limits_{x \to + \infty } (\frac{{{x^2} + 4x - 1}}{{x - 1}} - x) = 5\) nên y = x + 5 là tiệm cận xiên của đồ thị hàm số
\(\mathop {\lim }\limits_{x \to {1^ + }} y = \mathop {\lim }\limits_{x \to {1^ + }} \frac{{{x^2} + 4x - 1}}{{x - 1}} = + \infty ;\mathop {\lim }\limits_{x \to {1^ - }} y = \mathop {\lim }\limits_{x \to {1^ - }} \frac{{{x^2} + 4x - 1}}{{x - 1}} = - \infty \) nên x = 1 là tiệm cận đứng của đồ thị hàm số
Bảng biến thiên:
Ta có: \(y = 0 \Leftrightarrow \frac{{{x^2} + 4x - 1}}{{x - 1}} = 0 \Leftrightarrow \left[ \begin{array}{l}x = - 2 - \sqrt 5 \\x = - 2 + \sqrt 5 \end{array} \right.\)
Vậy đồ thị của hàm số giao với trục Ox tại điểm (\( - 2 - \sqrt 5 \); 0) và (\( - 2 + \sqrt 5 \); 0)
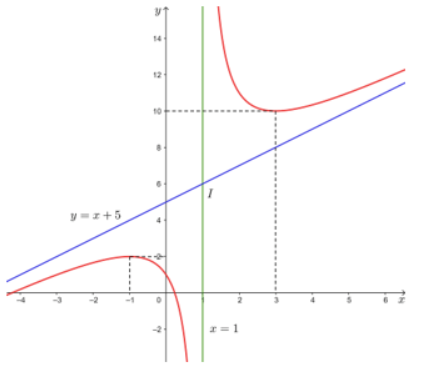
b) Bảng biến thiên:
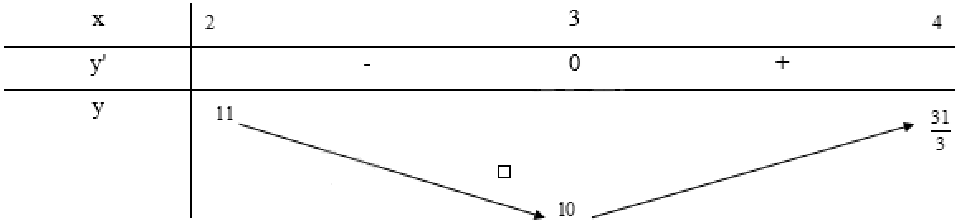
Từ bảng biến thiên, ta thấy \(\mathop {\min }\limits_{[2;4]} y = y(3) = 10\) và \(\mathop {\max }\limits_{[2;4]} y = y(2) = 11\)


