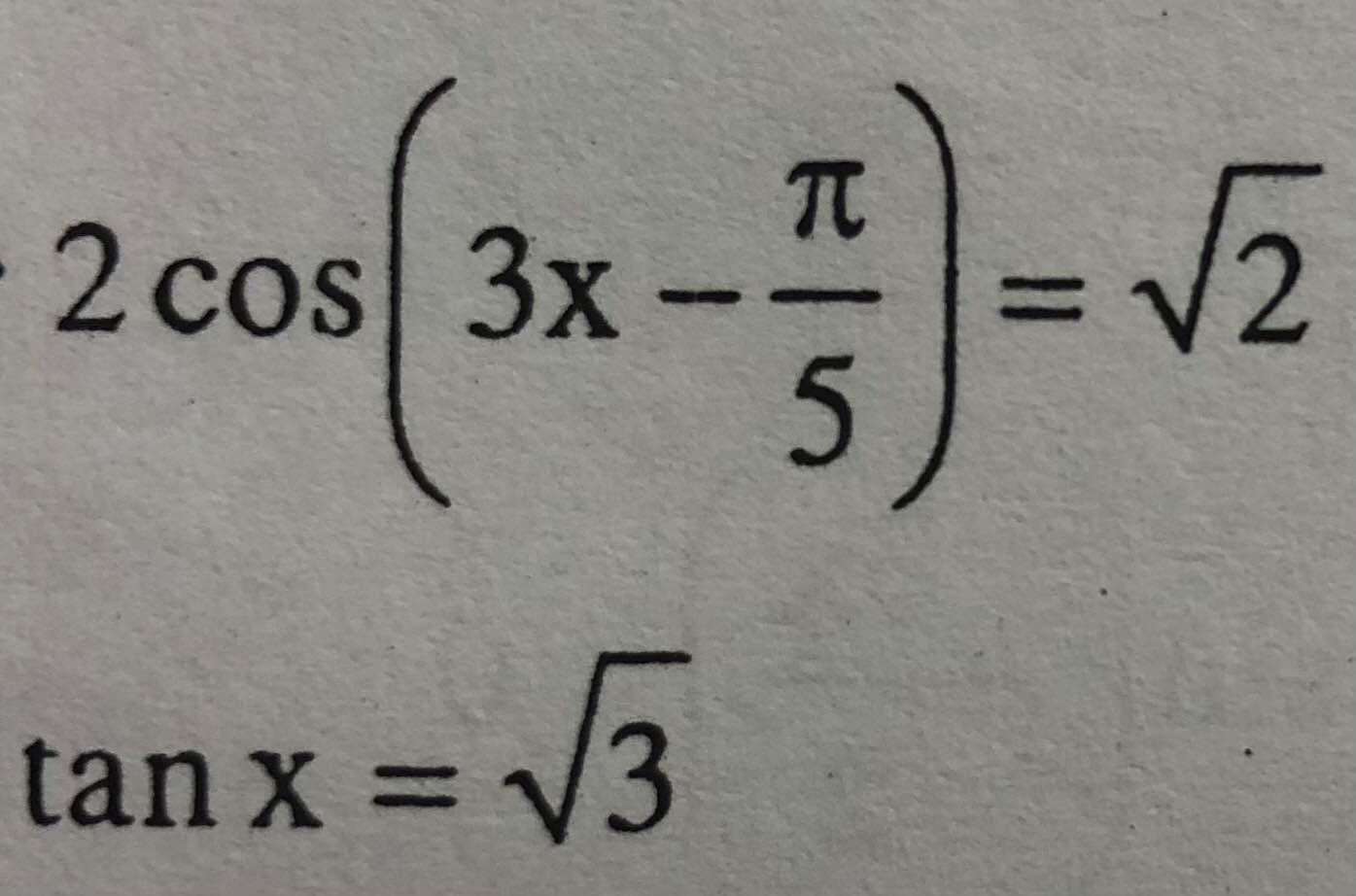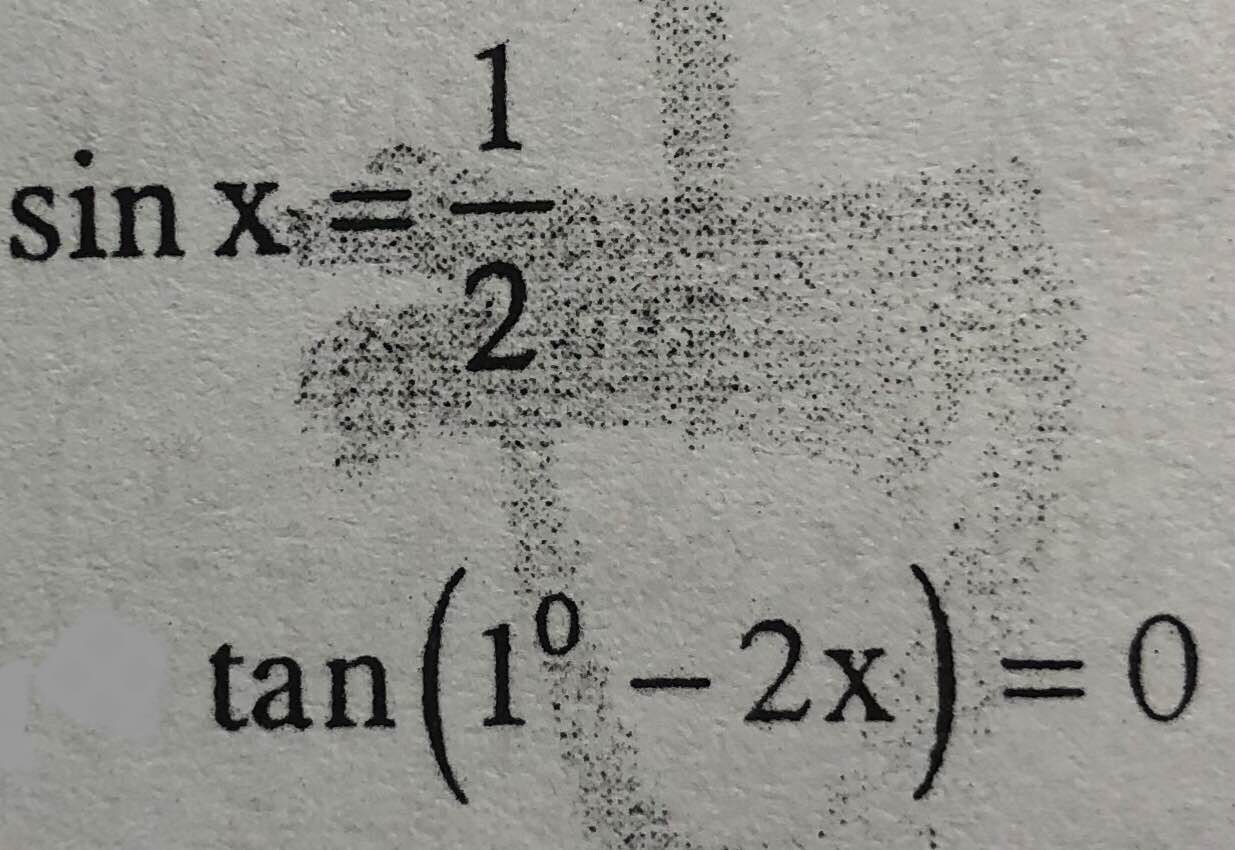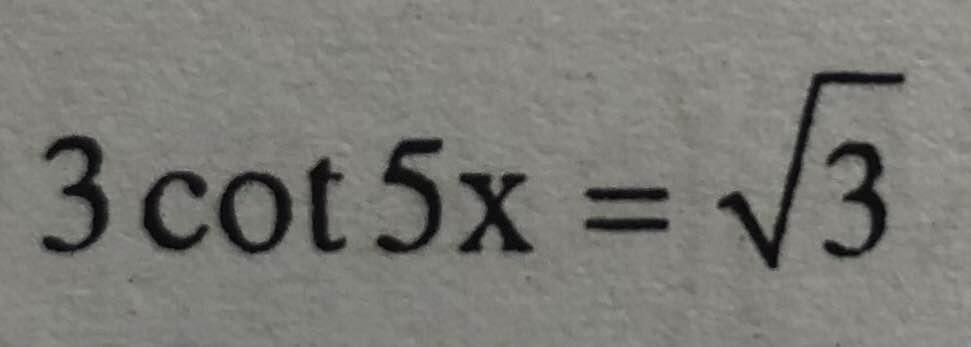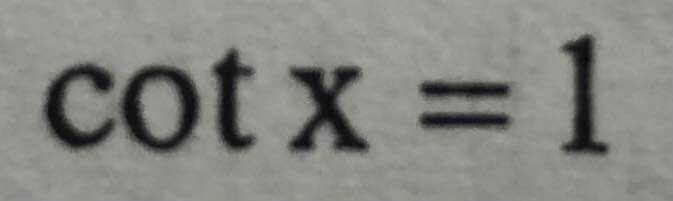a.
\(2cos\left(3x-\dfrac{\pi}{5}\right)=\sqrt{2}\)
\(\Leftrightarrow cos\left(3x-\dfrac{\pi}{5}\right)=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-\dfrac{\pi}{5}=\dfrac{\pi}{4}+k2\pi\\3x-\dfrac{\pi}{5}=-\dfrac{\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3x=\dfrac{9\pi}{20}+k2\pi\\3x=-\dfrac{\pi}{20}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3\pi}{20}+\dfrac{k2\pi}{3}\\x=-\dfrac{\pi}{60}+\dfrac{k2\pi}{3}\end{matrix}\right.\)
b.
\(tanx=\sqrt{3}\)
\(\Leftrightarrow tanx=tan\left(\dfrac{\pi}{3}\right)\)
\(\Leftrightarrow x=\dfrac{\pi}{3}+k\pi\)
c.
\(sinx=\dfrac{1}{2}\)
\(\Leftrightarrow sinx=sin\left(\dfrac{\pi}{6}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
d.
\(tan\left(1^0-2x\right)=0\)
\(\Leftrightarrow1^0-2x=k180^0\)
\(\Leftrightarrow2x=1^0+k180^0\)
\(\Leftrightarrow x=0,5^0+k90^0\)
e.
\(3cot5x=\sqrt{3}\)
\(\Leftrightarrow cot5x=\dfrac{1}{\sqrt{3}}\)
\(\Leftrightarrow5x=\dfrac{\pi}{3}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{15}+\dfrac{k\pi}{5}\)
f.
\(cotx=1\)
\(\Leftrightarrow x=\dfrac{\pi}{4}+k\pi\)