Đặt nhân tử chung rồi giản ước

Những câu hỏi liên quan
đặt thừa số chung rồi đơn giản biểu thức: ab-bd-be+ce+cd+ae
ab - bd - be + ce + cd + ae = (ab - be) - (bd - cd) + (ce + ae)
= b(a - e) - d(b - c) + e(c + a)
Xem lại đề nhé, hơi căng :))
Đúng 0
Bình luận (0)
Cho phân thức:
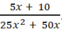
a) Phân tích tử và mẫu thành nhân tử rồi tìm nhân tử chung của chúng.
b) Chia cả tử và mẫu cho nhân tử chung.
a) 5x + 10 = 5(x + 2)
25x2 + 50x = 25x(x + 2)
⇒ Nhân tử chung của chúng là: 5(x + 2)
b)
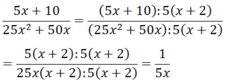
Đúng 0
Bình luận (0)
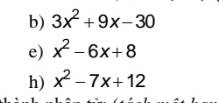
đặt nhân tử chung
h: =3(x^2+3x-10)
=3(x^2+5x-2x-10)
=3[x(x+5)-2(x+5)]
=3(x+5)(x-2)
e: =x^2-2x-4x+8
=x(x-2)-4(x-2)
=(x-2)(x-4)
h: =x^2-3x-4x+12
=x(x-3)-4(x-3)
=(x-3)(x-4)
Đúng 0
Bình luận (0)
Lời giải:
b. $3x^2+9x-30=3(x^2+3x-10)$
$=3[(x^2-2x)+(5x-10)]=3[x(x-2)+5(x-2)]=3(x-2)(x+5)$
e. $x^2-6x+8=(x^2-2x)-(4x-8)=x(x-2)-4(x-2)=(x-4)(x-2)$
h. $x^2-7x+12=(x^2-3x)-(4x-12)=x(x-3)-4(x-3)=(x-3)(x-4)$
Đúng 0
Bình luận (0)
phân tích đa thức thành nhân tử bằng phương pháp đặt nhân tử chung
Xem thêm câu trả lời
đặt nhân tử chung ạ
Đọc tiếp
đặt nhân tử chung ạ
\(=\left(3x+15\right)^2-\left(x-7\right)^2\\ =\left(3x+15-x+7\right)\left(3x+15+x-7\right)\\ =\left(2x+22\right)\left(4x+8\right)\\ =2\left(x+11\right)4\left(x+2\right)\\ =8\left(x+11\right)\left(x+2\right)\)
Đúng 2
Bình luận (0)
đặt nhân tử chung ạ
Đọc tiếp
đặt nhân tử chung ạ
\(=\left(x^2+y^2-5\right)^2-\left[2\left(xy+2\right)\right]^2\\ =\left(x^2+y^2-5-2xy-4\right)\left(x^2+y^2-5+2xy+4\right)\\ =\left[\left(x-y\right)^2-9\right]\left[\left(x+y\right)^2-1\right]\\ =\left(x-y-3\right)\left(x-y+3\right)\left(x+y-1\right)\left(x+y+1\right)\)
Đúng 2
Bình luận (0)
\(\left(x^2+y^2-5\right)^2-4\left(x^2y^2+4xy+4\right)\)
\(=\left(x^2+y^2-5\right)^2-4\left(xy+2\right)^2\)
\(=\left(x^2+y^2-5\right)^2-\left(2xy+4\right)^2=\left(x^2+y^2-5-2xy-4\right)\left(x^2+y^2-5+2xy+4\right)\)
\(=\left(x^2+y^2-2xy-9\right)\left(x^2+y^2+2xy-1\right)\)
\(=\left[\left(x-y\right)^2-9\right]\left[\left(x+y\right)^2-1\right]=\left(x-y-3\right)\left(x-y+3\right)\left(x+y-1\right)\left(x+y+1\right)\)
Đúng 2
Bình luận (0)
\(=\left(x^2+y^2-5\right)^2-4\left(xy+2\right)^2\)
\(=\left(x^2+y^2-5\right)^2-\left(2xy+4\right)^2\)
\(=\left(x^2+y^2-5-2xy-4\right)\left(x^2+y^2-5+2xy+4\right)\)
\(=\left[\left(x-y\right)^2-9\right]\left[\left(x-y\right)^2-1\right]\)
\(=\left(x-y-3\right)\left(x-y+3\right)\left(x-y-1\right)\left(x-y+1\right)\)
Đúng 2
Bình luận (0)
Phân tích đa thức thành nhân tử bằng phương pháp đặt nhân tử chung:
-x2-4xy-4y2
= \(-\left(x^2+4xy+4y^2\right)\)
= \(-\left(x+2y\right)^2\)
Đúng 1
Bình luận (0)
Biến đa thức thành nhân tử bằng phowung pháp đặt nhân tử chung.
3xy-21x2y2
3x2y-5xy2+7xy
\(3xy-21x^2y^2=3xy\left(1-7xy\right)\)
\(3x^2y-5xy^2+7xy\)
\(=xy\left(3x-5y+7\right)\)
Đúng 1
Bình luận (0)
\(3xy-21x^2y^2\\ =3xy-3xy.7xy\\ =3xy\left(1-7xy\right)\)
Cái thứ hai không phân tích bằng đặt nhân tử chung được đâu nha=)
Đúng 0
Bình luận (0)
đặt nhân tử chung 3x-6y



























