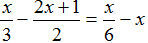\(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)

Những câu hỏi liên quan
Giải PT sau
\(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)
\(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)
\(\Leftrightarrow\dfrac{2+x}{5}-\dfrac{x}{2}=\dfrac{1-2x}{4}+\dfrac{1}{4}\)
\(\Leftrightarrow\dfrac{2+x}{5}-\dfrac{x}{2}=\dfrac{1-2x+1}{4}\)
\(\Leftrightarrow\dfrac{2+x}{5}-\dfrac{x}{2}=\dfrac{2-2x}{4}\)
\(\Leftrightarrow\dfrac{2+x}{5}=\dfrac{1-x}{2}+\dfrac{x}{2}\)
\(\Leftrightarrow\dfrac{2+x}{5}=\dfrac{1-x+x}{2}\)
\(\Leftrightarrow\dfrac{2+x}{5}=\dfrac{1}{2}\)
\(\Leftrightarrow2\left(2+x\right)=5\\ \Leftrightarrow2x+4-5=0\\ \Leftrightarrow2x-1=0\\ \Leftrightarrow x=\dfrac{1}{2}\)
Đúng 1
Bình luận (0)
\(PT.\Rightarrow\) \(\dfrac{8+4x-10x-5+10x-5}{20}=0.\Rightarrow4x=2.\Leftrightarrow x=\dfrac{1}{2}.\)
Đúng 1
Bình luận (0)
\(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\\ \Leftrightarrow\dfrac{4.\left(2+x\right)}{20}-\dfrac{20.0,5x}{20}=\dfrac{5.\left(1-2x\right)}{20}+\dfrac{0,25.20}{20}\\ \Leftrightarrow8+4x-10x=5-10x+5\\ \Leftrightarrow4x=2\\ \Leftrightarrow x=\dfrac{1}{2}\\ \Rightarrow S=\left\{\dfrac{1}{2}\right\}\)
Đúng 3
Bình luận (0)
Giải các phương trình sau:
\(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)
Giúp mình với ạ :333
Mãi iu mọi người ><
=>0,2x+0,4-0,5x=0,25-0,5x+0,25
=>0,2x+0,4=0,5
=>0,2x=0,1
=>x=1/2
Đúng 0
Bình luận (0)
Giải các phương trình :
a) \(\dfrac{x}{3}-\dfrac{2x+1}{2}=\dfrac{x}{6}-x\)
b) \(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)
a) \(\dfrac{x}{3}-\dfrac{2x+1}{2}=\dfrac{x}{6}-x\)
\(\Leftrightarrow\dfrac{2x}{6}-\dfrac{3\left(2x+1\right)}{6}=\dfrac{x}{6}=\dfrac{6x}{6}\)
\(\Leftrightarrow2x-3\left(2x+1\right)=x-6x\)
\(\Leftrightarrow2x-6x-3=x-6x\)
\(\Leftrightarrow2x-6x-x+6x=3\)
\(\Leftrightarrow x=3\)
\(S=\left\{3\right\}\)
b) \(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)
\(\Leftrightarrow\dfrac{4\left(2+x\right)}{20}-\dfrac{10x}{20}=\dfrac{5\left(1-2x\right)}{20}+\dfrac{5}{20}\)
\(\Leftrightarrow4\left(2+x\right)-10x=5\left(1-2x\right)+5\)
\(\Leftrightarrow8+4x-10x=5-10x+5\)
\(\Leftrightarrow4x-10x+10x=5+5-8\)
\(\Leftrightarrow4x=2\)
\(\Leftrightarrow x=\dfrac{1}{2}\)
\(S=\left\{\dfrac{1}{2}\right\}\)
Đúng 0
Bình luận (0)
giải pt sau
g) 11+8x-35x-3+x
h)4-2x+159x+4-2x
g)dfrac{3x+2}{2}-dfrac{3x+1}{6}dfrac{5}{3}+2x
h)dfrac{x+4}{5}-x+4dfrac{4x+2}{5}-5
i)dfrac{4x+3}{5}-dfrac{6x-2}{7}dfrac{5x+4}{3}+3
k) dfrac{5x+2}{6}-dfrac{8x-1}{3}dfrac{4x+2}{5}-5
m) dfrac{2x-1}{5}-dfrac{x-2}{3}dfrac{xx+7}{15}
n)dfrac{1}{4}left(x+3right)3-dfrac{1}{2}left(x+1right)-dfrac{1}{3}left(x+2right)
p)dfrac{x}{3}-dfrac{2x+1}{6}dfrac{x}{6}-x
q)dfrac{2+x}{5}-0,5xdfrac{1-2x}{4}+0,25
Đọc tiếp
giải pt sau
g) 11+8x-3=5x-3+x
h)4-2x+15=9x+4-2x
g)\(\dfrac{3x+2}{2}-\dfrac{3x+1}{6}=\dfrac{5}{3}+2x\)
h)\(\dfrac{x+4}{5}-x+4=\dfrac{4x+2}{5}-5\)
i)\(\dfrac{4x+3}{5}-\dfrac{6x-2}{7}=\dfrac{5x+4}{3}+3\)
k) \(\dfrac{5x+2}{6}-\dfrac{8x-1}{3}=\dfrac{4x+2}{5}-5\)
m) \(\dfrac{2x-1}{5}-\dfrac{x-2}{3}=\dfrac{xx+7}{15}\)
n)\(\dfrac{1}{4}\left(x+3\right)=3-\dfrac{1}{2}\left(x+1\right)-\dfrac{1}{3}\left(x+2\right)\)
p)\(\dfrac{x}{3}-\dfrac{2x+1}{6}=\dfrac{x}{6}-x\)
q)\(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)
giải pt sau
g) 11+8x-3=5x-3+x
\(\Leftrightarrow\) 8x + 8 = 6x - 3
<=> 8x-6x = -3 - 8
<=> 2x = -11
=> x=-\(\dfrac{11}{2}\)
Vậy tập nghiệm của PT là : S={\(-\dfrac{11}{2}\)}
h)4-2x+15=9x+4-2x
<=> 19 - 2x = 7x + 4
<=> -2x - 7x = 4 - 19
<=> -9x = -15
=> x=\(\dfrac{15}{9}=\dfrac{5}{3}\)
Vậy tập nghiệm của pt là : S={\(\dfrac{5}{3}\)}
g)\(\dfrac{3x+2}{2}-\dfrac{3x+1}{6}=\dfrac{5}{3}+2x\)
<=> \(\dfrac{3\left(3x+2\right)}{6}-\dfrac{3x+1}{6}=\dfrac{5.2+6.2x}{6}\)
<=> 9x + 6 - 3x + 1 = 10 + 12x
<=> 6x + 7 = 10 + 12x
<=> 6x -12x = 10-7
<=> -6x = 3
=> x= \(-\dfrac{1}{2}\)
Vậy tập nghiệm của PT là : S={\(-\dfrac{1}{2}\)}
\(h,\dfrac{x+4}{5}-x+4=\dfrac{4x+2}{5}-5\)
<=> \(\dfrac{x+4-5\left(x+4\right)}{5}=\dfrac{4x+2-5.5}{5}\)
<=> x + 4 - 5x - 20 = 4x + 2 - 25
<=> x - 5x - 4x = 2-25-4+20
<=> -8x = -7
=> x= \(\dfrac{7}{8}\)
Vậy tập nghiệm của PT là S={\(\dfrac{7}{8}\)}
\(i,\dfrac{4x+3}{5}-\dfrac{6x-2}{7}=\dfrac{5x+4}{3}+3\)
<=> \(\dfrac{21\left(4x+3\right)}{105}\)-\(\dfrac{15\left(6x-2\right)}{105}\)=\(\dfrac{35\left(5x+4\right)+3.105}{105}\)
<=> 84x + 63 - 90x + 30 = 175x + 140 + 315
<=> 84x - 90x - 175x = 140 + 315 - 63 - 30
<=> -181x = 362
=> x = -2
Vậy tập nghiệm của PT là : S={-2}
K) \(\dfrac{5x+2}{6}-\dfrac{8x-1}{3}=\dfrac{4x+2}{5}-5\)
<=> \(\dfrac{5\left(5x+2\right)}{30}-\dfrac{10\left(8x-1\right)}{30}=\dfrac{6\left(4x+2\right)-150}{30}\)
<=> 25x + 10 - 80x - 10 = 24x + 12 - 150
<=> -55x = 24x - 138
<=> -55x - 24x = -138
=> -79x = -138
=> x=\(\dfrac{138}{79}\)
Vậy tập nghiệm của PT là S={\(\dfrac{138}{79}\)}
m) \(\dfrac{2x-1}{5}-\dfrac{x-2}{3}=\dfrac{x+7}{15}\)
<=> \(\dfrac{3\left(2x-1\right)-5\left(x-2\right)}{15}=\dfrac{x+7}{15}\)
<=> 6x - 3 - 5x + 10 = x+7
<=> x + 7 = x+7
<=> 0x = 0
=> PT vô nghiệm
Vậy S=\(\varnothing\)
n)\(\dfrac{1}{4}\left(x+3\right)=3-\dfrac{1}{2}\left(x+1\right)-\dfrac{1}{3}\left(x+2\right)\)
<=> \(\dfrac{1}{4}x+\dfrac{3}{4}=3-\dfrac{1}{2}x-\dfrac{1}{2}-\dfrac{1}{3}x-\dfrac{2}{3}\)
<=> \(\dfrac{1}{4}x+\dfrac{1}{2}x+\dfrac{1}{3}x=3-\dfrac{1}{2}-\dfrac{2}{3}-\dfrac{3}{4}\)
<=> \(\dfrac{13}{12}x=\dfrac{13}{12}\)
=> x= 1
Vậy S={1}
p) \(\dfrac{x}{3}-\dfrac{2x+1}{6}=\dfrac{x}{6}-6\)
<=> \(\dfrac{2x-2x+1}{6}=\dfrac{x-36}{6}\)
<=> 2x -2x + 1= x-36
<=> 2x-2x-x = -37
=> x = 37
Vậy S={37}
q) \(\dfrac{2+x}{5}-0,5x=\dfrac{1-2x}{4}+0,25\)
<=> \(\dfrac{4\left(2+x\right)-20.0,5x}{20}=\dfrac{5\left(1-2x\right)+20.0,25}{20}\)
<=> 8 + 4x - 10x = 5 - 10x + 5
<=> 4x-10x + 10x = 5+5-8
<=> 4x = 2
=> x= \(\dfrac{1}{2}\)
Vậy S={\(\dfrac{1}{2}\)}
Đúng 0
Bình luận (10)
g) \(11+8x-3=5x-3+x\)
\(\Leftrightarrow8+8x=6x-3\)
\(\Leftrightarrow8x-6x=-3-8\)
\(\Leftrightarrow2x=-11\)
\(\Leftrightarrow x=-\dfrac{11}{2}\)
h, \(4-2x+15=9x+4-2x\)
\(\Leftrightarrow-2x-9x+2x=4-4-15\)
\(\Leftrightarrow-9x=-15\)
\(\Leftrightarrow x=\dfrac{-15}{-9}=\dfrac{5}{3}\)
Đúng 0
Bình luận (0)
g) 11+8x-3=5x-3+x
=> 8x -5x -x = -3 -11+3
<=> 2x = -11
<=> x = \(\dfrac{-11}{2}\)
h)4-2x+15=9x+4-2x
=> -2x -9x +2x = 4-4-15
<=> -9x = -15
<=> x = \(\dfrac{5}{3}\)
Đúng 0
Bình luận (0)
Thực hiện phép tính
\(B=(\dfrac{1}{3}-0,25-\dfrac{1}{12}).(4\dfrac{5}{7}-121\%+191)\)
Tìm x
\(x+30\%=-1,3\)
\(0,5x-\dfrac{2}{3}x=\dfrac{7}{12}\)
\(\dfrac{x}{5}=\dfrac{x+6}{15}\)
Tìm x:
a, x+30%=-1,3
<=> x+0,3=-1,3
<=> x=-1,3-0,3
<=> x=-1,6
Vậy x=-1,6
b, 0,5x-\(\dfrac{2}{3}x\) =\(\dfrac{7}{12}\)
<=>\(\dfrac{-1}{6}x\)=\(\dfrac{7}{12}\)
<=> x=\(\dfrac{-7}{2}\)
Vậy x=\(\dfrac{-7}{2}\)
Đúng 0
Bình luận (0)
Tìm x
\(\dfrac{3}{4}x+\dfrac{-1}{2}=\dfrac{-13}{8}\)
\(\left|x\right|+0,25=1,75.3\)
\(\dfrac{2x-1}{3}=\dfrac{-5}{0,6}\)
a, \(\dfrac{3}{4}x=-\dfrac{9}{8}\)
x= \(-\dfrac{3}{2}\)
b, |x| + 0,25= 5,25
|x | = 5
=> x\(\in\){ +- 5}
Ko chắc đúng, kiểm tra trc khi làm
Đúng 0
Bình luận (0)
\(\dfrac{2x-1}{3}=\dfrac{-5}{0.6}\)
\(\Leftrightarrow2x-1=-25\)
hay x=-12
Đúng 0
Bình luận (0)
Tìm x biết :
1) \(\dfrac{-7}{x+1}=\dfrac{-x-1}{\dfrac{4}{7}}\)
2) \(\dfrac{x-3}{x+5}=\dfrac{5}{7}\)
3) \(\dfrac{x+1}{2x+1}=\dfrac{0,5x+2}{x+3}\)
4) \(\dfrac{3x+2}{5x+7}=\dfrac{3x-1}{5x+1}\)
1: \(\Leftrightarrow\left(x+1\right)^2=4\)
=>x+1=2 hoặc x+1=-2
=>x=1 hoặc x=-3
2: \(\Leftrightarrow7x-21=5x+25\)
=>2x=46
=>x=23
3: \(\Leftrightarrow x^2+4x+3=x^2+0.5x+4x+2\)
=>4,5x+2=4x+3
=>x=1
Đúng 0
Bình luận (0)
a\(\dfrac{-3}{4}x+1=\dfrac{5}{6}\)
b \(\left(2x-3\right).\left(x-5\right)=0\)
c \(\dfrac{1}{2}-|x+1|=0,25\)
\(a,\dfrac{-3}{4}x+1=\dfrac{5}{6}\\ \Rightarrow\dfrac{-3}{4}x=\dfrac{-1}{6}\\ \Rightarrow x=\dfrac{2}{9}\\ b,\left(2x-3\right)\left(x-5\right)=0\\ \Rightarrow\left[{}\begin{matrix}2x-3=0\\x-5=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=5\end{matrix}\right.\\ c,\dfrac{1}{2}-\left|x+1\right|=0,25\\ \Rightarrow\left|x+1\right|=0,25\\ \Rightarrow\left[{}\begin{matrix}x+1=0,25\\x+1=-0,25\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=-0,75\\x=-1,25\end{matrix}\right.\)
Đúng 2
Bình luận (0)
a: =>-3/4x=-1/6
hay x=2/9
b: =>2x-3=0 hoặc x-5=0
hay x=3/2 hoặc x=5
c: =>|x+1|=1/4
\(\Leftrightarrow x+1\in\left\{\dfrac{1}{4};-\dfrac{1}{4}\right\}\)
hay \(x\in\left\{-\dfrac{3}{4};-\dfrac{5}{4}\right\}\)
Đúng 0
Bình luận (0)
b, \(=>2x-3=0\) hay \(x-5=0\)
\(2x=0+3\) \(x=0+5\)
\(2x=3\) \(x=5\)
\(x=\dfrac{3}{2}\)
Vậy x ϵ { \(\dfrac{3}{2}\);5}
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Tìm \(x\) :
\(a.1\dfrac{2}{4}x-1\dfrac{2}{3}=2\dfrac{3}{5}\) \(b.0,5x-\dfrac{2}{3}x=\dfrac{7}{12}\) \(c.5,5x=\dfrac{1}{2}\)
a: =>3/2x=64/15
=>x=128/45
b: =>-1/6x=7/12
=>x=-7/2
c: =>11/2*x=1/2
=>x=1/2:11/2=1/11
Đúng 0
Bình luận (0)