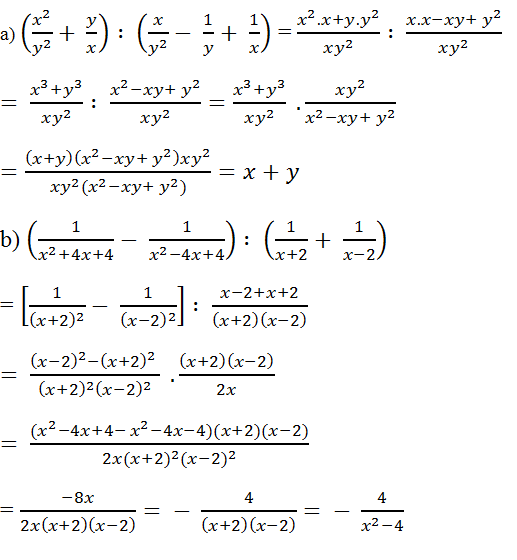b: \(=\dfrac{x^3+6x^2-25}{x\left(x+5\right)\left(x-2\right)}+\dfrac{x+5}{x\left(x-2\right)}\)

\(=\dfrac{x^3+6x^2-25+x^2+10x+25}{x\left(x+5\right)\left(x-2\right)}=\dfrac{x^3+7x^2+10x}{x\left(x+5\right)\left(x-2\right)}=\dfrac{x+2}{x-2}\)

Ty nhi ha

5-/2x+6/-/7-y/

bài 1:

   (-2x^5 y)^8:(-2x^5 y)^6

=(-2x^5 y)^8-6

=(-2x^5y)^2

=4x^25 y^2

MTC = (x - y)(x² + xy + y²)

\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)

\(=\dfrac{x^2+xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}-\dfrac{3xy}{\left(x-y\right)\left(x^2+xy+y^2\right)}+\dfrac{\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{x^2+xy+y^2-3xy+x^2-2xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2x^2-4xy+2y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2\left(x^2-2xy+y^2\right)}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)

1/x-y-3xy/x^3-y^3+x-y/x^2+xy+y^2

=1/x-y+-3xy/(x-y)(x^2+xy+y^2)+x-y/x^2+xy+y^2

=x^2+xy+y^2/(x-y)(x^2+xy+y^2)+-3xy/(x-y)(x^2+xy+y^2)+x^2-2xy+y^2/(x-y)(x^2+xy+y^2)

=x^2+xy+y^2-3xy+x^2-2xy-y^2/(x-y)(x^2+xy+y^2)

=2x^2-5xy/(x-y)(x^2+xy+y^2)

MTC = (x - y)(x² + xy + y²)

\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)

\(=\dfrac{x^2+xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}-\dfrac{3xy}{\left(x-y\right)\left(x^2+xy+y^2\right)}+\dfrac{\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{x^2+xy+y^2-3xy+x^2-2xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2x^2-4xy+2y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2\left(x^2-2xy+y^2\right)}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)

\(=\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)

\(\dfrac{3}{2x^2+y}+\dfrac{5}{xy^2+}+\dfrac{x}{y^3}\)

=\(\dfrac{3xy^5}{xy^2.y^3\left(2x^2+y\right)+}+\dfrac{10y^3x^2+5y^4}{xy^2.y^3\left(2x^2+y\right)}+\dfrac{2x^4y^2+x^2y^3}{xy^2.y^3\left(2x^2+y\right)}\)

=\(\dfrac{3xy^5+10y^3x^2+5y^4+2x^4y^2+x^2y^3}{xy^5\left(2x^2+y\right)}\)

=\(\dfrac{3xy^5+11y^3x^2+5y^4+2x^4y^2}{xy^5\left(2x^2+y\right)}\)

   ủa đáp án cứ sao sao:<

\(\dfrac{xy}{x-y}-\dfrac{2x^2}{y-2x}\)

\(=\dfrac{xy}{x-y}+\dfrac{2x^2}{2x-y}\)

\(=\dfrac{xy\left(2x-y\right)+2x^2\left(x-y\right)}{\left(x-y\right)\left(2x-y\right)}\)

\(=\dfrac{2x^2y-xy^2+2x^3-2x^2y}{\left(x-y\right)\left(2x-y\right)}\)

\(=\dfrac{2x^3-xy^2}{\left(x-y\right)\left(2x-y\right)}=\dfrac{x\left(2x^2-y^2\right)}{\left(x-y\right)\left(2x-y\right)}\)