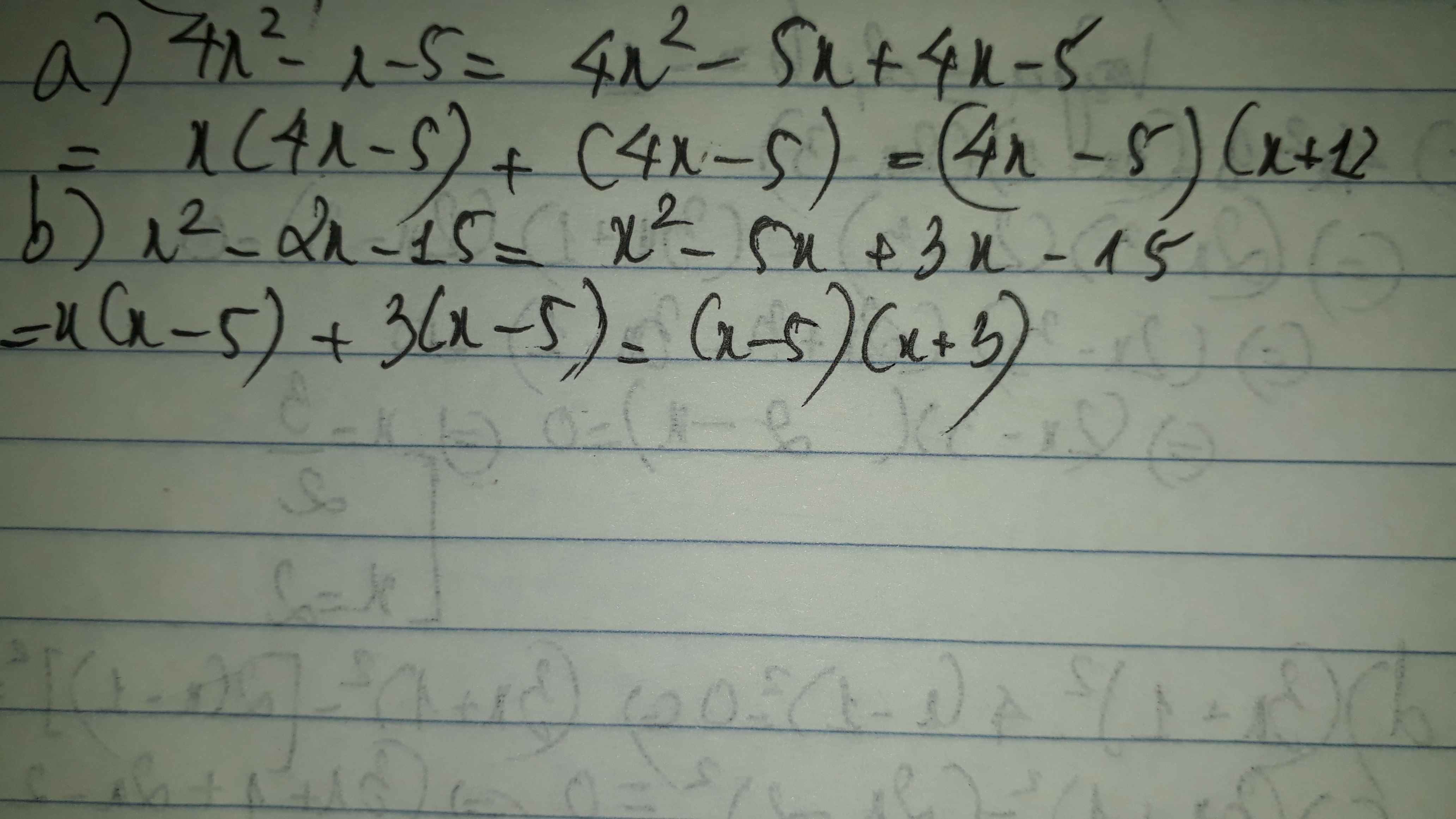a)

\(x^3-7x-6=x^3+1-7x-7\)

= \(\left(x+1\right)\left(x^2-x+1\right)-7\left(x+1\right)\)

= \(\left(x+1\right)\left(x^2-x+1-7\right)\)

= \(\left(x+1\right)\left(x^2-x-6\right)\)

b)

\(x^3-5x^2-14x=x^3+2x^2-7x^2-14x\)

= \(x^2\left(x+2\right)-7x\left(x+2\right)\)

= \(\left(x^2-7x\right)\left(x+2\right)\)

= \(x\left(x-7\right)\left(x+2\right)\)

a,x^3 -x-6x-6 = x(x^2 -1)-6(x+1)= x(x-1)(x+1)-6(x+1)=(x+1)(X^2-x-6)=(x+1)(x^2+2x-3x-6)=(x+1)(x(x+2)-3(x+2))=(x+1)(x+2)(x-3)

b,x(x^2-5x-14)=x(x^2+2x-7x-14)=x(x(x+2)-7(x+2))=x(x+2)(x-7)

a) \(=x^2-2x-4x+8\)

\(=x\left(x-2\right)-4\left(x-2\right)\)

\(=\left(x-2\right)\left(x-4\right)\)

c) \(=x^3-x-6x-6\)

\(=x\left(x^2-1\right)-6\left(x+1\right)\)

\(=x\left(x+1\right)\left(x-1\right)-6\left(x+1\right)\)

\(=x\left(x+1\right)\left(x-1-6\right)\)

\(=x\left(x+1\right)\left(x-7\right)\)

cái này chính là BĐT \(a^2+b^2+c^2\ge ab+bc+ca\Leftrightarrow2\left(a^2+b^2+c^2\right)\ge2\left(ab+bc+ca\right)\)

<=>\(\left(a-b\right)^2+\left(b-c\right)^2+\left(c-a\right)^2\ge0\)

với c=1 tì ta luôn có ĐPCM

a⁴ + a² +1

= (a²)² + 2a² +1 -a²

= (a² +1)² -a²

= (a² +1 -a)(a² +1 +a)

a, 3x^2 + 13x + 10  

= 3x^2 + 3x + 10x + 10 

= 3x(x + 1) + 10(x + 1)

= (3x + 10)(x + 1)

b, x^2 - 10x + 21

= x^2 - 3x - 7x + 21

= x(x - 3) - 7(x - 3)

= (x - 7)(x - 3)

c, 6x^2 - 5x + 1

= 6x^2 - 3x - 2x + 1

= 3x(2x - 1) - (2x - 1)

= (3x - 1)(2x - 1)

Bạn đăng 1 lần nhiều bài như vậy làm người khác nản lắm đấy =) đơn giản bài rất dài mà mik cx ko chắc là bản thân mik có đc k hay ko nên phải nản vậy thôi :)

1a)\(3x^2+13x+10=3x^2+3x+10x+10\)

\(3x\left(x+1\right)+10\left(x+1\right)=\left(3x+10\right)\left(x+1\right)\)

b)\(x^2-10x+21=x^2-3x-7x+21\)

\(=x\left(x-3\right)-7\left(x-3\right)=\left(x-7\right)\left(x-3\right)\)

c)\(6x^2-5x+1=6x^2-3x-2x+1\)

\(=3x\left(2x-1\right)-\left(2x-1\right)=\left(3x-1\right)\left(2x-1\right)\)

1,

\(a,3x^2+13x+10=3x^2+3x+10x+10\)

\(=3x\left(x+1\right)+10\left(x+1\right)\)

\(=\left(x+1\right)\left(3x+10\right)\)

\(b,x^2-10x+21=x^2-7x-3x+21\)

\(=x\left(x-7\right)-3\left(x-7\right)\)

\(=\left(x-7\right)\left(x-3\right)\)

\(c,6x^2-5x+1=6x^2-2x-3x+1\)

\(=2x\left(3x-1\right)-\left(3x-1\right)\)

\(=\left(3x-1\right)\left(2x-1\right)\)

2,

\(a,x^8+4=\left(x^4\right)^2+4x^4+2^2-4x^4\)

\(=\left(x^4+2\right)^2-4x^4\)

\(=\left(x^4+2-2x^2\right)\left(x^4+2+2x^2\right)\)

\(b,x^4+18^2=\left(x^2\right)^2+36x^2+18^2-36x^2\)

\(=\left(x^2+18\right)-36x^2\)

\(=\left(x^2+18-6x\right)\left(x^2+18+6x\right)\)

\(c,x^4+3x^2+4=x^4+4x^2+4-x^2\)

\(=\left(x^2+2\right)-x^2\)

\(=\left(x^2+2-x\right)\left(x^2+2+x\right)\)

Toán Học Team 

a) \(x^3+4x^2-21x\)

\(=x\left(x^2+4x-21\right)\)

\(=x\left(x^2-3x+7x-21\right)\)

\(=x\left[x\left(x-3\right)+7\left(x-3\right)\right]\)

\(=x\left(x-3\right)\left(x+7\right)\)

b) \(5x^3+6x^2+x\)

\(=x\left(5x^2+6x+1\right)\)

\(=x\left(5x^2+5x+x+1\right)\)

\(=x\left[5x\left(x+1\right)+\left(x+1\right)\right]\)

\(=x\left(x+1\right)\left(5x+1\right)\)

c) \(x^3-7x+6\)

\(=x^3+2x^2-3x-2x^2-4x+6\)

\(=x\left(x^2+2x-3\right)-2\left(x^2+2x-3\right)\)

\(=\left(x-2\right)\left(x^2+2x-3\right)\)

\(=\left(x-2\right)\left(x-1\right)\left(x+3\right)\)

d) \(3x^3+2x-5\)

\(=3x^3+3x^2+5x-3x^2-3x-5\)

\(=x\left(3x^2+3x+5\right)-\left(3x^2+3x+5\right)\)

\(=\left(x-1\right)\left(3x^2+3x+5\right)\)

a: \(4x^2-x-5=\left(4x-5\right)\left(x+1\right)\)

b: \(x^2-2x-15=\left(x-5\right)\left(x+3\right)\)

1) \(^{x^2\left(a+b-c\right)+x\left(a+b-c\right)}\)

\(=\left(x^2+x\right)\left(a+b-c\right)\)

\(=x\left(x+1\right)\left(a+b-c\right)\)

2) \(m\left(a^2-b^2\right)+n\left(a^2-b^2\right)=\left(m+n\right)\left(a^2-b^2\right)\)

3) \(12x^2-13x+3=12x^2-9x-4x+3\)

\(=3x\left(4x+3\right)-\left(4x+3\right)=\left(3x-1\right)\left(4x+3\right)\)

4) \(15x^2-31x+2=15x^2-30x-x+2\)

\(=15x\left(x-2\right)-\left(x-2\right)\)\(=\left(15x-1\right)\left(x-2\right)\)