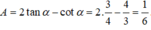cho sin α =\(\frac{-5}{13}\) và \(\frac{3\pi}{2}\) < α < π . Tìm sin α , tan α , cot α
Những câu hỏi liên quan
bài 1: a)biết sin α=√3/2.tính cos α,tan α,cot α
b)cho tan α=2.tính sin α,cos α,cot α
c)biết sin α=5/13.tính cos,tan,cot α
bài 2
biết sin α x cos α=12/25.tính sin,cos α
1:
a: sin a=căn 3/2
\(cosa=\sqrt{1-sin^2a}=\sqrt{1-\dfrac{3}{4}}=\sqrt{\dfrac{1}{4}}=\dfrac{1}{2}\)
\(tana=\dfrac{\sqrt{3}}{2}:\dfrac{1}{2}=\sqrt{3}\)
cot a=1/tan a=1/căn 3
b: \(tana=2\)
=>cot a=1/tan a=1/2
\(1+tan^2a=\dfrac{1}{cos^2a}\)
=>\(\dfrac{1}{cos^2a}=5\)
=>cos^2a=1/5
=>cosa=1/căn 5
\(sina=\sqrt{1-cos^2a}=\sqrt{\dfrac{4}{5}}=\dfrac{2}{\sqrt{5}}\)
c: \(cosa=\sqrt{1-\left(\dfrac{5}{13}\right)^2}=\dfrac{12}{13}\)
tan a=5/13:12/13=5/12
cot a=1:5/12=12/5
Đúng 0
Bình luận (0)
Cho α ∈ (0;\(\dfrac{\Pi}{2}\)) và tan α = 3. Khi đó sin(α +π) bằng
do a ∈ \(\left(0;\dfrac{\pi}{2}\right)\)⇒ \(\left\{{}\begin{matrix}sinx>0\\cosx>0\end{matrix}\right.\)
Mà tanx = 3 ⇒ \(\dfrac{sinx}{cosx}=3\Leftrightarrow\dfrac{sin^2x}{cos^2x}=9\Rightarrow10sin^2x=9\)
⇒ sinx = \(\dfrac{3}{\sqrt{10}}\)
⇒ sin (x + π) = -sinx = -\(\dfrac{3}{\sqrt{10}}\)
Đúng 0
Bình luận (0)
cho \(\dfrac{\pi}{2}\)<α<\(\pi\). tìm khẳng định đúng?
A. sin α<0 B. tan α>0 C. cot α>0 D. cos α<0
giải chi tiết nha
D
Vì 0 < α < π/2 nên sin α > 0, cos α > 0, tan α > 0, cot α > 0.
Đúng 4
Bình luận (0)
`\pi/2 < \alpha < \pi=>\alpha` nằm ở góc phần tư thứ `2`
`=>{(sin \alpha > 0;cos \alpha < 0),(tan \alpha < 0; cot \alpha < 0):}`
`->\bb D`
Đúng 2
Bình luận (0)
trang 40 SGK Toán 11 tập 1 - Kết nối tri thức
15 tháng 7 2023 lúc 12:46
Cho góc α
thỏa mãn `π\2`<α<π,cosα=−\(\dfrac{1}{\sqrt{3}}\). Tính giá trị của các biểu thức sau:
a) sin(α+\(\dfrac{\text{π}}{6}\))
b) cos(α+$\frac{\text{π}}{6}$)
c) sin(α−$\frac{\text{π}}{3}$)
d) cos(α−$\frac{\text{π}}{6}$)
a: pi/2<a<pi
=>sin a>0
\(sina=\sqrt{1-\left(-\dfrac{1}{\sqrt{3}}\right)^2}=\dfrac{\sqrt{2}}{\sqrt{3}}\)
\(sin\left(a+\dfrac{pi}{6}\right)=sina\cdot cos\left(\dfrac{pi}{6}\right)+sin\left(\dfrac{pi}{6}\right)\cdot cosa\)
\(=\dfrac{\sqrt{3}}{2}\cdot\dfrac{\sqrt{2}}{\sqrt{3}}+\dfrac{1}{2}\cdot-\dfrac{1}{\sqrt{3}}=\dfrac{\sqrt{6}-2}{2\sqrt{3}}\)
b: \(cos\left(a+\dfrac{pi}{6}\right)=cosa\cdot cos\left(\dfrac{pi}{6}\right)-sina\cdot sin\left(\dfrac{pi}{6}\right)\)
\(=\dfrac{-1}{\sqrt{3}}\cdot\dfrac{\sqrt{3}}{2}-\dfrac{\sqrt{2}}{\sqrt{3}}\cdot\dfrac{1}{2}=\dfrac{-\sqrt{3}-\sqrt{2}}{2\sqrt{3}}\)
c: \(sin\left(a-\dfrac{pi}{3}\right)\)
\(=sina\cdot cos\left(\dfrac{pi}{3}\right)-cosa\cdot sin\left(\dfrac{pi}{3}\right)\)
\(=\dfrac{\sqrt{2}}{\sqrt{3}}\cdot\dfrac{1}{2}+\dfrac{1}{\sqrt{3}}\cdot\dfrac{\sqrt{3}}{2}=\dfrac{\sqrt{2}+\sqrt{3}}{2\sqrt{3}}\)
d: \(cos\left(a-\dfrac{pi}{6}\right)\)
\(=cosa\cdot cos\left(\dfrac{pi}{6}\right)+sina\cdot sin\left(\dfrac{pi}{6}\right)\)
\(=\dfrac{-1}{\sqrt{3}}\cdot\dfrac{\sqrt{3}}{2}+\dfrac{\sqrt{2}}{\sqrt{3}}\cdot\dfrac{1}{2}=\dfrac{-\sqrt{3}+\sqrt{2}}{2\sqrt{3}}\)
Đúng 0
Bình luận (0)
cho góc α thoả mãn\(\dfrac{3\pi}{2}< \alpha< 2\pi\). Mệnh đề nào sau đây đúng?
A. \(tan\)α > 0 B. \(cot\)α > 0 C. \(sin\)α > 0 D. \(cos\)α > 0
Rút gọn biểu thức
\(E = cot(5π+α).cos(α-\dfrac{3π}{2})+cos(α-2π)-2.cos(\dfrac{π}{2}+α)\)\(D = sin(π+α)-cos(\dfrac{π}{2}-α)+cot(4π-α)+tan(\dfrac{5π}{2}-α)\)
28 tháng 7 2021 lúc 17:24
Cho sin α + cos α=√2
a, Tính cos α, sin α, tan α, cot α
b, Tính F = \(sin^5\alpha+cos^5\alpha\)
Cho góc
α
thỏa mãn
π
α
3
π
2
và sin
α
-2cos
α
1.Tính A 2tan
α
-cot
α
A. 6 B.
1
6
C. 2 D.
1
2
Đọc tiếp
Cho góc α thỏa mãn π < α < 3 π 2 và sin α -2cos α =1.Tính A= 2tan α -cot α
A. 6
B. 1 6
C. 2
D. 1 2
Cho tan α + cot α = 3. Tính sin α.cos α và tan2 α + cot2 α
Cho tan α + cot α = 3. Tính sin α.cos α và tan2 α + cot2 α
Ta có: \(tana+cota=3\Rightarrow\dfrac{sina}{cosa}+\dfrac{cosa}{sina}=3\)
\(\Rightarrow\dfrac{sin^2a+cos^2a}{sina\cdot cosa}=3\Rightarrow sina\cdot cosa=\dfrac{1}{3}\)
Ta có: \(\left(tana+cota\right)^2=9\)\(\Rightarrow tan^2a+cot^2a=9-2tana\cdot cota=9-2=7\)
Đúng 1
Bình luận (0)