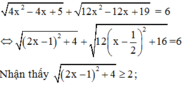a.

\(x^3-7x+6=0\)

\(\Leftrightarrow x^3-3x^2+2x+3x^2-9x+6=0\)

\(\Leftrightarrow x\left(x^2-3x+2\right)+3\left(x^2-3x+2\right)=0\)

\(\Leftrightarrow\left(x^2-3x+2\right)\left(x+3\right)=0\)

\(\Leftrightarrow\left(x^2-x-2x+2\right)\left(x+3\right)=0\)

\(\Leftrightarrow\left[x\left(x-1\right)-2\left(x-1\right)\right]\left(x+3\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x-2\right)\left(x+3\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x=1\\x=2\\x=-3\end{matrix}\right.\)

f.

\(x^4-4x^3+12x-9=0\)

\(\Leftrightarrow x^4-4x^3+3x^2-3x^2+12x-9=0\)

\(\Leftrightarrow x^2\left(x^2-4x+3\right)-3\left(x^2-4x+3\right)=0\)

\(\Leftrightarrow\left(x^2-4x+3\right)\left(x^2-3\right)=0\)

\(\Leftrightarrow\left(x^2-x-3x+3\right)\left(x^2-3\right)=0\)

\(\Leftrightarrow\left[x\left(x-1\right)-3\left(x-1\right)\right]\left(x^2-3\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x-3\right)\left(x^2-3\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x=1\\x=3\\x=\pm\sqrt{3}\end{matrix}\right.\)

g.

\(x^5-5x^3+4x=0\)

\(\Leftrightarrow x\left(x^4-5x^2+4\right)=0\)

\(\Leftrightarrow x\left(x^4-x^2-4x^2+4\right)=0\)

\(\Leftrightarrow x\left[x^2\left(x^2-1\right)-4\left(x^2-1\right)\right]=0\)

\(\Leftrightarrow x\left(x^2-1\right)\left(x^2-4\right)=0\)

\(\Leftrightarrow x\left(x-1\right)\left(x+1\right)\left(x-2\right)\left(x+2\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x=0\\x=\pm1\\x=\pm2\end{matrix}\right.\)

a, \(\Leftrightarrow\left(9x^2-4\right)\left(x+1\right)-\left(3x+2\right)\left(x-1\right)\left(x+1\right)=0\)

\(\Leftrightarrow\left(x+1\right)\left(\left(9x^2-4\right)-\left(\left(3x+2\right)\left(x-1\right)\right)\right)=0\)

\(\Leftrightarrow\left(x+1\right)\left(9x^2-4-\left(3x^2-x-2\right)\right)=0\)

\(\Leftrightarrow\left(x+1\right)\left(9x^2-4-3x^2+x+2\right)=0\)

\(\Leftrightarrow\left(x+1\right)\left(3x^2+x-2\right)=0\)

\(\Leftrightarrow\left(x+1\right)=0;3x^2+x-2=0\)

=> x=-1  

với \(3x^2+x-2=0\)

ta sử dụng công thức bậc 2 suy ra : \(x=\dfrac{2}{3};x=-1\)

Vậy  ghiệm của pt trên \(S\in\left\{-1;\dfrac{2}{3}\right\}\)

b: \(\Leftrightarrow x^2-2x+1-1+x^2=x+3-x^2-3x\)

\(\Leftrightarrow2x^2-2x=-x^2-2x+3\)

\(\Leftrightarrow3x^2=3\)

hay \(x\in\left\{1;-1\right\}\)

c: \(\Leftrightarrow\left(x-1\right)\left(x+1\right)\left(x+2\right)\left(x-3\right)-\left(x-1\right)\left(x-2\right)\left(x+2\right)\left(x+5\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left[\left(x+1\right)\left(x-3\right)-\left(x-2\right)\left(x+5\right)\right]=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left(x^2-2x-3-x^2-3x+10\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left(-5x+7\right)=0\)

hay \(x\in\left\{1;-2;\dfrac{7}{5}\right\}\)

Cả ba phương trình trên đều là phương trình trùng phương.

a)  3 x 4   –   12 x 2   +   9   =   0   ( 1 )

Đặt x 2   =   t ,  t ≥ 0.

(1) trở thành:  3 t 2   –   12 t   +   9   =   0   ( 2 )

Giải (2):

Có a = 3; b = -12; c = 9

⇒ a + b + c = 0

⇒ (2) có hai nghiệm  t 1   =   1   v à   t 2   =   3 .

Cả hai nghiệm đều thỏa mãn điều kiện.

+ t = 3 ⇒ x 2 = 3 ⇒ x = ± 3 + t = 1 ⇒ x 2 = 1 ⇒ x = ± 1

Vậy phương trình có tập nghiệm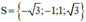 

b)  2 x 4   +   3 x 2   –   2   =   0   ( 1 )

Đặt x 2   =   t , t ≥ 0.

(1) trở thành:    2 t 2   +   3 t   –   2   =   0   ( 2 )

Giải (2) :

Có a = 2 ; b = 3 ; c = -2

⇒   Δ   =   3 2   –   4 . 2 . ( - 2 )   =   25   >   0

⇒ (2) có hai nghiệm

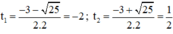
t 1   =   - 2   <   0  nên loại.

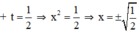
Vậy phương trình có tập nghiệm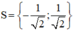 

c)  x 4   +   5 x 2   +   1   =   0   ( 1 )

Đặt  x 2   =   t ,   t   >   0 .

(1) trở thành:  t 2   +   5 t   +   1   =   0   ( 2 )

Giải (2):

Có a = 1; b = 5; c = 1

⇒   Δ   =   5 2   –   4 . 1 . 1   =   21   >   0

⇒ Phương trình có hai nghiệm:

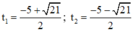
Cả hai nghiệm đều < 0 nên không thỏa mãn điều kiện.

Vậy phương trình (1) vô nghiệm.

Đáp án A

Bài 1: \(x^4-12x^2+27=0\)

\(\Leftrightarrow\left(x^2-3\right)\left(x^2-9\right)=0\)

hay \(x\in\left\{\sqrt{3};-\sqrt{3};3;-3\right\}\)