2 x - y = 5 x + y + 2 x + 2 y - 5 = 0

Ta đưa về giải hai hệ phương trình:

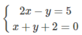
hoặc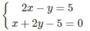 

Giải hệ:

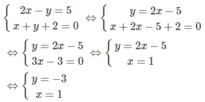
Giải hệ:

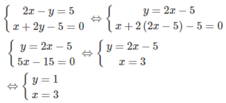
Vậy hệ phương trình đã cho có hai nghiệm

( x 1 ; y 1 ) = (1; -3) và ( x 2 ; y 2 ) = (3; 1)

\(a,\left\{{}\begin{matrix}x+y=3\\2x-3y=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}2x+2y=6\\2x-3y=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}5y=5\\2x-3y=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}y=1\\2x-3.1=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)

Vậy \(\left(x;y\right)=\left(2;1\right)\)

b, \(x^2-7x+10=0\\ \Leftrightarrow x^2-5x-2x+10=0\\ \Leftrightarrow x\left(x-5\right)-2\left(x-5\right)=0\\ \Leftrightarrow\left(x-2\right)\left(x-5\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-2=0\\x-5=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=2\\x=5\end{matrix}\right.\)

\(a,\)\(\left\{{}\begin{matrix}x+y=3\\2x-3y=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}3x+3y=9\\2x-3y=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}5x=10\\2x-3y=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=2\\2.2-3y=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)

Vậy hệ pt có nghiệm duy nhất \(\left(x;y\right)=\left(2;1\right)\)

\(b,x^2-7x+10=0\)

\(\Delta=b^2-4ac=\left(-7\right)^2-4.10=9>0\)

\(\Rightarrow\) Pt có 2 nghiệm \(x_1,x_2\)

Ta có :

\(\left\{{}\begin{matrix}x_1=\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{7+3}{2}=5\\x_2=\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{7-3}{2}=2\end{matrix}\right.\)

Vậy \(S=\left\{5;2\right\}\)

1: 2x+y=m+1 và x-2y=2

Khi m=-2 thì (1) sẽ là 2x+y=-1 và x-2y=2

=>x=0 và y=-1

2: 2x+y=m+1 và x-2y=2

=>2x+y=m+1 và 2x-4y=4

=>5y=m-3 và x-2y=2

=>y=(m-3)/5 và x=2+2y=2+2/5(m-3)=2+2/5m-6/5=2/5m+4/5

x+y=2

=>2/5m+4/5+1/5m-3/5=2

=>3/5m=2-1/5=9/5

=>m=3

a, ĐKXĐ : \(\left[{}\begin{matrix}x\le-3\\x\ge0\end{matrix}\right.\)

TH1 : \(x\le-3\) ( LĐ )

TH2 : \(x\ge0\)

BPT \(\Leftrightarrow x^2+2x+x^2+3x+2\sqrt{\left(x^2+2x\right)\left(x^2+3x\right)}\ge4x^2\)

\(\Leftrightarrow\sqrt{\left(x^2+2x\right)\left(x^2+3x\right)}\ge x^2-\dfrac{5}{2}x\)

\(\Leftrightarrow2\sqrt{\left(x+2\right)\left(x+3\right)}\ge2x-5\)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x< \dfrac{5}{2}\\x\ge-2\end{matrix}\right.\\\left\{{}\begin{matrix}x\ge\dfrac{5}{2}\\4x^2+20x+24\ge4x^2-20x+25\end{matrix}\right.\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}0\le x< \dfrac{5}{2}\\x\ge\dfrac{5}{2}\end{matrix}\right.\)

\(\Leftrightarrow x\ge0\)

Vậy \(S=R/\left(-3;0\right)\)

a, Thay \(m=-1\) vào

\(=>\left\{{}\begin{matrix}-x+y=1\\2x-y=-1\end{matrix}\right.\\ =>\left\{{}\begin{matrix}x=0\\y=1\end{matrix}\right.\)

b, Để hệ pt có nghiệm duy nhất :

\(\dfrac{m}{2}\ne\dfrac{1}{-1}\\ =>\dfrac{m}{2}\ne-1\\ =>m\ne-2\)

1) \(\left\{{}\begin{matrix}2x+y=10\\5x-3y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}10x+5y=50\\10x-6y=6\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}11y=44\\2x+y=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=4\\x=3\end{matrix}\right.\)

Vậy hpt có nghiệm (x;y) = (3;4)

2)

a) 3x² - 2x - 1 = 0

\(\Leftrightarrow3x^2-3x+x-1=0\)

\(\Leftrightarrow3x\left(x-1\right)+\left(x-1\right)=0\)

\(\Leftrightarrow\left(3x+1\right)\left(x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{3}\\x=1\end{matrix}\right.\)

Vậy pt có nghiệm x = 1 hoặc x = 3

b) Đặt x² = t (t \(\ge\) 0)

Pt trở thành: t² - 20t + 4 = 0

\(\Delta\) = (-20)² - 4.1.4 = 400 - 16 = 384

=> pt có 2 nghiệm phân biệt t₁ = \(\dfrac{20+8\sqrt{6}}{2}=10+4\sqrt{6}\)

t₂ = \(\dfrac{20-8\sqrt{6}}{2}=10-4\sqrt{6}\)

=> x₁ = \(\sqrt{10+4\sqrt{6}}=\sqrt{\left(2+\sqrt{6}\right)^2}=2+\sqrt{6}\)

x₂ = \(2-\sqrt{6}\)

a) \(\hept{\begin{cases}2x+my=5\\3x-y=0\end{cases}\left(1\right)}\)

Thay m=0 vào (1) \(\Rightarrow\hept{\begin{cases}2x=5\\3x-y=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=\frac{5}{2}\\\frac{5}{2}\cdot3=y\end{cases}\Leftrightarrow}\hept{\begin{cases}x=\frac{5}{2}\\y=\frac{15}{2}\end{cases}}}\)

\(\hept{\begin{cases}8\left(2x+y\right)^2-10\left(2x+y\right)\left(2x-y\right)-3\left(2x-y\right)^2=0\\2x+y-\frac{2}{2x-y}=2\end{cases}}\)

\(\hept{\begin{cases}8\left(2x+y\right)^2-10\left(2x+y\right)\left(2x-y\right)-3\left(2x-y\right)^2=0\\\left(2x+y\right)\left(2x-y\right)-2=2\left(2x-y\right)\end{cases}}\)

\(\Leftrightarrow\hept{\begin{cases}8\left(2x+y\right)^2-10\left(2x+y\right)\left(2x-y\right)-3\left(2x-y\right)^2=0\\\left(2x+y\right)\left(2x-y\right)=2\left(2x-y\right)+2\end{cases}}\)

\(\Rightarrow8\left(2+\frac{2}{2x-y}\right)^2-20\left(2x-y\right)-20-3\left(2x-y\right)^2=0\)

Giải pt này vs ẩn là (2x-y) được nghiệm là 2

Rồi bạn lm nốt nhá