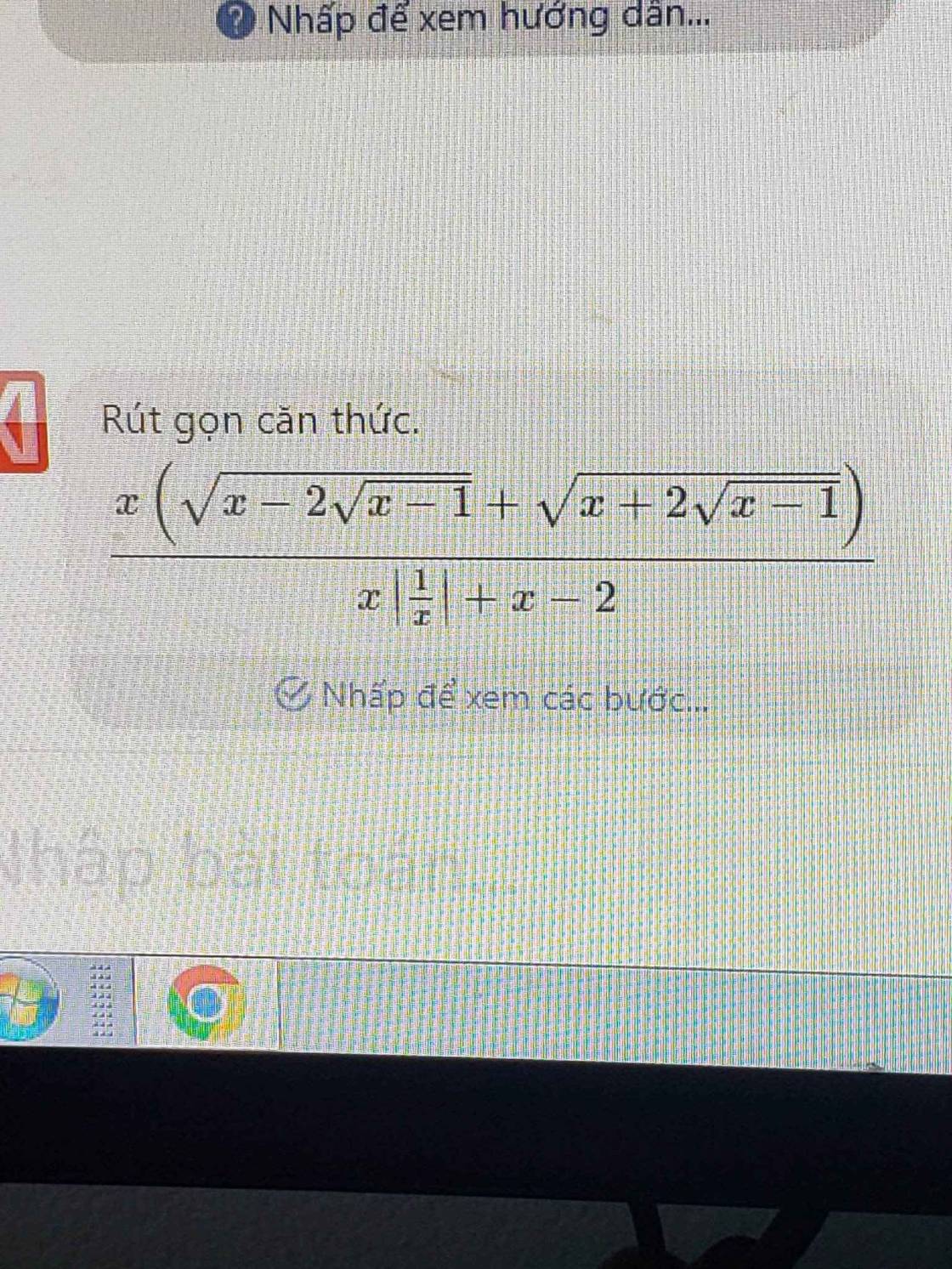
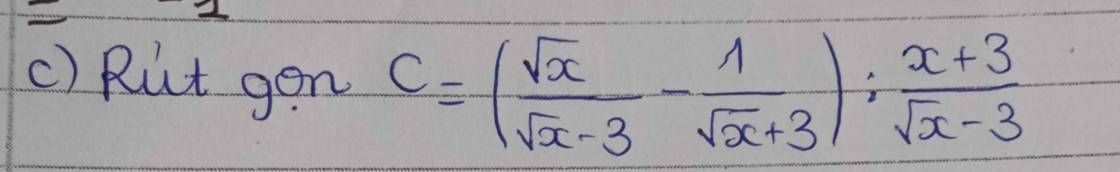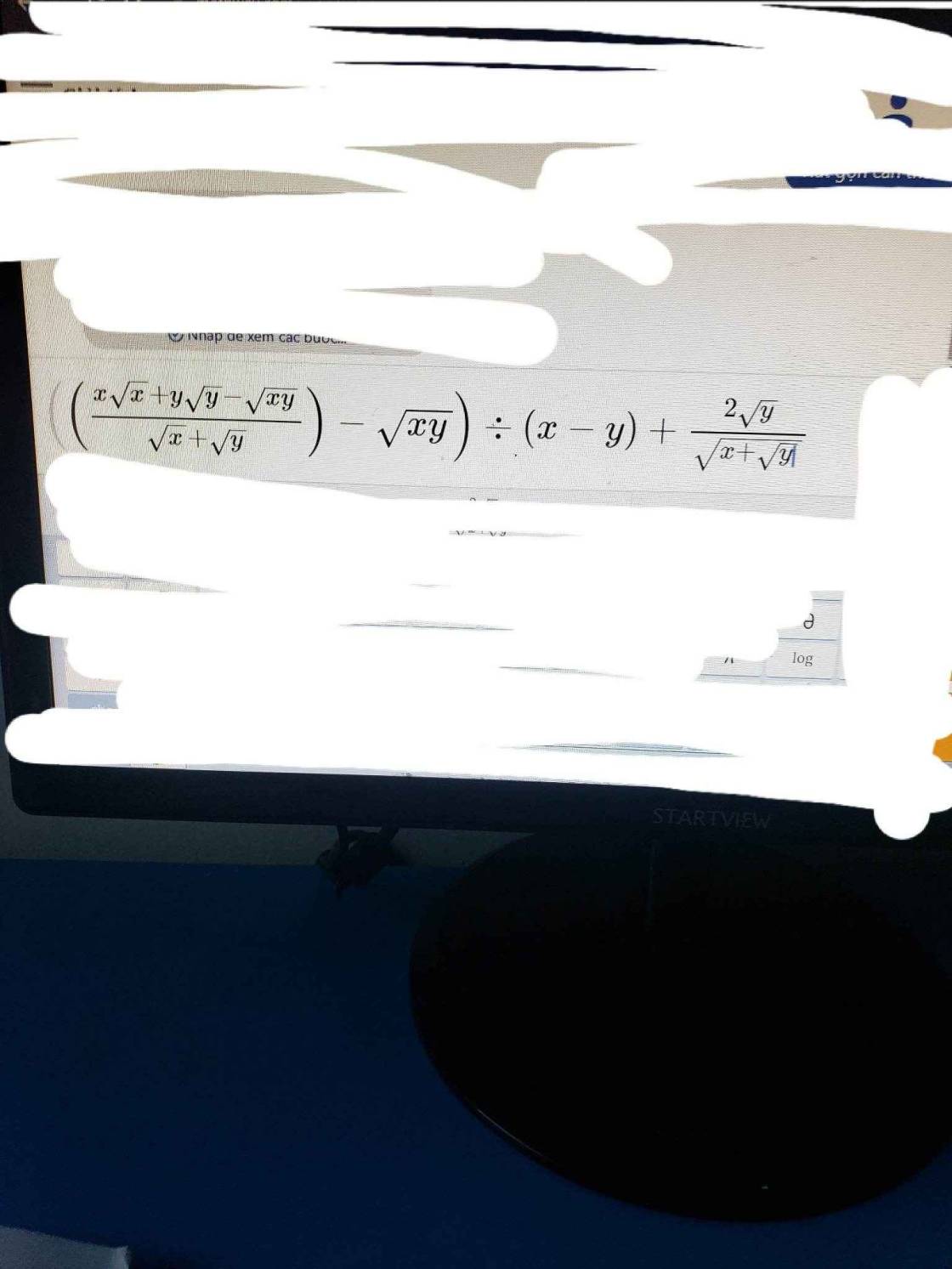Bài 2 :
a ) \(\sqrt{4x-8}+\sqrt{x-2}=4+\dfrac{1}{3}\sqrt{9x-18}\) ( ĐKXĐ : \(x\ge2\) )
\(\Leftrightarrow2\sqrt{x-2}+\sqrt{x-2}=4+\dfrac{1}{3}.3\sqrt{x-2}\)
\(\Leftrightarrow3\sqrt{x-2}-\sqrt{x-2}=4\)
\(\Leftrightarrow2\sqrt{x-2}=4\)
\(\Leftrightarrow\sqrt{x-2}=2\)
\(\Leftrightarrow x-2=4\)
\(\Leftrightarrow x=2\) ( thỏa mãn ĐKXĐ )
Vậy phương trình có nghiệm x = 2 .
Bài 2 :
b ) \(\sqrt{x^2-6x+9}-\dfrac{\sqrt{6}+\sqrt{3}}{\sqrt{2}+1}=0\)
\(\Leftrightarrow\sqrt{\left(x-3\right)^2}-\dfrac{\sqrt{3}\left(\sqrt{2}+1\right)}{\sqrt{2}+1}=0\)
\(\Leftrightarrow|x-3|-\sqrt{3}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3-\sqrt{3}=0\left(x\ge3\right)\\3-x-\sqrt{3}=0\left(x< 3\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3+\sqrt{3}\\x=3-\sqrt{3}\end{matrix}\right.\)
Vậy phương trình cón nghiệm \(x=3+\sqrt{3}\) hoặc \(x=3-\sqrt{3}\) .


 giúp vs ạ
giúp vs ạ