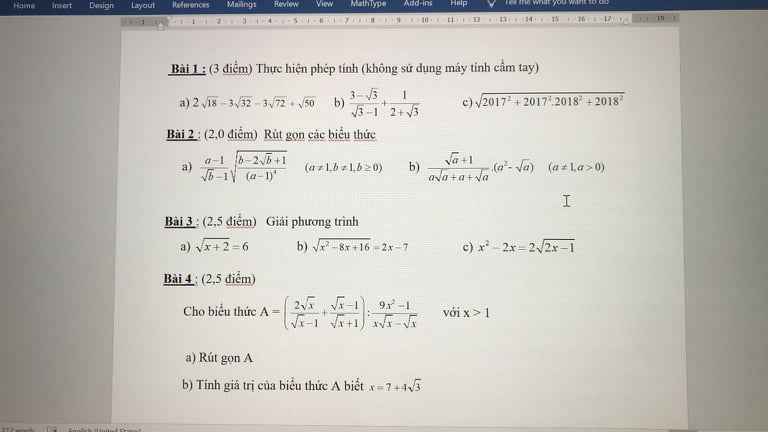
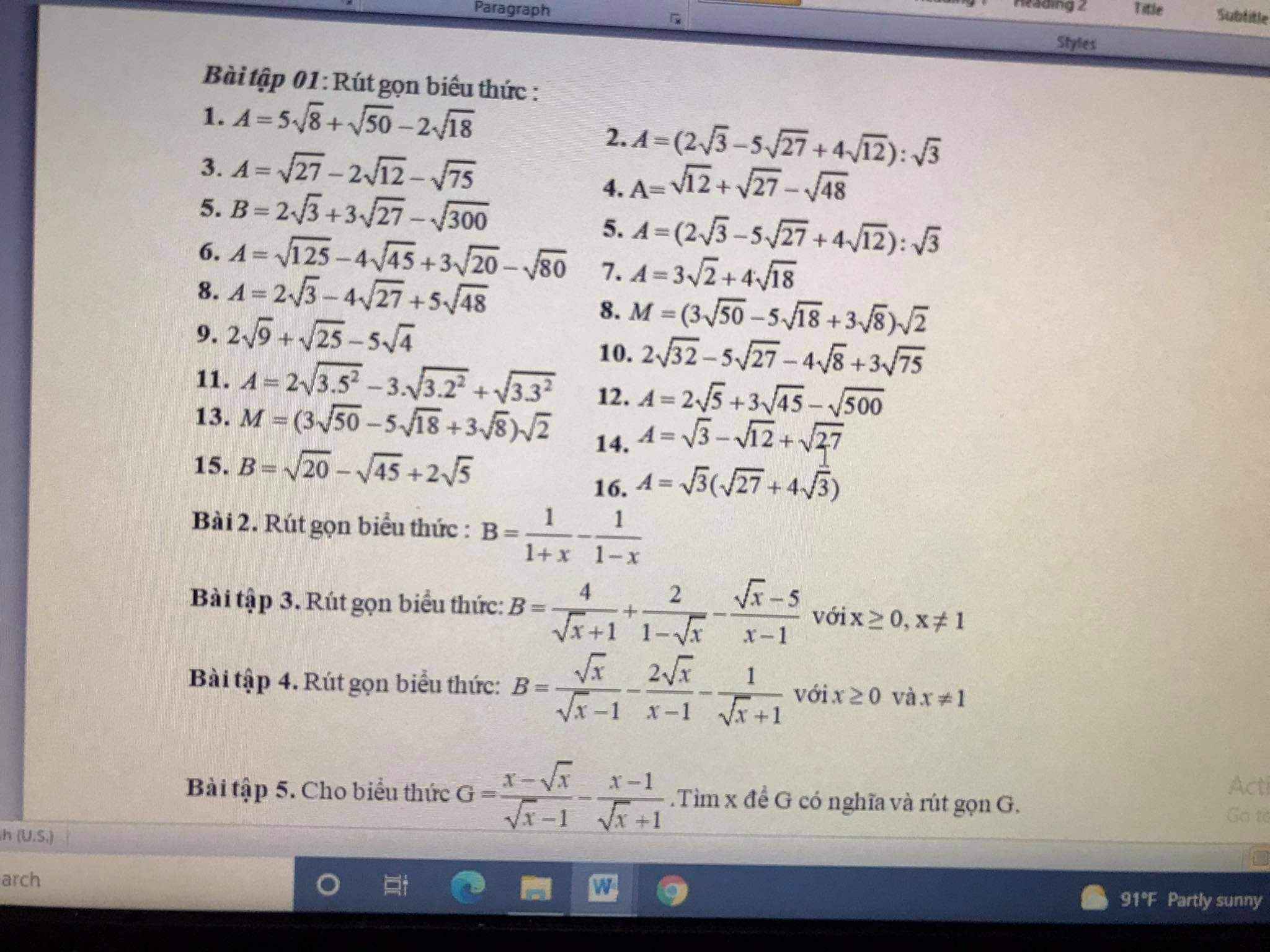
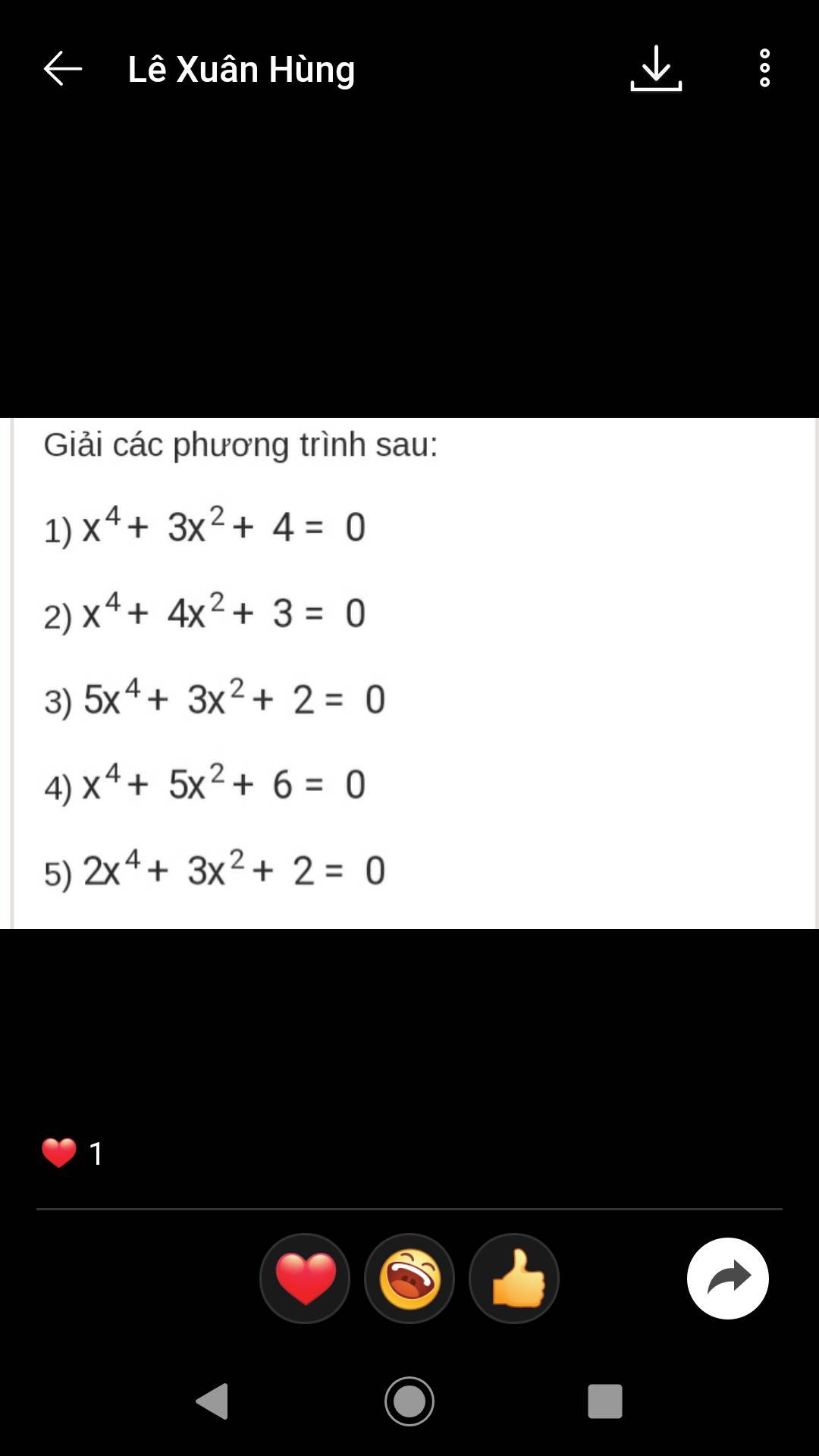
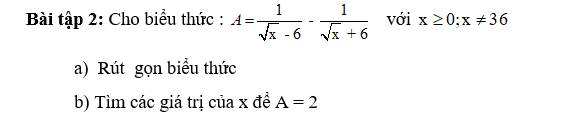Bài 4 :
b)\(x=7+4\sqrt{3}=\left(\sqrt{3}+2\right)^2\left(tmđk\right)\)
\(\rightarrow\sqrt{x}=\sqrt{3}+2\)
Từ đó \(\Rightarrow A=\dfrac{\sqrt{3}+2}{3\left(7+4\sqrt{3}\right)-1}\) =
Bài 4:
a)\(A=\left(\dfrac{2\sqrt{x}}{\sqrt{x}-1}+\dfrac{\sqrt{x}-1}{\sqrt{x}+1}\right):\dfrac{9x^2-1}{x\sqrt{x}-\sqrt{x}}\)
\(A=\dfrac{2\sqrt{x}\left(\sqrt{x}+1\right)+\left(\sqrt{x}-1\right)^2}{x-1}.\dfrac{\sqrt{x}\left(x-1\right)}{9x^2-1}\)
\(A=\dfrac{2x+2\sqrt{x}+x-2\sqrt{x}+1}{x-1}.\dfrac{\sqrt{x}\left(x-1\right)}{9x^2-1}\)
\(A=\dfrac{\left(3x+1\right).\sqrt{x}}{\left(3x+1\right)\left(3x-1\right)}\)
\(A=\dfrac{\sqrt{x}}{3x-1}\)
Mình đang cần gấp giúp mình với!
