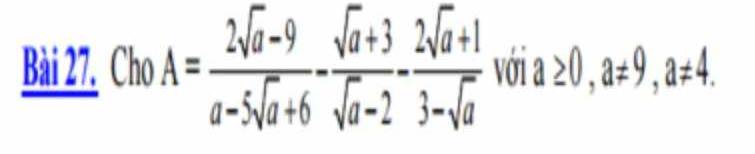a: \(A=\left(\dfrac{4}{x}-1\right):\left(1-\dfrac{x-3}{x^2+x+1}\right)\)

\(=\dfrac{4-x}{x}:\dfrac{x^2+x+1-x+3}{x^2+x+1}\)

\(=\dfrac{4-x}{x}\cdot\dfrac{x^2+x+1}{x^2+4}=\dfrac{\left(4-x\right)\left(x^2+x+1\right)}{x\left(x^2+4\right)}\)

b: x^4-7x^2-4x+20=0

=>(x-2)^2(x^2+4x+5)=0

=>x=2

Khi x=2 thì \(A=\dfrac{\left(4-2\right)\left(4+2+1\right)}{2\left(4+4\right)}=\dfrac{7}{8}\)

\(P=\dfrac{15\sqrt{x}-11-\left(3\sqrt{x}-2\right)\left(\sqrt{x}+3\right)-\left(2\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\)

\(=\dfrac{15\sqrt{x}-11-3x-9\sqrt{x}+2\sqrt{x}+6-2x+2\sqrt{x}-3\sqrt{x}+3}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\)

\(=\dfrac{-5x+7\sqrt{x}-2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}=\dfrac{-5x+5\sqrt{x}+2\sqrt{x}-2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\)

\(=\dfrac{\left(\sqrt{x}-1\right)\left(-5\sqrt{x}+2\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}=\dfrac{-5\sqrt{x}+2}{\sqrt{x}+3}\)

=\(\frac{30x^2y^3}{8x^2y^2}\)

Ta có :

\(\frac{6x^2y^2}{8xy^5}=\frac{3x}{4y^3}\)

\(\frac{x^2-xy}{5xy-5y^2}=\frac{x\left(x-y\right)}{5y\left(x-y\right)}=\frac{x}{5y}\)

Hok tốt !

\(\frac{6x^2y^2}{8xy^5}=\frac{6x^2y^2}{8xy^5}=\frac{6x}{8y^3}=\frac{3x}{4y^3}\)

\(\frac{x^2-xy}{5xy-5y^2}=\frac{x\left(x-y\right)}{5y\left(x-y\right)}=\frac{x}{5y}\)

\(ĐKXĐ:x\ne\pm1\)

\(A=\left(1+\frac{\sqrt{x}}{x+1}\right)\div\left(\frac{1}{\sqrt{x}-1}-\frac{2\sqrt{x}}{x\sqrt{x}+\sqrt{x}-x-1}\right)\)

\(\Leftrightarrow A=\frac{x+\sqrt{x}+1}{x+1}:\left(\frac{1}{\sqrt{x}-1}-\frac{2\sqrt{x}}{\left(x+1\right)\left(\sqrt{x}-1\right)}\right)\)

\(\Leftrightarrow A=\frac{x+\sqrt{x}+1}{x+1}:\frac{x+1-2\sqrt{x}}{\left(x+1\right)\left(\sqrt{x}-1\right)}\)

\(\Leftrightarrow A=\frac{x+\sqrt{x}+1}{x+1}:\frac{\left(\sqrt{x}-1\right)^2}{\left(x+1\right)\left(\sqrt{x}-1\right)}\)

\(\Leftrightarrow A=\frac{x+\sqrt{x}+1}{x+1}:\frac{\sqrt{x}-1}{x+1}\)

\(\Leftrightarrow A=\frac{x+\sqrt{x}+1}{\sqrt{x}-1}\)

b. Tìm giá trị của x sao cho A > 3 

Giúp mình ý tiếp với 

Bài làm:

đk: \(x>0;x\ne9;x\ne25\)

Ta có: \(\left(\frac{3+\sqrt{x}}{3-\sqrt{x}}-\frac{3-\sqrt{x}}{3+\sqrt{x}}+\frac{36}{9-x}\right)\div\left(\frac{\sqrt{x}-5}{3\sqrt{x}-x}\right)\)

\(=\left[\frac{\left(3+\sqrt{x}\right)^2-\left(3-\sqrt{x}\right)^2+36}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\right]\div\left[\frac{\sqrt{x}-5}{\left(3-\sqrt{x}\right)\sqrt{x}}\right]\)

\(=\frac{12\sqrt{x}+36}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\cdot\frac{\left(3-\sqrt{x}\right)\sqrt{x}}{\sqrt{x}-5}\)

\(=\frac{12\left(3+\sqrt{x}\right)}{3+\sqrt{x}}\cdot\frac{\sqrt{x}}{\sqrt{x}-5}=\frac{12\sqrt{x}}{\sqrt{x}-5}\)

Với a ≥ 0; a ≠ 1 ta có:

\(\dfrac{1-a\sqrt{a}}{1-\sqrt{a}}=\dfrac{(1-\sqrt{a})\left(1+\sqrt{a}+a\right)}{1-\sqrt{a}}\)\(=1+\sqrt{a}+a\)

\(a,C=\dfrac{2x^2-x-x-1+2-x^2}{x-1}\left(x\ne1\right)\\ C=\dfrac{x^2-2x+1}{x-1}=\dfrac{\left(x-1\right)^2}{x-1}=x-1\\ b,D=\dfrac{1+\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-1\right)^2}{\sqrt{a}+1}\left(a>0;a\ne1\right)\\ D=\dfrac{\sqrt{a}-1}{\sqrt{a}}\)

Có 

A = 5 + 5 ^ 2 + 5 ^ 3 + ... + 5 ^ 50

5 A = 5 ^ 2 + 5 ^ 3 + 5 ^ 4 + ... + 5 ^ 51

5 A - A = ( 5 ^ 2 + 5 ^ 3 + 5 ^ 4 + ... + 5 ^ 51 )

            -  ( 5 + 5 ^ 2 + 5 ^ 3 + ... + 5 ^ 50 )

4 A      = 5 ^ 51 - 5

A         = \(\frac{5^{51}-5}{4}\)

A=5^1+5^21+5^3+...+5^50

5^1A=5(5^1+5^2+5^3+..+5^50)

5A=5^2+5^3+..+5^50+5^51

5A-A=(5^2+5^3+..+5^50+5^51)-(5^1+5^2+5^3+..+5^50)

4A=5^51-5^1

A=(5^51-5^1):4