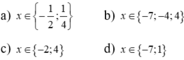lớp 8 có pt bậc 2 ak??

\(m,x^2+6x-16=0\)

\(\Leftrightarrow x^2-2x+8x-16=0\)

\(\Leftrightarrow x\left(x-2\right)+8\left(x-2\right)=0\)

\(\Leftrightarrow\left(x+8\right)\left(x-2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x+8=0\\x-2=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-8\\x=2\end{matrix}\right.\)

\(n,2x^2+5x-3=0\)

\(\Leftrightarrow2x^2-x+6x-3=0\)

\(\Leftrightarrow x\left(2x-1\right)+3\left(2x-1\right)=0\)

\(\Leftrightarrow\left(x+3\right)\left(2x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\2x-1=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=\dfrac{1}{2}\end{matrix}\right.\)

\(k,x\left(2x-7\right)-4x+14=0\)

\(\Leftrightarrow2x^2-4x-7x+14=0\)

\(\Leftrightarrow2x\left(x-2\right)-7\left(x-2\right)=0\)

\(\Leftrightarrow\left(2x-7\right)\left(x-2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}2x-7=0\\x-2=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=2\end{matrix}\right.\)

a) Thực hiện rút gọn VT = -2x – 64

Giải phương trình -2x – 64 = 0 thu được x = -32.

b) Thực hiện rút gọn VT = -62 x +12

Giải phương trình -62x + 12 = -50 thu được x = 1.

b) (x – 4). (x2 + 4x + 16) – x. (x2 - 6) = 2

⇔ x3 + 4x2 + 16x – 4x2 – 16x – 64 – (x3 - 6x ) – 2= 0

⇔ x3 + 4x2 + 16x – 4x2 – 16x – 64 – x3 + 6x – 2= 0

⇔ 6x – 66 =0

⇔ 6x = 66

⇔ x = 66 : 6

⇔ x = 11

Vậy x = 11

1)(x²-4x+16)(x+4)-x(x+1)(x+2)+3x²=0

\(\Rightarrow\)(x³+64)-x(x²+2x+x+2)+3x²=0

\(\Rightarrow\)x³+64-x³-2x²-x²-2x+3x²=0

\(\Rightarrow\)-2x+64=0

\(\Rightarrow\)-2x=-64

\(\Rightarrow\)x=\(\dfrac{-64}{-2}\)

\(\Rightarrow x=32\)

2)(8x+2)(1-3x)+(6x-1)(4x-10)=-50

\(\Rightarrow\)8x-24x²+2-6x+24x²-60x-4x+10=50

\(\Rightarrow\)-62x+12=50

\(\Rightarrow\)-62x=50-12

\(\Rightarrow\)-62x=38

\(\Rightarrow\)x=\(-\dfrac{38}{62}=-\dfrac{19}{31}\)

3)(x²+2x+4)(2-x)+x(x-3)(x+4)-x²+24=0

\(\Rightarrow\)8-x³+x(x²+4x-3x-12)-x²+24=0

\(\Rightarrow\)8-x³+x³+4x²-3x²-12x-x²+21=0

\(\Rightarrow\)-12x+29=0

\(\Rightarrow\)-12x=-29

\(\Rightarrow\)x=\(\dfrac{-29}{-12}=\dfrac{29}{12}\)

cac ban giai giup minh voi

:(((

b) \(\left(x-1\right)\left(x^2+x+1\right)-x\left(x-3\right)\left(x+3\right)=8\)

\(\Rightarrow x^3-1-x\left(x^2-9\right)=8\)

\(\Rightarrow x^3-1-x^3+9x=8\)

\(\Rightarrow9x=9\Rightarrow x=1\)

c) \(\left(x^2+2\right)\left(x-4\right)-\left(x+2\right)\left(x^2+4x+4\right)=-16\)

\(\Rightarrow x^3-4x^2+2x-8-\left(x+2\right)\left(x+2\right)^2=-16\)

\(\Rightarrow x^3-4x^2+2x-8-\left(x+2\right)^3=-16\)

\(\Rightarrow x^3-4x^2+2x-8-\left(x^3+6x^2+12x+8\right)=-16\)

\(\Rightarrow x^3-4x^2+2x-8-x^3-6x^2-12x-8=-16\)

\(\Rightarrow-10x^2-10x-16=-16\)

\(\Rightarrow10x^2+10x=0\)

\(\Rightarrow10x\left(x+1\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\x+1=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)

Đề bài bạn ơi

Giải pt à bạn:P?

\(\left(x+4\right)\left(x^2-4x+16\right)-\left(x-2\right)^3=0\)

\(\Leftrightarrow x^3+4^3-\left(x^3-8-6x^2+12x\right)=0\)

\(\Leftrightarrow x^3+4^3-x^3+8+6x^2-12x=0\)

\(\Leftrightarrow72+6x^2-12x=0\Leftrightarrow6\left(x^2-2x+12\right)=0\Leftrightarrow x^2-2x+12=0\)

Ta lại có: \(x^2-2x+12=x^2-2x+1+11=\left(x-1\right)^2+11\ge11>0\ne0\)

=> Pt vô nghiệm.

(x+4).(x²-4x+16)-(x-2)³

= x³ - 4x² + 16x + 4x² - 16x + 64 - (x³ -4x² + 8x - 4)

=  x³ - 4x² + 16x + 4x² - 16x + 64 - x³ +4x² - 8x + 4

=  (x³ - x³ ) + (- 4x²  + 4x² +4x² ) +(16x - 16x - 8x)+ (64  + 4)

= 4x² - 8x + 68

= 2(2x² - 4x + 34)

\(=x^3+64\\ =x^3-27y^3\\ =x^6-\dfrac{1}{27}\)

\(\left(x+4\right)\left(x^2-4x+16\right)=x^3+64\)

\(\left(x-3y\right)\left(x^2+3xy+9y^2\right)=x^3-27y^3\)

\(\left(x^2-\dfrac{1}{3}\right)\left(x^4+\dfrac{1}{3}x^2+\dfrac{1}{9}\right)=x^6-\dfrac{1}{27}\)

a) x 2  – x – 12                              b) x 3  – 64.

c) m 3 n 3   –   m 2 n   +   5 mn 2   –   5          d) 16 x 4  – 1.