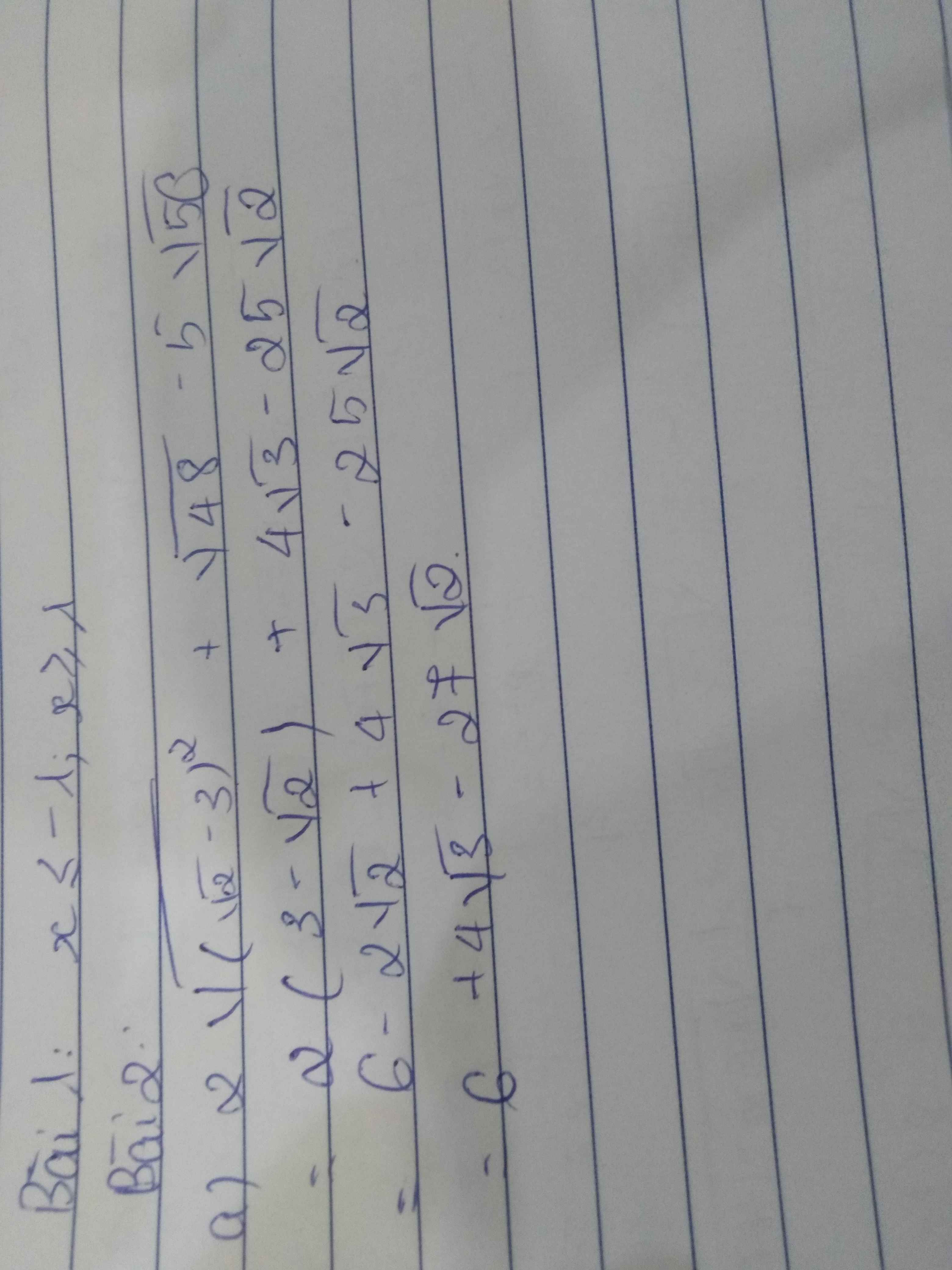`\sqrt{48}-\sqrt{3}=\sqrt{4^2 . 3}-\sqrt{3}=4\sqrt{3}-\sqrt{3}=3\sqrt{3}`

like playing soccer, don't you
goes to school late, doesn't he
can swim very well, can't you
is going to the party, isn't she
was published in Germany in 1550, wasn't it
are sold all over the world, aren't they
have been built this year, haven't they
was given a book, wasn't he 
were bought by Mrs Brown yesterday, weren't they
is used everyday, isn't it

a: ĐKXĐ: \(x>0\)

b: Ta có: \(A=\dfrac{x^2+\sqrt{x}}{x-\sqrt{x}+1}-\dfrac{2x+\sqrt{x}}{\sqrt{x}}+1\)

\(=x+\sqrt{x}-2\sqrt{x}-1+1\)

\(=x-\sqrt{x}\)

Bình phương 2 vế lên ta được:

\(x+\sqrt{x+\sqrt{x+\sqrt{x+...}}}=16\) 16

Kết hợp bài ra ta thu được x=12

đặt tính à bạn ơi?

18725:206=90 dư 185

3160:56=56 dư 3

41982:206=203 dư 82

Bài 1 : 

\(a.\sqrt{x^2-1}\)

\(ĐK:\)

\(x^2-1\ge0\)

\(\Leftrightarrow x^2\ge1\)

\(\Leftrightarrow\left[{}\begin{matrix}x\le-1\\x\ge1\end{matrix}\right.\)

Bài 2 : 

\(2\cdot\sqrt{\left(\sqrt{2}-3\right)^2}+\sqrt{48}-5\sqrt{50}\)

\(=2\cdot\left|\sqrt{2}-3\right|+4\sqrt{3}-25\sqrt{2}\)

\(=-2\cdot\left(\sqrt{2}-3\right)+4\sqrt{3}-25\sqrt{2}\)

\(=-2\sqrt{2}-6+4\sqrt{3}-25\sqrt{2}\)

\(=-27\sqrt{2}-6+4\sqrt{3}\)

\(\dfrac{1}{5}\sqrt[]{25x+50}-5\sqrt[]{x+2}+\sqrt[]{9x+18}+9=0\)

\(\Leftrightarrow\dfrac{1}{5}\sqrt[]{25\left(x+2\right)}-5\sqrt[]{x+2}+\sqrt[]{9\left(x+2\right)}+9=0\)

\(\Leftrightarrow\dfrac{1}{5}.5\sqrt[]{x+2}-5\sqrt[]{x+2}+3\sqrt[]{x+2}+9=0\)

\(\Leftrightarrow\sqrt[]{x+2}-5\sqrt[]{x+2}+3\sqrt[]{x+2}+9=0\)

\(\Leftrightarrow\sqrt[]{x+2}\left(1-5+3\right)+9=0\)

\(\Leftrightarrow-\sqrt[]{x+2}+9=0\)

\(\Leftrightarrow\sqrt[]{x+2}=9\)

\(\Leftrightarrow x+2=81\)

\(\Leftrightarrow x=79\)

Đặt \(A=\sqrt[3]{2+\sqrt{5}}+\sqrt[3]{2-\sqrt{5}}\)

Áp dụng \(\left(a+b\right)^3=a^3+b^3+3ab\left(a+b\right)\) ta có:

\(\sqrt[3]{2+\sqrt{5}}+\sqrt[3]{2-\sqrt{5}}\)

\(A^3=2+\sqrt{5}+2-\sqrt{5}+3\sqrt[3]{4-5}\left(\sqrt[3]{2+\sqrt{5}}+\sqrt[3]{2-\sqrt{5}}\right)\)

\(=4-3A\)

Giải PT:

\(A^3+3A-4=0\Leftrightarrow A^3-1+3A-3=0\)\(\Leftrightarrow\left(A-1\right)\left(A^2+A+1\right)+3\left(A-1\right)=0\)\(\Leftrightarrow\left(A-1\right)\left(A^2+A+4\right)=0\)\(\Leftrightarrow\orbr{\begin{cases}A-1=0\\A^2+A+4=0\end{cases}\Leftrightarrow\orbr{\begin{cases}A=1\\A^2+2.\frac{1}{2}A+\frac{1}{4}-\frac{1}{4}+4=0\end{cases}}}\)

\(\Leftrightarrow\orbr{\begin{cases}A=1\\\left(A+\frac{1}{2}\right)^2+\frac{15}{4}=0\end{cases}\Leftrightarrow\orbr{\begin{cases}A=1\\\left(A+\frac{1}{2}\right)^2=-\frac{15}{4}\left(L\right)\end{cases}}}\)

Vậy \(A=1\)

\(=\dfrac{\sqrt{a}+2+\sqrt{a}-2}{a-4}:\dfrac{\sqrt{a}+2-2}{\sqrt{a}+2}\)

\(=\dfrac{2\sqrt{a}}{a-4}\cdot\dfrac{\sqrt{a}+2}{\sqrt{a}}=\dfrac{2}{\sqrt{a}-2}\)