Giúp mik với ạaaa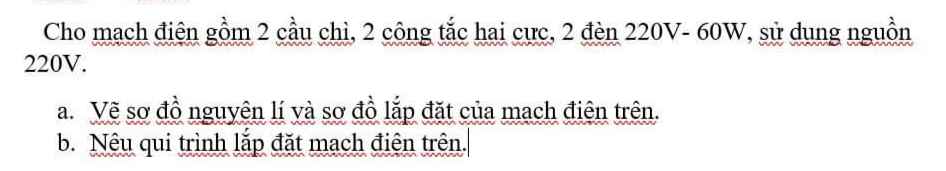

Những câu hỏi liên quan
Giúp mik với ạaaa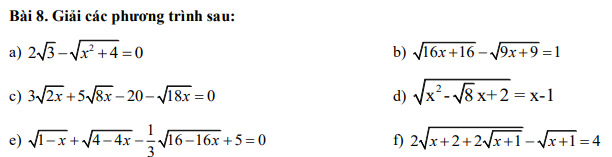
a.
$2\sqrt{3}-\sqrt{x^2+4}=0$
$\Leftrightarrow 2\sqrt{3}=\sqrt{x^2+4}$
$\Leftrightarrow 12=x^2+4$
$\Leftrightarrow 8=x^2$
$\Leftrightarrow x=\pm 2\sqrt{2}$
b. ĐKXĐ: $x\geq -1$
$\sqrt{16(x+1)}-\sqrt{9(x+1)}=1$
$\Leftrightarrow 4\sqrt{x+1}-3\sqrt{x+1}=1$
$\Leftrightarrow \sqrt{x+1}=1$
$\Leftrightarrow x+1=1$
$\Leftrightarrow x=0$ (tm)
c. ĐKXĐ: $x\geq 0$
$3\sqrt{2x}+5\sqrt{8x}-20-\sqrt{18x}=0$
$\Leftrightarrow 3\sqrt{2x}+10\sqrt{2x}-20-3\sqrt{2x}=0$
$\Leftrightarrow 10\sqrt{2x}-20=0$
$\Leftrightarrow \sqrt{2x}=2$
$\Leftrightarrow 2x=4$
$\Leftrightarrow x=2$ (tm)
Đúng 0
Bình luận (0)
d.
PT \(\Rightarrow \left\{\begin{matrix} x-1\geq 0\\ x^2-\sqrt{8x}+2=(x-1)^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 1\\ x=\frac{1+\sqrt{2}}{2}\end{matrix}\right.\Leftrightarrow x=\frac{1+\sqrt{2}}{2}\)
e. ĐKXĐ: $x\leq 1$
PT $\Leftrightarrow \sqrt{1-x}+2\sqrt{1-x}-\frac{4}{3}\sqrt{1-x}+5=0$
$\Leftrightarrow \frac{5}{3}\sqrt{1-x}=-5< 0$ (vô lý)
Vậy pt vô nghiệm.
f. ĐKXĐ: $x\geq -1$
PT $\Leftrightarrow 2\sqrt{(\sqrt{x+1}+1)^2}-\sqrt{x+1}=4$
$\Leftrightarrow 2(\sqrt{x+1}+1)-\sqrt{x+1}=4$
$\Leftrightarrow \sqrt{x+1}=2$
$\Leftrightarrow x=3$ (tm)
Đúng 0
Bình luận (0)
a) Ta có: \(2\sqrt{3}-\sqrt{x^2+4}=0\)
\(\Leftrightarrow\sqrt{x^2+4}=2\sqrt{3}\)
\(\Leftrightarrow x^2+4=12\)
\(\Leftrightarrow x^2=8\)
hay \(x\in\left\{2\sqrt{2};-2\sqrt{2}\right\}\)
b) Ta có: \(\sqrt{16x+16}-\sqrt{9x+9}=1\)
\(\Leftrightarrow4\sqrt{x+1}-3\sqrt{x+1}=1\)
\(\Leftrightarrow\sqrt{x+1}=1\)
\(\Leftrightarrow x+1=1\)
hay x=0
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Giúp với ạaaa mik cần gấp lắm ạ
 Giúp mik ạaaa
Giúp mik ạaaa
He was dehydrated because he had not drunk water in two days
Her family was worried because she had not come home
He couldn't call for help because the battery in phone had died
She was lost because she had wandered from the path
Đúng 4
Bình luận (0)
Giúp e với ạaaa
Lời giải:
a. Để $f(x)=x^2-2mx+3m+4\geq 0$ với mọi $x\in\mathbb{R}$ thì:
\(\left\{\begin{matrix}
a=1>0\\
\Delta'=m^2-3m-4\leq 0\end{matrix}\right.\Leftrightarrow m^2-3m-4\leq 0\)
$\Leftrightarrow (m+1)(m-4)\leq 0$
$\Leftrightarrow -1\leq m\leq 4$
b.
Để pt có 2 nghiệm pb cùng dấu thì:
\(\left\{\begin{matrix}
\Delta'=m^2-3m-4>0\\
P=3m+4>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix}
(m+1)(m-4)> 0\\
m> \frac{-4}{3}\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix}
m> 4 \text{hoặc} m< -1\\
m> \frac{-4}{3}\end{matrix}\right.\)
$\Leftrightarrow m>4$ hoặc $\frac{-4}{3}< m < -1$
Đúng 1
Bình luận (0)
Lời giải:
a. Để $f(x)=x^2-2mx+3m+4\geq 0$ với mọi $x\in\mathbb{R}$ thì:
\(\left\{\begin{matrix}
a=1>0\\
\Delta'=m^2-3m-4\leq 0\end{matrix}\right.\Leftrightarrow m^2-3m-4\leq 0\)
$\Leftrightarrow (m+1)(m-4)\leq 0$
$\Leftrightarrow -1\leq m\leq 4$
b.
Để pt có 2 nghiệm pb cùng dấu thì:
\(\left\{\begin{matrix}
\Delta'=m^2-3m-4>0\\
P=3m+4>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix}
(m+1)(m-4)> 0\\
m> \frac{-4}{3}\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix}
m> 4 \text{hoặc} m< -1\\
m> \frac{-4}{3}\end{matrix}\right.\)
$\Leftrightarrow m>4$ hoặc $\frac{-4}{3}< m < -1$
giúp e với ạaaa
Đọc tiếp
giúp e với ạaaa
Gọi chữ số hàng chục là x và chữ số hàng đơn vị là y (x;y là các chữ số từ 0 đến 9)
Do chữ số hàng chục hơn chữ số hàng đơn vị là 4
\(\Rightarrow x-y=4\)
Giá trị chữ số ban đầu: \(10x+y\)
Giá trị chữ số sau khi đổi chỗ: \(10y+x\)
Do tổng số mới và số cũ là 132 nên ta có pt:
\(10x+y+10y+x=132\Rightarrow11\left(x+y\right)=132\Rightarrow x+y=12\)
Ta được hệ: \(\left\{{}\begin{matrix}x-y=4\\x+y=12\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=8\\y=4\end{matrix}\right.\)
Vậy số đó là 84
Đúng 1
Bình luận (1)
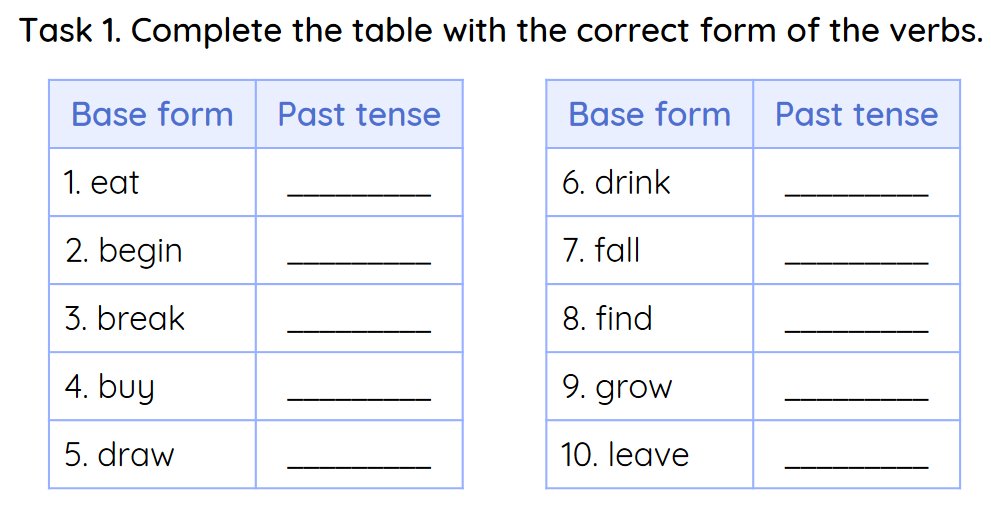
giúp em với ạaaa
`@` `\text {Ans}`
`\downarrow`
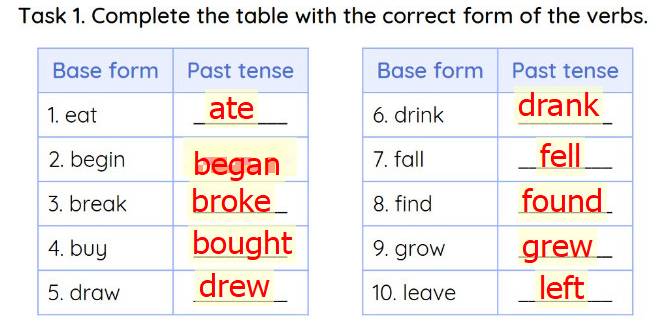
Đúng 3
Bình luận (0)

giúp em với ạaaa
`@` `\text {Ans}`
`\downarrow`
1. Ago
2. Last
3. Ago
4. Ago
5. Last
Đúng 2
Bình luận (0)
 Giúp với m.n ạaaa
Giúp với m.n ạaaa
 Giải giúp e với ạaaa
Giải giúp e với ạaaa
Gọi số học sinh nam và số học sinh nữ lần lượt là a,b
Theo đề, ta có: a+b=46 và 2a+3b=118
=>a=20 và b=26
Đúng 0
Bình luận (0)













