Chứng minh các đa thức sau không có nghiệm
4x2 - 4x + 2
9x2 + 6x + 3
Những câu hỏi liên quan
chuwmgs minh đa thức sau ko có nghiệm 9x2+6x+2
cho hai đa thức : P(x)=5x^3+6x^2-9x+4 . Q(x)=-5x^3-4x^2+9x+5 . chứng minh rằng : không tồn tại giá trị nào của x để hai đa thức P(x) và Q(x) có cùng giá trị không dương
Chứng minh các đa thức sau không có nghiệm :
a) 8^2+11x+3
b)4x^2-3x-1
b) 4x2 - 3x - 1
vì 4x2 lớn hơn hoặc bằng 0
=> 4x2 - 3x - 1 lớn hơn hoặc bằng 1 > 0
=> đa thức này ko có nghiệm
t i c k mk nhoa oa oa buồn ngủ rùi ^ 0 ^ !!!!
Đúng 0
Bình luận (0)
Rút gọn rồi tính giá trị các biểu thức sau: 4 x - 9 x 2 + 6 x + 1 t ạ i x = - 3
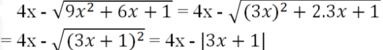
Tại x = -√3 ta được:
= 4(-√3) - |3(-√3) + 1|
= -4√3 - |-3√3 + 1|
= -4√3 - (3√3 - 1)
= -7√3 + 1
Đúng 0
Bình luận (0)
Chứng tỏ các đa thức sau không có nghiệm:
a) x^2+6x+10
b) x^2+4x+7
c) x^4+2x^2+1
a,x2+6x+10
=x2+3x+3x+3.3+1
=x(3+x)+3(3+x)+1
=(3+x)(3+x)+1
=(3+x)2+1
Vì (3+x)2>hoặc=0
=>(3+x)2+1>1
Vậy đa thức trên ko có ngiệm
Đúng 0
Bình luận (0)
a) x2 + 6x + 10
= x2 + 3x + 3x + 9 + 1
= x ( x + 3 ) + 3 ( x + 3 ) + 1
= ( x + 3 ).( x + 3 ) + 1
= ( x + 3 )2 + 1 . Vì ( x + 3 ) > 0 hoặc = 0 với mọi x
Vậy đa thức trên vô nghiệm
b) x2 + 4x + 7
= x2 + 2x + 2x + 4 + 3
= x ( x + 2 ) + 2 ( x + 2 ) + 3
= ( x + 2 ).( x + 2 ) + 3
= ( x + 2 )2 + 3 . Vì ( x + 2 )2 > 0 hoặc = 0 với mọi x
Vậy đa thức trên vô nghiệm
Đúng 0
Bình luận (0)
a)x2+6x+10
=(x+3)2+1
Mà (x+3)2>=0 với mọi x thuộc R nên
(x+3)2+1>=1 Vậy ...
b)x2+4x+7
=(x+2)2+3
Mà (x+2)2>=0 với mọi x thuộc R nên
(x+2)2+3>=3 Vậy ...
c) Tương tự nha
Đúng cho mình :))
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Chứng minh biểu thức sau không phụ thuộc vào biến
(2x+3)(4x²-6x+3)-2(4x²+3)
Xem chi tiết
(6x−5)(x+8)−(3x−1)(2x+3)−9(4x−3)=6x2+43x−40−6x2−7x+3−36x+27=−10
Chứng minh các biểu thức sau không phụ thuộc vào x:1, A3left(sin^4x+cos^4xright)-2left(sin^6x+cos^6xright)2, Bcos^6x+2sin^4x.cos^2x+3sin^2x.cos^4x+sin^4x3, Ccosleft(x-dfrac{pi}{3}right).cosleft(x+dfrac{pi}{4}right)+cosleft(x+dfrac{pi}{6}right).cosleft(x+dfrac{3pi}{4}right)4, Dcos^2x+cos^2left(x+dfrac{2pi}{3}right)+cos^2left(dfrac{2pi}{3}-xright)5, E2left(sin^4x+cos^4x+sin^2x.cos^2xright)-left(sin^8x+cos^8xright)6, Fcosleft(pi-xright)+sinleft(dfrac{-3pi}{2}+xright)-tanleft(dfrac{pi}{2}+xright).c...
Đọc tiếp
Chứng minh các biểu thức sau không phụ thuộc vào x:
1, \(A=3\left(sin^4x+cos^4x\right)-2\left(sin^6x+cos^6x\right)\)
2, \(B=cos^6x+2sin^4x.cos^2x+3sin^2x.cos^4x+sin^4x\)
3, \(C=cos\left(x-\dfrac{\pi}{3}\right).cos\left(x+\dfrac{\pi}{4}\right)+cos\left(x+\dfrac{\pi}{6}\right).cos\left(x+\dfrac{3\pi}{4}\right)\)
4, \(D=cos^2x+cos^2\left(x+\dfrac{2\pi}{3}\right)+cos^2\left(\dfrac{2\pi}{3}-x\right)\)
5, \(E=2\left(sin^4x+cos^4x+sin^2x.cos^2x\right)-\left(sin^8x+cos^8x\right)\)
6, \(F=cos\left(\pi-x\right)+sin\left(\dfrac{-3\pi}{2}+x\right)-tan\left(\dfrac{\pi}{2}+x\right).cot\left(\dfrac{3\pi}{2}-x\right)\)
1,\(A=3\left(sin^4x+cos^4x\right)-2\left(sin^2x+cos^2x\right)\left(sin^4x-sin^2x.cos^2x+cos^4x\right)\)
\(=3\left(sin^4x+cos^4x\right)-2\left(sin^4x-sin^2x.cos^4x+cos^4x\right)\)
\(=sin^4x+2sin^2x.cos^2x+cos^4x=\left(sin^2x+cos^2x\right)^2=1\)
Vậy...
2,\(B=cos^6x+2sin^4x\left(1-sin^2x\right)+3\left(1-cos^2x\right)cos^4x+sin^4x\)
\(=-2cos^6x+3sin^4x-2sin^6x+3cos^4x\)
\(=-2\left(sin^2x+cos^2x\right)\left(sin^4x-sin^2x.cos^2x+cos^4x\right)+3\left(cos^4x+sin^4x\right)\)
\(=-2\left(sin^4x-sin^2x.cos^2x+cos^4x\right)+3\left(cos^4x+sin^4x\right)\)\(=cos^4x+sin^4x+2sin^2x.cos^2x=1\)
Vậy...
3,\(C=\dfrac{1}{2}\left[cos\left(-\dfrac{7\pi}{12}\right)+cos\left(2x-\dfrac{\pi}{12}\right)\right]+\dfrac{1}{2}\left[cos\left(-\dfrac{7\pi}{12}\right)+cos\left(2x+\dfrac{11\pi}{12}\right)\right]\)
\(=cos\left(-\dfrac{7\pi}{12}\right)+\dfrac{1}{2}\left[cos\left(2x-\dfrac{\pi}{12}\right)+cos\left(2x+\dfrac{11\pi}{12}\right)\right]\)\(=\dfrac{-\sqrt{6}+\sqrt{2}}{4}+\dfrac{1}{2}\left[cos\left(2x-\dfrac{\pi}{12}\right)+cos\left(2x-\dfrac{\pi}{12}+\pi\right)\right]\)
\(=\dfrac{-\sqrt{6}+\sqrt{2}}{4}+\dfrac{1}{2}\left[cos\left(2x-\dfrac{\pi}{12}\right)-cos\left(2x-\dfrac{\pi}{12}\right)\right]\)\(=\dfrac{-\sqrt{6}+\sqrt{2}}{4}\)
Vậy...
4, \(D=cos^2x+\left(-\dfrac{1}{2}cosx-\dfrac{\sqrt{3}}{2}sinx\right)^2+\left(-\dfrac{1}{2}.cosx+\dfrac{\sqrt{3}}{2}.sinx\right)^2\)
\(=cos^2x+\dfrac{1}{4}cos^2x+\dfrac{\sqrt{3}}{4}cosx.sinx+\dfrac{3}{4}sin^2x+\dfrac{1}{4}cos^2x-\dfrac{\sqrt{3}}{4}cosx.sinx+\dfrac{3}{4}sin^2x\)
\(=\dfrac{3}{2}\left(cos^2x+sin^2x\right)=\dfrac{3}{2}\)
Vậy...
5, Xem lại đề
6,\(F=-cosx+cosx-tan\left(\dfrac{\pi}{2}+x\right).cot\left(\pi+\dfrac{\pi}{2}-x\right)\)
\(=tan\left(\pi-\dfrac{\pi}{2}-x\right).cot\left(\dfrac{\pi}{2}-x\right)\)\(=tan\left(\dfrac{\pi}{2}-x\right).cot\left(\dfrac{\pi}{2}-x\right)\)\(=cotx.tanx=1\)
Vậy...
Đúng 1
Bình luận (0)
Bài 1: Chứng minh các biểu thức sau không phụ thuộc vào biến x.
a/ (2x + 1)(4x – 3) – 6x(x + 5) – 2x(x – 7) + 18x
b/ 9x(2x – 5) – (6x + 2)(3x – 2) + 39x
c/ 4x(2x – 3) + x(x + 2) – 9x(x – 1) + x – 5
a/ (2x + 1)(4x – 3) – 6x(x + 5) – 2x(x – 7) + 18x
=8x^2-6x+4x-3-6x^2-30x-2x^2+14x+18x
=-3
vậy...
Đúng 2
Bình luận (0)
a) Ta có: \(\left(2x+1\right)\left(4x-3\right)-6x\left(x+5\right)-2x\left(x-7\right)+18x\)
\(=8x^2-6x+4x-3-6x^2-30x-2x^2+14x+18x\)
\(=-3\)
b) Ta có: \(9x\left(2x-5\right)-\left(6x+2\right)\left(3x-2\right)+39x\)
\(=18x^2-45x-18x^2+12x-6x+4+39x\)
\(=4\)
c) Ta có: \(4x\left(2x-3\right)+x\left(x+2\right)-9x\left(x-1\right)+x-5\)
\(=8x^2-12x+x^2+2x-9x^2+9x+x-5\)
\(=-5\)
Đúng 2
Bình luận (1)
Chứng tỏ đa thức không có nghiệm
\(4x^2+8x+10\)
\(x^2+4x+6\)
\(4x^{2010}+6x^{2012}+2021\)
