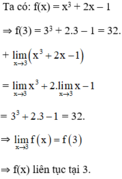Hàm số 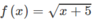 có tập xác định là [-5; +∞).

Do đó, nó xác định trên khoảng (-5; +∞) chứa x = 4

Vì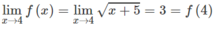  

nên f(x) liên tục tại x = 4

Vì hai làm lượng giác \(y = \sin x,y = \cos x\) liên tục trên \(\mathbb{R}\)

\( \Rightarrow f\left( x \right) = \sin x + \cos x\) liên tục trên \(\mathbb{R}\)

Ta có \(f\left( {{x_0}} \right) = f\left( 1 \right) = {1^3} + 1 = 2;\mathop {\lim }\limits_{x \to {x_0}} f\left( x \right) = \mathop {\lim }\limits_{x \to 1} \left( {{x^3} + 1} \right) = \mathop {\lim }\limits_{x \to 1} {x^3} + 1 = 1 + 1 = 2\)

\( \Rightarrow \mathop {\lim }\limits_{x \to 1} f\left( x \right) = f\left( 1 \right)\)

Vậy hàm số \(f\left( x \right)\) liên tục tại \({x_0} = 1.\)

+) Ta có :

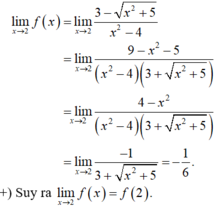
- Vậy hàm số đã cho liên tục tại x = 2.

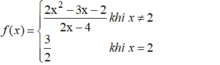
- Tập xác định D = R.

- Ta có: f(2) = 3/2.

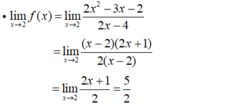
- Vì  nên hàm số không liên tục tại x = 2.

\(\lim\limits_{x\rightarrow2}f\left(x\right)=\lim\limits_{x\rightarrow2}\dfrac{x^2+x-6}{x-2}=\lim\limits_{x\rightarrow2}\dfrac{\left(x-2\right)\left(x+3\right)}{x-2}=\lim\limits_{x\rightarrow2}\left(x+3\right)=5\\ f\left(2\right)=5\\ \rightarrow\lim\limits_{x\rightarrow2}f\left(x\right)=f\left(2\right)\)

Suy ra f(x) liên tục tại x = 2.

Hàm số \(f\left( x \right) = 2{x^3} + x + 1\) xác định trên \(\mathbb{R}\).

Ta có: \(\begin{array}{l}\mathop {\lim }\limits_{x \to 2} f\left( x \right) = \mathop {\lim }\limits_{x \to 2} \left( {2{x^3} + x + 1} \right) = {2.2^3} + 2 + 1 = 17\\f\left( 2 \right) = {2.2^3} + 2 + 1 = 17\\ \Rightarrow \mathop {\lim }\limits_{x \to 2} f\left( x \right) = f\left( 2 \right)\end{array}\)

Do đó hàm số liên tục tại x = 2.

Xét tính liên tục của hàm số sau trên tập xác định của nó:

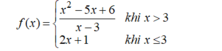
● Hàm số liên tục với mọi x ≠ 3.

● Tại x = 3, ta có:

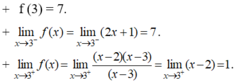
⇒ Hàm số không liên tục tại x = 3.

- Vậy hàm số liên tục trên các khoảng (-∞ ; 3), (3 ; +∞).

1.

\(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x+2}-\sqrt{2-x}}{x}=\lim\limits_{x\rightarrow0}\dfrac{2x}{x\left(\sqrt{x+2}+\sqrt{2-x}\right)}=\lim\limits_{x\rightarrow0}\dfrac{2}{\sqrt{x+2}+\sqrt{2-x}}=\dfrac{2}{2\sqrt{2}}=\dfrac{\sqrt{2}}{2}\)

Vậy cần bổ sung \(f\left(0\right)=\dfrac{\sqrt{2}}{2}\) để hàm liên tục tại \(x=0\)

2.

a. \(f\left(0\right)=\lim\limits_{x\rightarrow0^-}f\left(x\right)=\lim\limits_{x\rightarrow0^-}\left(x+\dfrac{3}{2}\right)=\dfrac{3}{2}\)

\(\lim\limits_{x\rightarrow0^+}f\left(x\right)=\lim\limits_{x\rightarrow0^+}\dfrac{\sqrt{x+1}-1}{\sqrt[3]{1+x}-1}=\lim\limits_{x\rightarrow0^+}\dfrac{x\left(\sqrt[3]{\left(x+1\right)^2}+\sqrt[3]{x+1}+1\right)}{x\left(\sqrt[]{x+1}+1\right)}\)

\(=\lim\limits_{x\rightarrow0^+}\dfrac{\sqrt[3]{\left(x+1\right)^2}+\sqrt[3]{x+1}+1}{\sqrt[]{x+1}+1}=\dfrac{3}{2}\)

\(\Rightarrow f\left(0\right)=\lim\limits_{x\rightarrow0^+}f\left(x\right)=\lim\limits_{x\rightarrow0^-}f\left(x\right)\) nên hàm liên tục tại \(x=0\)

2b.

\(\lim\limits_{x\rightarrow1^-}f\left(x\right)=\lim\limits_{x\rightarrow1^-}\dfrac{x^3-x^2+2x-2}{x-1}=\lim\limits_{x\rightarrow1^-}\dfrac{x^2\left(x-1\right)+2\left(x-1\right)}{x-1}\)

\(=\lim\limits_{x\rightarrow1^-}\dfrac{\left(x^2+2\right)\left(x-1\right)}{x-1}=\lim\limits_{x\rightarrow1^-}\left(x^2+2\right)=3\)

\(\lim\limits_{x\rightarrow1^+}f\left(x\right)=f\left(1\right)=\lim\limits_{x\rightarrow1^+}\left(3x+a\right)=a+3\)

- Nếu \(a=0\Rightarrow f\left(1\right)=\lim\limits_{x\rightarrow1^-}f\left(x\right)=\lim\limits_{x\rightarrow1^+}f\left(x\right)\) hàm liên tục tại \(x=1\)

- Nếu \(a\ne0\Rightarrow\lim\limits_{x\rightarrow1^-}f\left(x\right)\ne\lim\limits_{x\rightarrow1^+}f\left(x\right)\Rightarrow\) hàm không liên tục tại \(x=1\)