Rút gọn biểu thức với điều kiện đã cho của x rồi tính giá trị của nó: x - 2 4 3 - x 2 + x 2 - 1 x - 3 (x < 3) tại x = 0,5

Những câu hỏi liên quan
Rút gọn biểu thức với điều kiện đã cho của x rồi tính giá trị của nó:
4
x
-
8
+
x
3
+
2
x
2
x
+
2
(x -2) tại x
-
2
Đọc tiếp
Rút gọn biểu thức với điều kiện đã cho của x rồi tính giá trị của nó: 4 x - 8 + x 3 + 2 x 2 x + 2 (x > -2) tại x = - 2
Ta có:
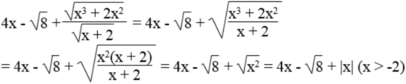
* Nếu x > 0 thì |x| = x
Ta có: 4x - 8 + |x| = 4x - 8 +x = 5x - 8
Với x = - 2 ta có: 5(- 2 ) - 8 = -5 2 - 2 2 = -7 2
* Nếu -2 < x < 0 thì |x| = -x
Ta có: 4x - 8 + |x| = 4x - 8 - x = 3x - 8
Với x = - 2 ta có: 3(- 2 ) - 8 = -3 2 - 2 2 = -5 2
Đúng 0
Bình luận (0)
Bài 2: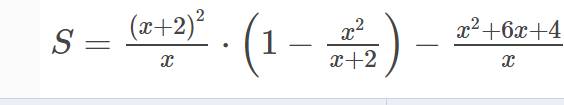
a) tìm điều kiện xác định của biểu thức S
b) Rút gọn rồi tính giá trị của biểu thức S tại x=0;1
c) tìm giá trị lớn nhất của biểu thức S
a) ĐKXĐ: \(x\ne0;x\ne-2\)
b) \(S=\dfrac{\left(x+2\right)^2}{x}\cdot\left(1-\dfrac{x^2}{x+2}\right)-\dfrac{x^2+6x+4}{x}\)
\(=\dfrac{\left(x+2\right)^2}{x}\cdot\dfrac{x+2-x^2}{x+2}-\dfrac{x^2+6x+4}{x}\)
\(=\dfrac{\left(x+2\right)\left(x+2-x^2\right)}{x}-\dfrac{x^2+6x+4}{x}\)
\(=\dfrac{x^2+2x-x^3+2x+4-2x^2-x^2-6x-4}{x}\)
\(=\dfrac{-x^3-2x^2-2x}{x}\)
\(=\dfrac{x\left(-x^2-2x-2\right)}{x}\)
\(=-x^2-2x-2\)
Với \(x=0\Rightarrow\) loại
Với \(x=1\), thay vào \(S\) ta được
\(S=-1^2-2\cdot1-2=-5\)
c) Có: \(S=-x^2-2x-2\)
\(=-\left(x^2+2x+2\right)\)
\(=-\left(x^2+2x+1\right)-1\)
\(=-\left(x+1\right)^2-1\)
Ta thấy: \(\left(x+1\right)^2\ge0\forall x\ne0;x\ne-2\)
\(\Rightarrow-\left(x+1\right)^2\le0\forall x\ne0;x\ne-2\)
\(\Rightarrow S=-\left(x+1\right)^2-1\le-1\forall x\ne0;x\ne-2\)
Dấu \("="\) xảy ra khi: \(x+1=0\Leftrightarrow x=-1\left(tmdk\right)\)
\(\text{#}\mathit{Toru}\)
Đúng 2
Bình luận (0)
Bài 1: Cho phân thức: 3x2+6x+12x3−83x2+6x+12x3−8a,Tìm điều kiện của x để phân thức đã cho được xác địnhb, Rút gọn phân thứcc, Tính giá trị của phân thức sau khi rút gọn với x 4001200040012000Bài 2: Cho phân thức: x2−10x+25x2−5xx2−10x+25x2−5xa, Tìm giá trị của x để phân thức bằng 0b, Tìm x để giá trị của phân thức bằng 5252c, Tìm x nguyên để phân thức có giá trị nguyênBài 3: Cho biểu thức: (x+12x−2+3x2−1−x+32x+2)(4x2−45)(x+12x−2+3x2−1−x+32x+2)(4x2−45)a, Tìm điều kiện của x để giá trị của biểu th...
Đọc tiếp
Bài 1: Cho phân thức: 3x2+6x+12x3−83x2+6x+12x3−8
a,Tìm điều kiện của x để phân thức đã cho được xác định
b, Rút gọn phân thức
c, Tính giá trị của phân thức sau khi rút gọn với x = 4001200040012000
Bài 2: Cho phân thức: x2−10x+25x2−5xx2−10x+25x2−5x
a, Tìm giá trị của x để phân thức bằng 0
b, Tìm x để giá trị của phân thức bằng 5252
c, Tìm x nguyên để phân thức có giá trị nguyên
Bài 3: Cho biểu thức: (x+12x−2+3x2−1−x+32x+2)(4x2−45)(x+12x−2+3x2−1−x+32x+2)(4x2−45)
a, Tìm điều kiện của x để giá trị của biểu thức được xác định
b, CMR: Khi giá trị của biểu thức được xác định thì nó không phụ thuộc vào giá trị của biến x
x2−10x+25x2−5x=(x−5)2x(x−5)=x−5x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x2−10x+25x2−5x=(x−5)2x(x−5)=x−5x
x−5x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x−5x phải có giá trị nguyên.
x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)
x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5
(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5
(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
2(x+1)25+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline-table; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2(x+1)25+185−25x2−45x
2(x2+2x+1)5+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2(x2+2x+1)5+185−25x2−45x
2x2+4x+25+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+25+185−25x2−45x
2x2+4x+2+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+2+185−25x2−45x
2x2+4x+205−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+205−25x2−45x
c) tự làm, đkxđ: x≠1;x≠−1
Cho biểu thức
a) Tìm điều kiện của x để biểu thức A xác định.
b) Rút gọn A
c) Tính giá trị của A khi x= -1
ĐK: \(x\ne\pm2\)
\(A=\left(\dfrac{x}{x^2-4}+\dfrac{2}{2-x}+\dfrac{1}{x+2}\right).\dfrac{x+2}{2}\)
\(=\left[\dfrac{x}{\left(x-2\right)\left(x+2\right)}-\dfrac{2\left(x+2\right)}{\left(x+2\right)\left(x-2\right)}+\dfrac{x-2}{\left(x+2\right)\left(x-2\right)}\right].\dfrac{x+2}{2}\)
\(=\dfrac{x-2x-2+x-2}{\left(x-2\right)\left(x+2\right)}.\dfrac{x+2}{2}\)
\(=\dfrac{2}{2-x}\)
Đúng 2
Bình luận (0)
rút gọn biểu thức với điều kiện đã cho của x rồi tính giá trị của nó:
a)\(\sqrt{\frac{\left(x-2\right)^4}{\left(3-x\right)^2}+\frac{x^2-1}{x-3}}ĐK:\left(x< 3;\right)tạix=0,5\)
b)\(4x-\sqrt{8}+\frac{\sqrt{X^3+2X^2}}{\sqrt{X+2}}ĐK:\left(X.-2\right)TẠIX=-\sqrt{2}\)
b) \(4x-\sqrt{8}+\frac{\sqrt{x^3+2x^2}}{\sqrt{x+2}}\)
\(=4x-\sqrt{8}+\frac{\sqrt{x^2\left(x+2\right)}}{x+2}\)
\(=4x-\sqrt{8}+\frac{x\left(x+2\right)}{x+2}\)
\(=4x-\sqrt{8}+x\)
\(=5x-\sqrt{8}\)
Với \(x=-\sqrt{2}\) ta có:
\(5x-\sqrt{8}=5\cdot\left(-\sqrt{2}\right)-\sqrt{4\cdot2}=-5\sqrt{2}-2\sqrt{2}=-7\sqrt{2}\)
Đúng 0
Bình luận (0)
Cho biểu thức A(dfrac{x+1}{X-1}-dfrac{x-1}{x+1}):dfrac{2x}{5x-5}a)Rút gọn biểu thức A b)Tính giá trị của biểu thức A biết x -3c) Tính giá trị của A biết |x-2|4-2xd)Với giá trị nào của x thì A 2e)Tìm điều kiện của x để A 0f)Tìm giá trị của x để A nhận giá trị nguyên g) Tìm điều kiện của x để A -1
Đọc tiếp
Cho biểu thức A=(\(\dfrac{x+1}{X-1}-\dfrac{x-1}{x+1}\)):\(\dfrac{2x}{5x-5}\)
a)Rút gọn biểu thức A
b)Tính giá trị của biểu thức A biết x =-3
c) Tính giá trị của A biết |x-2|=4-2x
d)Với giá trị nào của x thì A =2
e)Tìm điều kiện của x để A <0
f)Tìm giá trị của x để A nhận giá trị nguyên
g) Tìm điều kiện của x để A >-1
a)
A=\(\left(\dfrac{x+1}{x-1}-\dfrac{x-1}{x+1}\right)\div\dfrac{2x}{5x-5}\)
\(\Leftrightarrow\left(\dfrac{x+1}{x-1}-\dfrac{x-1}{x+1}\right)\div\dfrac{2x}{5\left(x-1\right)}\)
ĐKXĐ: \(\left\{{}\begin{matrix}x-1\ne0\\x+1\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0+1\\x=0-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\x=-1\end{matrix}\right.\)
MTC: 5(x-1)(x+1)
\([\dfrac{5\left(x+1\right)\left(x+1\right)}{5\left(x-1\right)\left(x+1\right)}-\dfrac{5\left(x-1\right)\left(x-1\right)}{5\left(x-1\right)\left(x+1\right)}]\div\dfrac{2x\left(x+1\right)}{5\left(x-1\right)\left(x+1\right)}\)
\(\Rightarrow[5\left(x+1\right)\left(x+1\right)-5\left(x-1\right)\left(x-1\right)]\div2x\left(x+1\right)\)
\(\Leftrightarrow[5\left(x+1\right)^2-5\left(x-1\right)^2]\div2x^2+2x\)
\(\Leftrightarrow[5\left(x^2+2x+1\right)-5\left(x^2-2x+1\right)]\div2x^2+2x\)
\(\Leftrightarrow(5x^2+10x+5-5x^2+10x-5)\div2x^2+2x\)
\(\Leftrightarrow20x\div\left(2x^2+2x\right)\)
\(\Leftrightarrow10x+10\)
Đúng 3
Bình luận (0)
cho biểu thức A=(2+x/2-x - 4x^2/x^2+4) - 2-x/2+x):(x^2-3x/2x^2-x^3)
a)tìm điều kiện xác định rồi rút gọn biểu thức A
b)tìm giá trị của x để A>0
C)tính giá trị của A khi x thỏa mãn |x-7|=4
Cho biểu thức với . a) Rút gọn biểu thức ; b) Tìm điều kiện của để ; c) Tìm các giá trị nguyên của để có giá trị nguyên; d)* Với , hãy tìm giá trị lớn nhất của . Bài 8: Cho biểu thức ; với . a) Tính giá trị biểu thức khi . b) Rút gọn biểu thức . c) So sánh với 1. d) Tìm để có giá trị nguyên.
Cho biểu thức A=x2-4x+4/5x-10
a) Với điều kiện nào của x thì giá trị biểu thức của A được xác định
b) Rút gọn biểu thức A
c) Tìm giá trị của biểu thức A tại x=-2018
a) \(A=\dfrac{x^2-4x+4}{5x-10}.\) ĐK: \(x\ne2.\)
b) \(A=\dfrac{x^2-4x+4}{5x-10}=\dfrac{\left(x-2\right)^2}{5\left(x-2\right)}=\dfrac{x-2}{5}.\)
c) \(Thay\) \(x=-2018:\) \(\dfrac{-2018-2}{5}=-404.\)
Đúng 1
Bình luận (0)






















