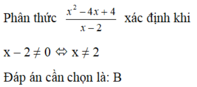a) ĐKXĐ:

$\begin{cases}1-2x\ge 0\\3-4x\ge 0\end{cases}\\\Leftrightarrow \begin{cases}2x\le 1\\4x\le 3\end{cases}\\\Leftrightarrow \begin{cases}x\le \dfrac{1}{2}\\x\le \dfrac{3}{4}\end{cases}\\\Leftrightarrow x\le \dfrac{1}{2}$

b) ĐKXĐ:

$\begin{cases}1+x\ge 0\\-4x\ge 0\end{cases}\\\Leftrightarrow \begin{cases}x\ge -1\\x\le 0\end{cases}\\\Leftrightarrow-1\le x\le 0$

\(ĐK:4x^2-12x+9=\left(2x-3\right)^2\ge0\) (luôn đúng) hay \(ĐK:x\in R\)

\(a,ĐK:2-x^2\ge0\Leftrightarrow x^2\le2\Leftrightarrow-\sqrt{2}\le x\le\sqrt{2}\\ b,ĐK:5x^2-3>0\Leftrightarrow x^2>\dfrac{3}{5}\Leftrightarrow\left[{}\begin{matrix}x>\dfrac{\sqrt{15}}{5}\\x< -\dfrac{\sqrt{15}}{5}\end{matrix}\right.\\ c,ĐK:-\left(2x-1\right)^2\ge0\Leftrightarrow x=\dfrac{1}{2}\\ d,ĐK:x^2+x-2>0\\ \Leftrightarrow\left(x-1\right)\left(x+2\right)>0\\ \Leftrightarrow\left[{}\begin{matrix}x>1\\x< -2\end{matrix}\right.\)

 \(\sqrt{4x^2-4x+1}=0\Rightarrow\sqrt{\left(2x-1\right)^2}=0\Rightarrow2x-1=0\Rightarrow x=\frac{1}{2}\)

Vậy ĐKCĐ: \(x\ge\frac{1}{2}\)

\(A=\frac{\sqrt{4x^2-4x+1}}{4x^2-1}=\frac{\sqrt{\left(2x-1\right)^2}}{4x^2-1}=\frac{2x-1}{\left(2x-1\right)\left(2x+1\right)}=\frac{1}{2x+1}\)

Để phân thức xác định: 

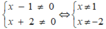
Vậy điều kiện để phân thức xác định là x ≠ -2 và x ≠ 1

Mình làm thử, bạn xem có đúng hông nha!

   \(ĐKXĐ:\hept{\begin{cases}4x+2\ge0\\x^2+4x+1\ge0\end{cases}\Leftrightarrow\hept{\begin{cases}x\ge-\frac{1}{2}\\\left(x+2\right)^2-3\ge-3\Leftrightarrow x=-2\end{cases}\Leftrightarrow}x\ge-\frac{1}{2}}\)

Mình giải thử lun nha!

  \(\sqrt{4x+2}=\sqrt{x^2+4x+1}\) (1)

Bình phương cả 2 vế của pt, ta được:

  \(\left(1\right)\Leftrightarrow\left(\sqrt{4x+2}\right)^2=\left(\sqrt{x^2+4x+1}\right)^2\)

\(\Leftrightarrow4x+2=x^2+4x+1\)

\(\Leftrightarrow x^2-1=0\)

\(\Leftrightarrow\left(x-1\right)\left(x+1\right)=0\)

\(\Leftrightarrow\hept{\begin{cases}x-1=0\\x+1=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=1\left(\text{nhận }\right)\\x=-1\left(\text{loại}\right)\end{cases}}}\)

Vậy: \(S=\left\{1\right\}\)

(Nếu đúng thì tíck cho mìk vs nhé!)

-4x² + 4x - 1 = -[(2x)² - 2.2x.1 + 1²] = -(2x - 1)².

-(2x - 1)² \(\ge0\)(biểu thức lấy căn) mà -(2x - 1)²\(\le0\)(vì\(\left(2x-1\right)^2\ge0\))

\(\Rightarrow-\left(2x-1\right)^2=0\Rightarrow2x-1=0\Rightarrow x=0,5\)