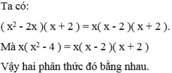Ba phân thức sau có bằng nhau không?
\(\dfrac{x^2-2x-3}{x^2+x};\dfrac{x-3}{x};\dfrac{x^2-4x+3}{x^2-x}\)

Những câu hỏi liên quan
Ba phân thức sau có bằng nhau không ?
\(\dfrac{x^2-2x-3}{x^2+x};\dfrac{x-3}{x};\dfrac{x^2-4x+3}{x^2-x}\)
\(\text{Ta có : }\dfrac{x^2-2x-3}{x^2+x}\\ =\dfrac{x^2+x-3x-3}{x\left(x+1\right)}\\ =\dfrac{\left(x^2+x\right)-\left(3x+3\right)}{x\left(x+1\right)}\\ \\ =\dfrac{x\left(x+1\right)-3\left(x+1\right)}{x\left(x+1\right)}\\ \\ =\dfrac{\left(x+1\right)\left(x-3\right)}{x\left(x+1\right)}\\ \\ =\dfrac{x-3}{x}\text{ }\text{ }\text{ }\left(1\right)\)
\(\dfrac{x^2-4x+3}{x^2-x}\\ =\dfrac{x^2-x-3x+3}{x\left(x-1\right)}\\ \\ =\dfrac{\left(x^2-x\right)-\left(3x-3\right)}{x\left(x-1\right)}\\ \\ =\dfrac{x\left(x-1\right)-3\left(x-1\right)}{x\left(x-1\right)}\\ \\ =\dfrac{\left(x-3\right)\left(x-1\right)}{x\left(x-1\right)}\\ \\ =\dfrac{x-3}{x}\text{ }\text{ }\left(2\right)\)
Từ \(\left(1\right)\) và \(\left(2\right)\) suy ra : \(\dfrac{x^2-2x-3}{x^2+x}=\dfrac{x-3}{x}=\dfrac{x^2-4x+3}{x^2-x}\)
Vậy 3 phân thức \(\dfrac{x^2-2x-3}{x^2+x};\dfrac{x-3}{x};\dfrac{x^2-4x+3}{x^2-x}\) bằng nhau
Đúng 0
Bình luận (0)
Giả sử :
\(\dfrac{x^2-2x-3}{x^2+x}=\dfrac{x-3}{x}=\dfrac{x^2-4x+3}{x^2-x}\)
\(\Leftrightarrow\) \(\dfrac{\left(x+1\right)\left(x-3\right)}{x\left(x+1\right)}=\dfrac{x-3}{x}=\dfrac{\left(x-1\right)\left(x-3\right)}{x\left(x-1\right)}\)
\(\Leftrightarrow\dfrac{x-3}{x}=\dfrac{x-3}{x}=\dfrac{x-3}{x}\)
Vậy 3 thức trên bằng nhau
Đúng 0
Bình luận (0)
1.Dùng tính chất của phân thức giải thích tại sao các cặp phân thức bằng nhaudfrac{3-x}{2-x}dfrac{x-3}{2-x} ; dfrac{x}{x-1}dfrac{x^2-x}{x^2-2x-1}2.Thực hiện phép tínha.dfrac{1+x}{x+1}-dfrac{x-1}{x^2+x} b.dfrac{2x}{x+23}.dfrac{3x}{x-1}+dfrac{2x}{x+23}.dfrac{23-2x}{x-1}3.Cho hình bình hành ABCD có góc A 60 độ và BC 2AB.Gọi M và N lần lượt là trung điểm của BC và DA.Trên tia đối tia BA lấy E sao cho BE BA.C/ma.AMDN là hbh b.ABMN là hình thoi c.AMNE
Đọc tiếp
1.Dùng tính chất của phân thức giải thích tại sao các cặp phân thức bằng nhau
\(\dfrac{3-x}{2-x}=\dfrac{x-3}{2-x}\) ; \(\dfrac{x}{x-1}=\dfrac{x^2-x}{x^2-2x-1}\)
2.Thực hiện phép tính
a.\(\dfrac{1+x}{x+1}-\dfrac{x-1}{x^2+x}\) b.\(\dfrac{2x}{x+23}.\dfrac{3x}{x-1}+\dfrac{2x}{x+23}.\dfrac{23-2x}{x-1}\)
3.Cho hình bình hành ABCD có góc A= 60 độ và BC = 2AB.Gọi M và N lần lượt là trung điểm của BC và DA.Trên tia đối tia BA lấy E sao cho BE =BA.C/m
a.AMDN là hbh b.ABMN là hình thoi c.AM=NE
Bài 2:
a: ĐKXĐ: \(x\notin\left\{0;-1\right\}\)
\(\dfrac{1+x}{x+1}-\dfrac{x-1}{x^2+x}\)
\(=\dfrac{x\left(x+1\right)-x+1}{x\left(x+1\right)}\)
\(=\dfrac{x^2+x-x+1}{x^2+x}=\dfrac{x^2+1}{x^2+x}\)
b: ĐKXĐ: \(x\notin\left\{-23;1\right\}\)
\(\dfrac{2x}{x+23}\cdot\dfrac{3x}{x-1}+\dfrac{2x}{x+23}\cdot\dfrac{23-2x}{x-1}\)
\(=\dfrac{2x}{x+23}\cdot\left(\dfrac{3x}{x-1}+\dfrac{23-2x}{x-1}\right)\)
\(=\dfrac{2x}{x+23}\cdot\dfrac{3x+23-2x}{x-1}\)
\(=\dfrac{2x}{x+23}\cdot\dfrac{x+23}{x-1}=\dfrac{2x}{x-1}\)
Bài 3:
a: Sửa đề: AMCN
Ta có: ABCD là hình bình hành
=>BC=AD(1)
Ta có: M là trung điểm của BC
=>\(BM=MC=\dfrac{BC}{2}\left(2\right)\)
Ta có: N là trung điểm của AD
=>\(NA=ND=\dfrac{AD}{2}\left(3\right)\)
Từ (1),(2),(3) suy ra BM=MC=NA=ND
Xét tứ giác AMCN có
MC//AN
MC=AN
Do đó: AMCN là hình bình hành
b: Xét tứ giác ABMN có
BM//AN
BM=AN
Do đó: ABMN là hình bình hành
Hình bình hành ABMN có \(AB=BM\left(=\dfrac{BC}{2}\right)\)
nên ABMN là hình thoi
c: Ta có: BM//AD
=>\(\widehat{EBM}=\widehat{EAD}\)(hai góc đồng vị)
=>\(\widehat{EBM}=60^0\)
Xét ΔBEM có BE=BM(=BA) và \(\widehat{EBM}=60^0\)
nên ΔBEM đều
=>\(\widehat{BEM}=60^0\)
Xét hình thang ANME có \(\widehat{MEA}=\widehat{EAN}=60^0\)
nên ANME là hình thang cân
=>AM=NE
Đúng 2
Bình luận (0)
Hai phân thức sau có bằng nhau không ? ( x 2 - 2 x ) ( x 2 - 4 ) và x ( x + 2 ) .
Mỗi cặp phân thức sau đây có bằng nhau không? Tại sao?
a) \(\dfrac{{x{y^2}}}{{xy + y}}\) và \(\dfrac{{xy}}{{x + 1}}\) b) \(\dfrac{{xy - y}}{x}\) và \(\dfrac{{xy - x}}{y}\)
`a, (xy^2)/(xy+y) = (xy^2)/(y(x+1))`
`=(xy)/(x+1)`
Vậy `2` cặp phân thức bằng nhau.
`b, (xy-y)/x = (y(x-1))/x = (y^2(x-1))/(xy)`
`(xy-x)/y = (x(y-1))/y = (x^2(y-1))/(xy)`
Vậy `2` đa thức không bằng nhau
Đúng 1
Bình luận (0)
Bài 2: Hai phân thức sau có bằng nhau không ?
a)x3-x2/x3-2x2+x và x/x-1
b)x2+2x+1/2x2-2 và x+1/2x-2
a) `(x^3-x^2)/(x^3-2x^2+x)`
`=(x^2(x-1))/(x(x-1)(x-1))`
`=x/(x-1)`
`=>` 2 phân thức bằng nhau.
b) `(x^2+2x+1)/(2x^2-2)`
`=((x+1)(x+1))/(2(x+1)(x-1))`
`=(x+1)/(2(x-1))`
`=(x+1)/(2x-2)`
`=>` 2 phân thức bằng nhau
Đúng 1
Bình luận (0)
a) Ta có: \(\dfrac{x^3-x^2}{x^3-2x^2+x}\)
\(=\dfrac{x^2\left(x-1\right)}{x\left(x^2-2x+1\right)}\)
\(=\dfrac{x\cdot\left(x-1\right)}{\left(x-1\right)^2}=\dfrac{x}{x-1}\)
b) Ta có: \(\dfrac{x^2+2x+1}{2x^2-2}\)
\(=\dfrac{\left(x+1\right)^2}{2\left(x+1\right)\left(x-1\right)}\)
\(=\dfrac{x+1}{2x-2}\)
Đúng 0
Bình luận (0)
Mỗi cặp phân thức sau đây có bằng nhau không? Tại sao?
a) \(\dfrac{xy^2}{xy-y}\) và \(\dfrac{xy}{x-1}\)
b) \(\dfrac{xy+y}{x}\) và \(\dfrac{xy+x}{y}\)
c) \(\dfrac{-6}{4y}\) và \(\dfrac{3y}{-2y^2}\)
a: \(\dfrac{xy^2}{xy-y}=\dfrac{y\cdot xy}{y\cdot\left(x-1\right)}=\dfrac{xy}{x-1}\)
=>Hai phân thức này bằng nhau
b: \(\dfrac{xy+y}{x}=\dfrac{y\left(x+1\right)}{x}\)
\(\dfrac{xy+x}{y}=\dfrac{x\left(y+1\right)}{y}\)
Vì \(\dfrac{y\left(x+1\right)}{x}\ne\dfrac{x\left(y+1\right)}{y}\)
nên hai phân thức này không bằng nhau
c: \(\dfrac{-6}{4y}=\dfrac{-6:2}{4y:2}=\dfrac{-3}{2y}\)
\(\dfrac{3y}{-2y^2}=\dfrac{-3y}{2y^2}=\dfrac{-3y}{y\cdot2y}=\dfrac{-3}{2y}\)
Do đó: \(\dfrac{-6}{4y}=\dfrac{3y}{-2y^2}\)
=>Hai phân thức này bằng nhau
Đúng 1
Bình luận (0)
Ba phân thức sau có bằng nhau không?
x2−2x−3x2+x,x2−2x−3x2+x,; x−3xx−3x ; x2−4x+3x2−xx2−4x+3x2−x.
Dùng định nghĩa hai phân thức bằng nhau, hãy tìm đa thức A trong mỗi đẳng thức sau :
a) \(\dfrac{A}{2x-1}=\dfrac{6x^2+3x}{4x^2-1}\)
b) \(\dfrac{4x^2-3x-7}{A}=\dfrac{4x-7}{2x+3}\)
c) \(\dfrac{4x^2-7x+3}{x^2-1}=\dfrac{A}{x^2+2x+1}\)
d) \(\dfrac{x^2-2x}{2x^2-3x-2}=\dfrac{x^2+2x}{A}\)
Ba phân thức
x
2
−
x
−
2
x
2
+
x
,
x
−
2
x
và
x
2
−
3...
Đọc tiếp
Ba phân thức x 2 − x − 2 x 2 + x , x − 2 x và x 2 − 3 x + 2 x 2 − x có bằng nhau không ? Tại sao?
Đưa về M = x − 1 ( x + 3 ) 2 N . Chọn N = ( x + 3 ) 2 Þ M = x - 1.
Đúng 0
Bình luận (0)