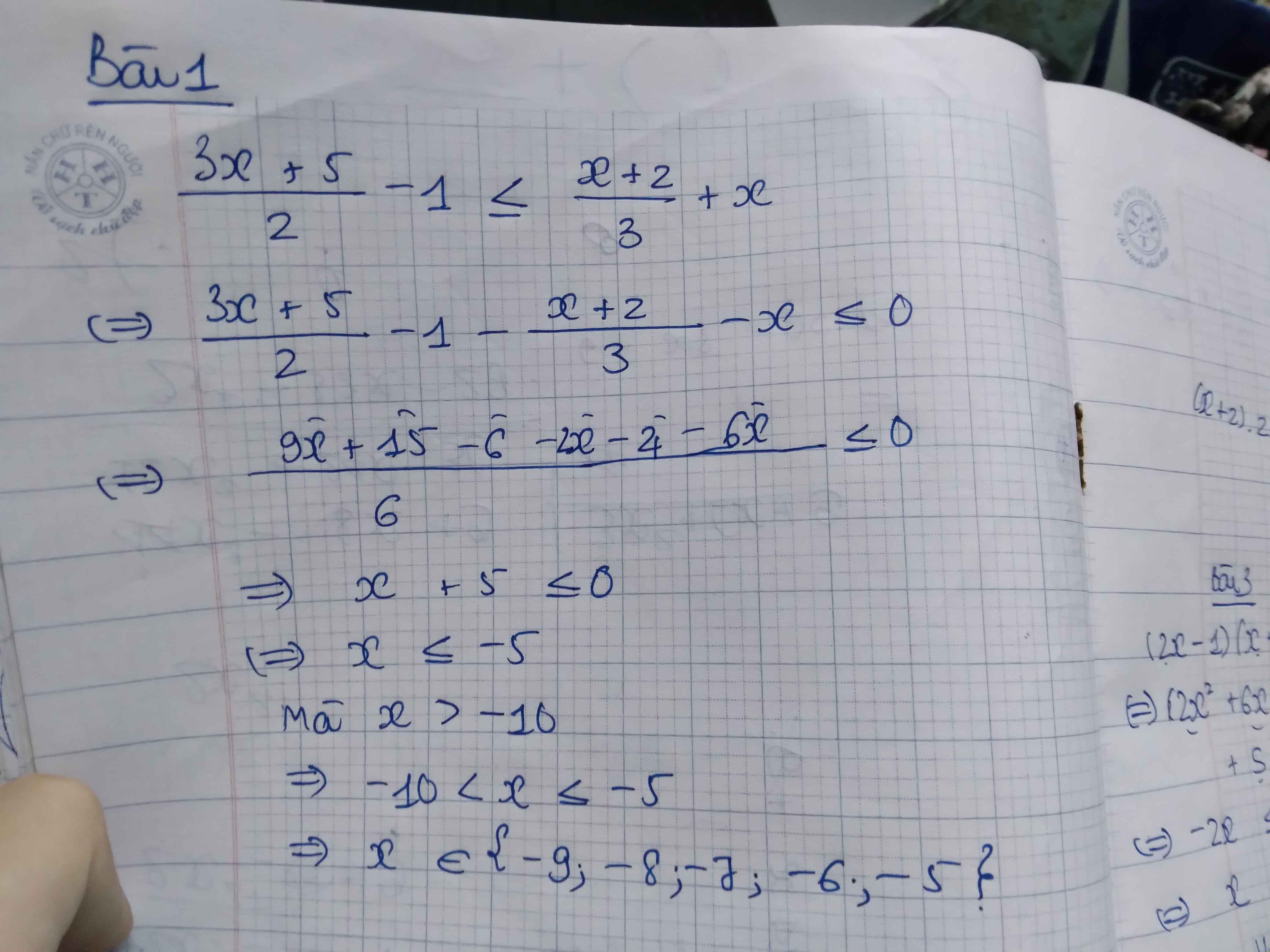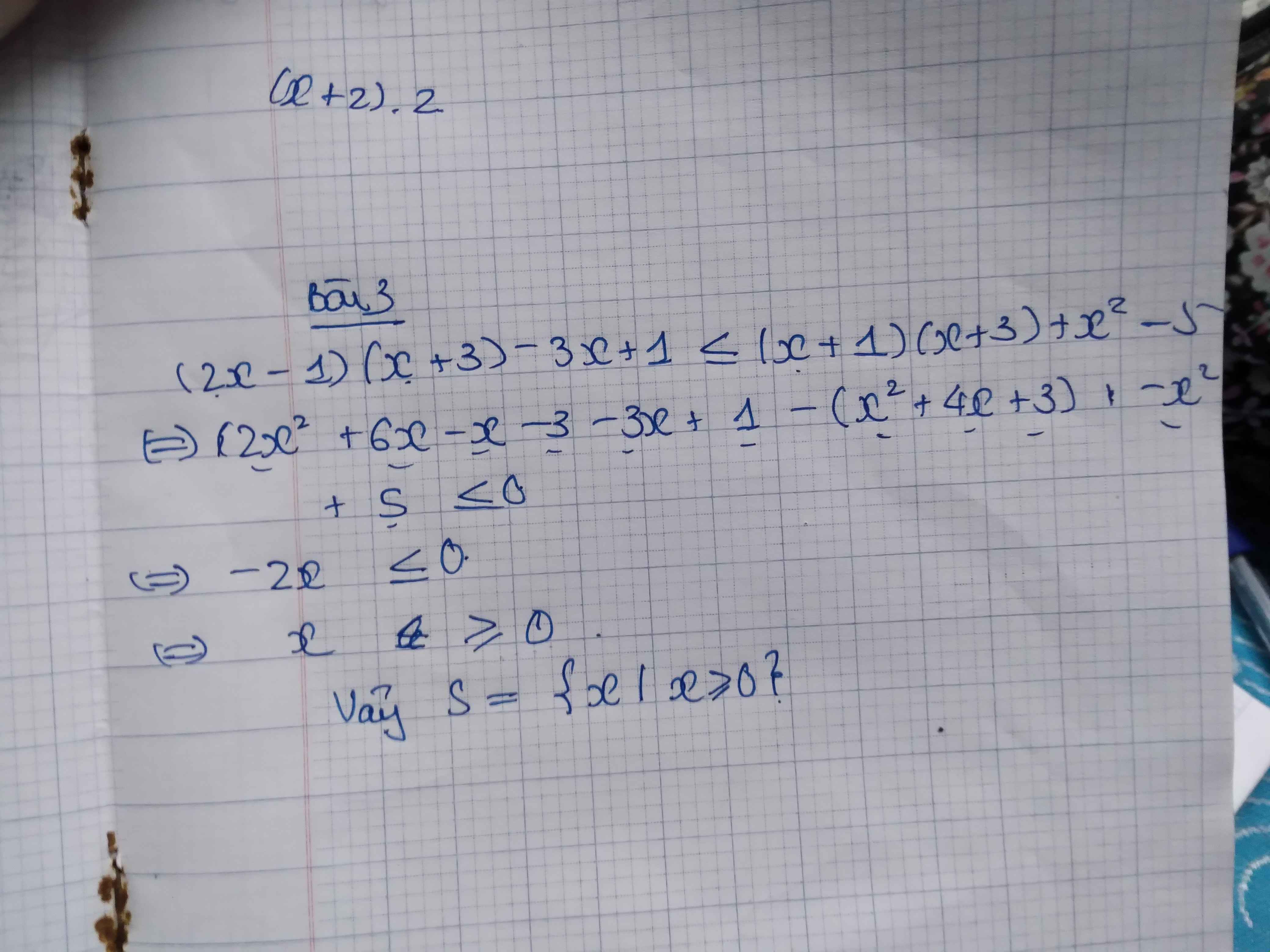1.

ĐKXĐ: \(x=2\)

Xét \(x=2\), bất phương trình vô nghiệm

\(\Rightarrow\) bất phương trình đã cho vô nghiệm

\(\Rightarrow\) Không tồn tại \(a,b\) thỏa mãn

Đề bài lỗi chăng.

\(f\left(x\right)=x+\dfrac{\sqrt{x}}{x+1}\Rightarrow f'\left(x\right)=1+\dfrac{1-x}{2\sqrt{x}\left(x+1\right)^2}\)

\(f'\left(x\right)-1>0\Leftrightarrow\dfrac{1-x}{2\sqrt{x}\left(x+1\right)^2}>0\)

\(\Rightarrow0< x< 1\)

Khi \(x\ge0\Rightarrow2x+1>0\) nên BPT tương đương:

\(x^2-3x+m>\left(2x+1\right)^2\)

\(\Leftrightarrow x^2-3x+m>4x^2+4x+1\)

\(\Leftrightarrow3x^2+7x+1< m\)

Xét hàm \(f\left(x\right)=3x^2+7x+1\) trên \(\left[0;2\right]\)

\(-\dfrac{b}{2a}=-\dfrac{7}{6}\notin\left[0;2\right]\) ; \(f\left(0\right)=1\) ; \(f\left(2\right)=27\)

\(\Rightarrow f\left(x\right)\ge1\Rightarrow\) pt có nghiệm trên đoạn đã cho khi \(m>1\)

Bài 1 :

Ta có : \(\dfrac{3x+5}{2}-1\le\dfrac{x+2}{3}+x\)

\(\Leftrightarrow\dfrac{3x+5}{2}-1-\dfrac{x+2}{3}-x\le0\)

\(\Leftrightarrow\dfrac{3\left(3x+5\right)-6-2\left(x+2\right)-6x}{6}\le0\)

\(\Leftrightarrow9x+15-6-2x-4-6x\le0\)

\(\Leftrightarrow x\le-5\)

Mà \(\left\{{}\begin{matrix}x\in Z\\x>-10\end{matrix}\right.\)

Vậy \(x\in\left\{-5;-6;-7;-8;-9\right\}\)

b3\(\Leftrightarrow2x^2+5x-3-3x+1\le x^2+2x-3+x^2-5\\ \Leftrightarrow0.x\le-6\Leftrightarrow x\in\varnothing\)

\(-x^2-2\left(m-1\right)x+2m-1>0\)

\(\Leftrightarrow x^2+2\left(m-1\right)x-2m+1< 0\)

\(f\left(x\right)=x^2+2\left(m-1\right)x-2m+1\)

Yêu cầu bài toán thỏa mãn khi \(f\left(x\right)=0\) có hai nghiệm phân biệt thỏa mãn \(x_1\le0< 1\le x_2\)

\(\Leftrightarrow\left\{{}\begin{matrix}\Delta'=\left(m-1\right)^2+2m-1>0\\f\left(1\right)\le0\\f\left(0\right)\le0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}m^2>0\\1+2\left(m-1\right)-2m+1\le0\\-2m+1\le0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}m\ne0\\m\ge\dfrac{1}{2}\end{matrix}\right.\)

\(\Leftrightarrow m\ge\dfrac{1}{2}\)

ĐKXĐ: \(\left[{}\begin{matrix}x\ge3\\x\le-3\end{matrix}\right.\) ; \(x\ne-5\)

- Với \(x=\pm3\) thỏa mãn

- Với \(x\ne\pm3\)

\(\Leftrightarrow\frac{3x-1}{x+5}\le x\Leftrightarrow x-\frac{3x-1}{x+5}\ge0\)

\(\Leftrightarrow\frac{x^2+2x+1}{x+5}\ge0\Leftrightarrow\frac{\left(x+1\right)^2}{x+5}\ge0\)

\(\Rightarrow x>-5\)

Vậy nghiệm của BPT trên \(\left[-5;5\right]\) là: \(\left[{}\begin{matrix}-5< x\le-3\\3\le x\le5\end{matrix}\right.\)

Tính tổng nghiệm hay tổng nghiệm nguyên?

Tổng nghiệm là \(\sum x=5\)

\(\sqrt{2-f\left(x\right)}=f\left(x\right)\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)\ge0\\f^2\left(x\right)+f\left(x\right)-2=0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)=1\\f\left(x\right)=-2< 0\left(loại\right)\end{matrix}\right.\) 

\(\Rightarrow f\left(1\right)=f\left(2\right)=f\left(3\right)=1\)

\(\sqrt{2g\left(x\right)-1}+\sqrt[3]{3g\left(x\right)-2}=2.g\left(x\right)\)

\(VT=1.\sqrt{2g\left(x\right)-1}+1.1\sqrt[3]{3g\left(x\right)-2}\)

\(VT\le\dfrac{1}{2}\left(1+2g\left(x\right)-1\right)+\dfrac{1}{3}\left(1+1+3g\left(x\right)-2\right)\)

\(\Leftrightarrow VT\le2g\left(x\right)\)

Dấu "=" xảy ra khi và chỉ khi \(g\left(x\right)=1\)

\(\Rightarrow g\left(0\right)=g\left(3\right)=g\left(4\right)=g\left(5\right)=1\)

Để các căn thức xác định \(\Rightarrow\left\{{}\begin{matrix}f\left(x\right)-1\ge0\\g\left(x\right)-1\ge0\end{matrix}\right.\)

Ta có:

\(\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+f\left(x\right).g\left(x\right)-f\left(x\right)-g\left(x\right)+1=0\)

\(\Leftrightarrow\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+\left[f\left(x\right)-1\right]\left[g\left(x\right)-1\right]=0\)

\(\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)=1\\g\left(x\right)=1\end{matrix}\right.\) \(\Leftrightarrow x=3\)

Vậy tập nghiệm của pt đã cho có đúng 1 phần tử