Dat \(x+y=t;xy=v\left(t,v\ne0\right)\)

HPT tro thanh 

\(\hept{\begin{cases}t+\frac{t}{v}=\frac{9}{2}\\v+\frac{1}{v}=\frac{5}{2}\end{cases}}\)

\(\Leftrightarrow\hept{\begin{cases}t+\frac{t}{v}=\frac{9}{2}\left(1\right)\\v^2-\frac{5}{2}v+1=0\left(2\right)\end{cases}}\)

Xet (2):

\(\Delta=\frac{25}{4}-4=\frac{9}{4}\)

Suy ra:

\(v_1=4;v_2=1\)

Voi \(v=4\)thi thay vao HPT thay khong thoa man nen loai 

Voi \(v=1\)thay vao HPT thay khong thoa man nen loai 

Vay HPT vo nghiem 

\(\left\{{}\begin{matrix}\left(x-1\right)\left(y+1\right)=xy-1\\\left(x-2\right)\left(y-2\right)=xy-8\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}xy+x-y-1=xy-1\\xy-2x-2y+4=xy-8\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}x-y=0\\-2x-2y=-12\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x-2y=0\\2x+2y=12\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}4x=12\\x-y=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=x=3\end{matrix}\right.\)

\(\left\{{}\begin{matrix}\left(x-1\right)\left(y+1\right)=xy-1\\\left(x-2\right)\left(y-2\right)=xy-8\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}xy+x-y-1=xy-1\\xy-2x-2y+4=xy-8\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x-y=0\\x+y=6\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=3\end{matrix}\right.\)

Bài 1:

Đặt \(\hept{\begin{cases}S=x+y\\P=xy\end{cases}}\) hpt thành:

\(\hept{\begin{cases}S^2-P=3\\S+P=9\end{cases}}\)\(\Leftrightarrow\hept{\begin{cases}S^2-P=3\\S=9-P\end{cases}}\Leftrightarrow\left(9-P\right)^2-P=3\)

\(\Leftrightarrow\orbr{\begin{cases}P=6\Rightarrow S=3\\P=13\Rightarrow S=-4\end{cases}}\).Thay 2 trường hợp S và P vào ta tìm dc

\(\hept{\begin{cases}x=3\\y=0\end{cases}}\)và\(\hept{\begin{cases}x=0\\y=3\end{cases}}\)

Câu 3: ĐK: \(x\ge0\)

Ta thấy \(x-\sqrt{x-1}=0\Rightarrow x=\sqrt{x-1}\Rightarrow x^2-x+1=0\) (Vô lý), vì thế \(x-\sqrt{x-1}\ne0.\)

Khi đó \(pt\Leftrightarrow\frac{3\left[x^2-\left(x-1\right)\right]}{x+\sqrt{x-1}}=x+\sqrt{x-1}\Rightarrow3\left(x-\sqrt{x-1}\right)=x+\sqrt{x-1}\)

\(\Rightarrow2x-4\sqrt{x-1}=0\)

Đặt \(\sqrt{x-1}=t\Rightarrow x=t^2+1\Rightarrow2\left(t^2+1\right)-4t=0\Rightarrow t=1\Rightarrow x=2\left(tm\right)\)

Câu 3 :

ĐKXĐ : \(x\ge1\)

\(3\left(x^2-x+1\right)=\left(x+\sqrt{x-1}\right)^2\)

\(\Leftrightarrow3\left[x^2-\left(x-1\right)\right]=\left(x+\sqrt{x-1}\right)^2\)

\(\Leftrightarrow3\left(x-\sqrt{x-1}\right)\left(x+\sqrt{x-1}\right)=\left(x+\sqrt{x-1}\right)^2\)

\(\Leftrightarrow\left(x+\sqrt{x-1}\right)\left(x+\sqrt{x-1}-3x+3\sqrt{x-1}\right)=0\)

\(\Leftrightarrow\left(\sqrt{x}+\sqrt{x-1}\right)\left(4\sqrt{x-1}-2x\right)=0\)

Tới đây thì dễ rồi ^^

\(\left\{{}\begin{matrix}\left(x+1\right)\left(y-1\right)=xy-1\\\left(x-3\right)\left(y-3\right)=xy-3\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}xy-x+y-1=xy-1\\xy-3x-3y+9=xy-3\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}-x+y=0\\-3x-3y=-12\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}-x+y=0\\x+y=4\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}2y=4\\x+y=4\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}y=2\\x+2=4\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}y=2\\x=2\end{matrix}\right.\)

Vậy (2;2) là nghiệm

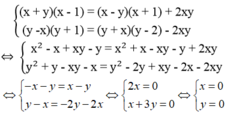
Vậy nghiệm của hệ phương trình là (x; y) = (0; 0)

Ai giúp mình với đi ạ
Mình cảm ơn nhiều.

a) \(\left\{{}\begin{matrix}\dfrac{2x}{x+1}+\dfrac{y}{y+1}=2\\\dfrac{x}{x+1}+\dfrac{3y}{y+1}=-1\end{matrix}\right.\)(Đk: \(x\ne-1;y\ne-1\))

Đặt \(\dfrac{x}{x+1}\)  là A

\(\dfrac{y}{y+1}\) là B 

Ta có HPT mới : \(\left\{{}\begin{matrix}2A+B=2\\A+3B=-1\end{matrix}\right.\)(1)

Giải HPT (1) ta được A=  \(\dfrac{7}{5}\) ; B=\(-\dfrac{4}{5}\)

+Với A=\(\dfrac{7}{5}\) ta có: 

\(\dfrac{x}{x+1}=\dfrac{7}{5}\)

<=>\(5x=7x+7\)

<=>-2x=7

<=> x=\(-\dfrac{7}{2}\)

+Với B = \(-\dfrac{4}{5}\) ta có:

\(\dfrac{y}{y+1}=-\dfrac{4}{5}\)

<=>5y=-4y-4

<=>9y=-4

<=>y=\(-\dfrac{4}{9}\)

Vậy HPT có nghiệm (x;y) = \(\left\{-\dfrac{7}{2};-\dfrac{4}{9}\right\}\)

câu 2 có lẽ dễ nhất luôn :

tách x^2+(1+y)^2=1 thành x^2+1+2y+y^2=1   (1)

tách y^2+(1+x)^2=1 thành y^2+1+2x+x^2=1    (2)

lấy(1) trừ( 2)

==>>>> x=y 

tự làm tiếp nhé