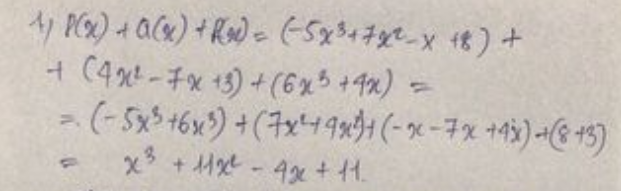Giải bất phương trình : x mũ 2 - 2 sqrt ( x mũ 2 - 7x + 10 ) < 7x - 2

Những câu hỏi liên quan
Giải bất phương trình : x mũ 2 - 2 sqrt( x mũ 2 - 7x + 10) < 7x - 2
\(x^2-2\sqrt{x^2-7x+10}< 7x-2\)
\(ĐK:x\ge5\)
BPT \(\Leftrightarrow x^2-7x+2-2\sqrt{x^2-7x+10}< 0\)
\(\Leftrightarrow t^2-8-2t< 0\left(t=\sqrt{x^2-7x+10}\ge0\right)\)
\(\Leftrightarrow\left(t+2\right)\left(t-4\right)< 0\)
\(\Leftrightarrow-2< t< 4\Leftrightarrow-2< \sqrt{x^2-7x+10}< 4\)
\(\Leftrightarrow\sqrt{x^2-7x+10}< 4\Leftrightarrow x^2-7x-6< 0\)
\(\Leftrightarrow\orbr{\begin{cases}5\le x< \frac{7+\sqrt{73}}{2}\\\frac{7-\sqrt{73}}{2}< x\le2\end{cases}}\)
Chúc bạn học tốt !!!
Đúng 0
Bình luận (0)
giải bất phương trình \(\sqrt{25-x^2}+\sqrt{x^2+7x}>3\)
Giải bất phương trình:
\(\sqrt{3x^2-7x+3}+\sqrt{x^2-3x+4}>\sqrt{x^2-2}+\sqrt{3x^2-5x-1}\)
Chứng minh giá trị của các biểu thức sau không phụ thuộc vào biến x
a. A=-3x(x-5)+3(x mũ 2 -4x)-3x+10
b. B=4x(x mũ 2 -7x+2)-4(x mũ 3-7x mũ 2+2x-5)
P(x)+Q(x)+R(x)=(-5x mũ 3 + 7x mũ 2 -x + 8) +(4x mũ 2 -7x+3)
Phân tích đa thức thành nhân tử bằng phương pháp dùng hệ số bất định với các hê số nguyên x mũ 4 - 5xmũ 3 + 7x mũ 2 - 6
Đặt H \(=x^4-5x^3+7x^2-6\)
Gỉa sử : \(H=\left(x^2+ax+b\right)\left(x^2+cx+d\right)\)
\(=x^4+cx^3+dx^2+ax^{3\:}+acx^2+adx+bx^2+bcx+bd\)
\(=x^4+\left(a+c\right)x^3+\left(ac+b+d\right)x^2+\left(ad+bc\right)x+bd\)
\(\Leftrightarrow\hept{\begin{cases}a+c=-5\\ac+b+d=7\\ad+bc=0\end{cases}}\)
\(\left\{bd=6\right\}\)
\(\Leftrightarrow\hept{\begin{cases}a=-3\\b=3\\c=-2\end{cases}}\)
\(\left\{d=-2\right\}\)
\(\Rightarrow H=\left(x^2-3x+3\right)\left(x^2-2x-2\right)\)
Chúc bạn học tốt !!!
Đúng 1
Bình luận (0)
cho bất phương trình \(\sqrt{7x+7}+\sqrt{7x-6}+2\sqrt{49x^2+7x-42}< 181-14x\)
với t \(=\sqrt{7x+7}+\sqrt{7x-6}\) (t \(\ge\)0 ), bất phương trình sẽ trở thành ?
\(\sqrt{7x+7}+\sqrt{7x-6}=t\ge0\)
\(bpt\Leftrightarrow t+t^2< 182\Leftrightarrow-14< t< 13\Leftrightarrow t< 13\Leftrightarrow\sqrt{7x+7}+\sqrt{7x-6}< 13\left(đk:x\ge\dfrac{6}{7}\right)\Leftrightarrow14x+1+2\sqrt{\left(7x+7\right)\left(7x-6\right)}< 169\Leftrightarrow2\sqrt{\left(7x+7\right)\left(7x-6\right)}< 168-14x\Leftrightarrow\left\{{}\begin{matrix}\left(7x+7\right)\left(7x-6\right)\ge0\\168-14x\ge0\\4\left(7x+7\right)\left(7x-6\right)< \left(168-14x\right)^2\end{matrix}\right.\)
\(giảibpt\Rightarrowđáp\) \(số\)
Đúng 0
Bình luận (0)
Giải các phương trình sau: \(\left(\sqrt{x+5}-\sqrt{x+2}\right).\left(4+\sqrt{x^2+7x+10}\right)=6\)
2x-10 phần x mũ 2 - 7x + 10 - 2x phần x mũ 2 - 4 + 1 phần 2-x