Tính a,b
\(\left\{{}\begin{matrix}2a+b=82\\15a-13b=0\end{matrix}\right.\)
Những câu hỏi liên quan
Tính
\(\left\{{}\begin{matrix}2a+b=82\\15a-13b=0\end{matrix}\right.\)
Chú ý: chỉ cần cách giải, phép tính, cách làm, không cần đáp số
Ta có 15a - 13b = 0
\(\Rightarrow\) \(\frac{a}{\frac{1}{15}}=\frac{b}{\frac{1}{13}}\)
Áp dụng tính chất dãy tỉ số bằng nhau
\(\Rightarrow\) \(\frac{2a}{\frac{2}{15}}=\frac{b}{\frac{1}{13}}=\frac{2a+b}{\frac{2}{15}+\frac{1}{13}}=\frac{82}{\frac{41}{195}}=390\)
suy ra \(\left\{{}\begin{matrix}15a=390\Leftrightarrow a=26\\13b=390\Leftrightarrow b=30\end{matrix}\right.\)
Vậy a = 26, b = 30
Đúng 0
Bình luận (0)
1.\(\left\{{}\begin{matrix}a,b>0\\2a+3b=4\end{matrix}\right.\)Tìm GTNN của
M=\(\dfrac{2002}{a}+\dfrac{2017}{b}+2096a-5501b\)
Bài này mình làm lâu rồi á bann sửa dấu `2a+3b<=4` thành `2a+3b=4` nhé!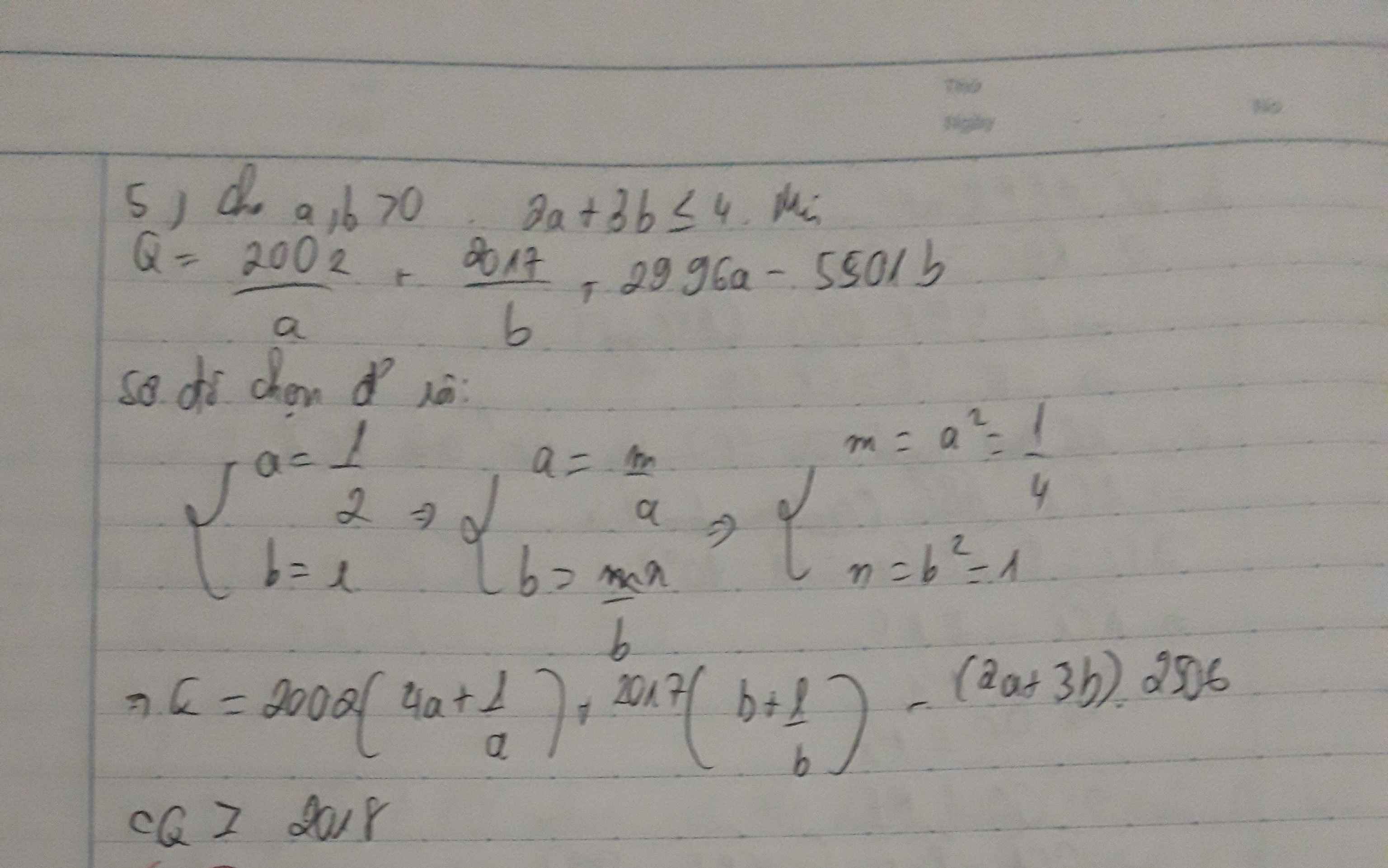
Đúng 0
Bình luận (1)
1.) liệt kê các tập hợp sau :
a.) A left{{}begin{matrix}end{matrix}right.xin N|}2le xle10left{right}
b.) B left{{}begin{matrix}end{matrix}right.xin Z|9le x^2le36left{right}}
c.) C left{{}begin{matrix}end{matrix}right.nin N}^{cdot}|3le n^2le30left{right}
B.) B là tập hợp các số thực x thỏa x2 - 4x +2 0
d.) D left{{}begin{matrix}end{matrix}right.frac{1}{n+1}}|nin N;nle4left{right}
e.) E left{{}begin{matrix}end{matrix}right.2n^2-1|nin N^{cdot}},nle7left{right}
2.) chỉ ra tính chất đặc t...
Đọc tiếp
1.) liệt kê các tập hợp sau :
a.) A = \(\left\{{}\begin{matrix}\\\end{matrix}\right.x\in N|}2\le x\le10\left\{\right\}\)
b.) B =\(\left\{{}\begin{matrix}\\\end{matrix}\right.x\in Z|9\le x^2\le36\left\{\right\}}\)
c.) C = \(\left\{{}\begin{matrix}\\\end{matrix}\right.n\in N}^{\cdot}|3\le n^2\le30\left\{\right\}\)
B.) B là tập hợp các số thực x thỏa x2 - 4x +2 = 0
d.) D = \(\left\{{}\begin{matrix}\\\end{matrix}\right.\frac{1}{n+1}}|n\in N;n\le4\left\{\right\}\)
e.) E = \(\left\{{}\begin{matrix}\\\end{matrix}\right.2n^2-1|n\in N^{\cdot}},n\le7\left\{\right\}\)
2.) chỉ ra tính chất đặc trưng :
a.) A = \(\left\{{}\begin{matrix}\\\end{matrix}\right.0;1;2;3;4\left\{\right\}}\)
b.) B = \(\left\{{}\begin{matrix}\\\end{matrix}\right.0;4;8;12;16\left\{\right\}}\)
c.) C = \(\left\{{}\begin{matrix}\\\end{matrix}\right.0;4;9;16;25;36\left\{\right\}}\)
3.) Trong các tập hợp sau , tập hợp nào là con tập nào :
a.) A = \(\left\{{}\begin{matrix}\\\end{matrix}\right.1;2;3\left\{\right\}}\)
B = \(\left\{{}\begin{matrix}\\\end{matrix}\right.x\in N^{\cdot}|n\le4\left\{\right\}}\)
b.) A = \(\left\{{}\begin{matrix}\\\end{matrix}\right.n\in N^{\cdot}}|n\le5\left\{\right\}\)
B = \(\left\{{}\begin{matrix}\\\end{matrix}\right.n\in Z|0\le|n|\le5\left\{\right\}}\)
ycbt\(\Leftrightarrow\left\{{}\begin{matrix}a< 0\\\Delta^{phay}\le0\end{matrix}\right.hay\left\{{}\begin{matrix}-1< 0\\a^2-\left[\left(-1\right)\left(-a+2a-6\right)\right]\le0\end{matrix}\right.\)
\(\Leftrightarrow a^2+a-6\le0\Leftrightarrow-3\le a\le2\)\(\Rightarrow a\in\left[-3;2\right]\)
giai hpt
a.\(\left\{{}\begin{matrix}x=y+4\\2x+3=0\end{matrix}\right.\)
b.\(\left\{{}\begin{matrix}2x+y=7\\3y-x=7\end{matrix}\right.\)
c.\(\left\{{}\begin{matrix}5x+y=3\\-x-\dfrac{1}{5}y=\dfrac{-3}{5}\end{matrix}\right.\)
d.\(\left\{{}\begin{matrix}3x-5y=-18\\x-5=2y\end{matrix}\right.\)
\(a) \begin{cases}x=y+4\\2x+3=0\end{cases}\Leftrightarrow\begin{cases}x = y + 4\\2x = -3\end{cases}\Leftrightarrow\begin{cases}\dfrac{-3}{2} = y + 4\\x = \dfrac{-3}{2}\end{cases}\Leftrightarrow\begin{cases}y = \dfrac{-11}{2}\\x = \dfrac{-3}{2}\end{cases}\\b) \begin{cases}2x + y = 7\\3y - x = 7\end{cases}\Leftrightarrow\begin{cases}2x + y = 7\\6y - 2x = 14\end{cases}\Leftrightarrow\begin{cases}2x + y = 7\\7y = 21\end{cases}\Leftrightarrow\begin{cases}2x + 3 = 7\\y = 3\end{cases}\Leftrightarrow\begin{cases}x=2\\y=3\end{cases}\\ c) \begin{cases} 5x + y = 3 \\ -x - \dfrac{1}{5}y=\dfrac{-3}{5} \end{cases} \Leftrightarrow \begin{cases} 5x + y = 3 \\ 5x + y = 3 \end{cases} (luôn\ đúng) \Leftrightarrow Phương\ trình\ vô\ số\ nghiệm \\d) \begin{cases} 3x - 5y = -18 \\ x - 5 = 2y \end{cases} \Leftrightarrow \begin{cases} 3x - 5y = -18 \\ 3x - 6y = 15 \end{cases} \Leftrightarrow \begin{cases} x - 5 = 2.(-33)\\ y = -13 \end{cases} \Leftrightarrow \begin{cases}x = -61\\y=-33 \end{cases} \)
Đúng 1
Bình luận (0)
Bài 1
(I)left{{}begin{matrix}x-y02x+y3end{matrix}right. ; (II) left{{}begin{matrix}2x-3y-42x-3y5end{matrix}right.; (III) left{{}begin{matrix}x+2y3-x-2y-3end{matrix}right.
Bài 2
a)left{{}begin{matrix}2x+y1x-y2end{matrix}right.; b)left{{}begin{matrix}x+2y2x+2y5end{matrix}right.; c)left{{}begin{matrix}2x+y3-2x-y-3end{matrix}right.
Đọc tiếp
Bài 1
(I)\(\left\{{}\begin{matrix}x-y=0\\2x+y=3\end{matrix}\right.\) ; (II) \(\left\{{}\begin{matrix}2x-3y=-4\\2x-3y=5\end{matrix}\right.\); (III) \(\left\{{}\begin{matrix}x+2y=3\\-x-2y=-3\end{matrix}\right.\)
Bài 2
a)\(\left\{{}\begin{matrix}2x+y=1\\x-y=2\end{matrix}\right.\); b)\(\left\{{}\begin{matrix}x+2y=2\\x+2y=5\end{matrix}\right.\); c)\(\left\{{}\begin{matrix}2x+y=3\\-2x-y=-3\end{matrix}\right.\)
Bài 2:
a: 2x+y=1 và x-y=2
=>3x=3 và x-y=2
=>x=1 và y=-1
b: x+2y=2 và x+2y=5
=>0x=-3 và x+2y=2
=>\(\left(x,y\right)\in\varnothing\)
c: 2x+y=3 và -2x-y=-3
=>0x=0 và 2x+y=3
=>\(\left\{{}\begin{matrix}x\in R\\y=3-2x\end{matrix}\right.\)
Đúng 0
Bình luận (0)
giải hpt:
a) \(\left\{{}\begin{matrix}4x+9y=6\\3x^2+6xy-x+3y=0\end{matrix}\right.\)
b) \(\left\{{}\begin{matrix}\left(x+y+2\right)\left(2x+2y-1\right)=0\\3x^2-32y^2+5=0\end{matrix}\right.\)
c) \(\left\{{}\begin{matrix}2x^2-xy+3y^2=7x+12y-1\\x-y+1=0\end{matrix}\right.\)
\(\left\{{}\begin{matrix}a^3+b^3+ab=2a+4\\ab+a+2b=1\end{matrix}\right.\)
Tìm m để hệ bất phương trình có nghiệma) left{{}begin{matrix}2x-10x-m 2end{matrix}right.b) left{{}begin{matrix}3left(x-6right) -3dfrac{5x+m}{2}7end{matrix}right.c) left{{}begin{matrix}x^2-1le0x-m0end{matrix}right.d) left{{}begin{matrix}x-2ge0left(m^2+1right)x 4end{matrix}right.e) left{{}begin{matrix}mleft(mx-1right) 2mleft(mx-2right)ge2m+1end{matrix}right.
Đọc tiếp
Tìm m để hệ bất phương trình có nghiệm
a) \(\left\{{}\begin{matrix}2x-1>0\\x-m< 2\end{matrix}\right.\)
b) \(\left\{{}\begin{matrix}3\left(x-6\right)< -3\\\dfrac{5x+m}{2}>7\end{matrix}\right.\)
c) \(\left\{{}\begin{matrix}x^2-1\le0\\x-m>0\end{matrix}\right.\)
d) \(\left\{{}\begin{matrix}x-2\ge0\\\left(m^2+1\right)x< 4\end{matrix}\right.\)
e) \(\left\{{}\begin{matrix}m\left(mx-1\right)< 2\\m\left(mx-2\right)\ge2m+1\end{matrix}\right.\)
a, hệ\(\Leftrightarrow\)$\left \{ {{x>\frac{1}{2} } \atop {x<m+2}} \right.$
để hệ có nghiệm ⇒ m+2< $\frac{1}{2}$ ⇒ m<$\frac{-3}{2}$
Đúng 1
Bình luận (0)