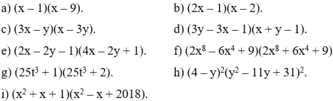BPT\(\Leftrightarrow\left(2017x^2+2018\right)\left(2x-1\right)-\left(2017x^2+2018\right)\left(4-5x\right)\ge0\)

\(\Leftrightarrow\left(2017x^2+2018\right)\left(2x-1-4+5x\right)\ge0\)

\(\Leftrightarrow\left(2017x^2+2018\right)\left(7x-5\right)\ge0\)

DO 2017x²+2018 luôn luôn lớn hơn 0

ĐỂ B PT \(\ge\)0\(\Leftrightarrow7x-5\ge0\)

                              \(\Leftrightarrow x\ge\frac{5}{7}\)

vậy ...........

Ta có : x - 1 = 2018 - 1 = 2017 

N = x⁶ - 2017x⁵ - 2017x⁴ - 2017x³ - 2017x² - 2017x - 2017

N = x⁶ - ( x - 1 ).x⁵ - ( x - 1 ).x⁴ - ( x - 1 ).x³ - ( x - 1 ).x² - ( x - 1 ).x - ( x - 1 )

N = x⁶ - x⁶ + x⁵ - x⁵ + x⁴ - x⁴ + x³ - x³ + x² - x² + x - x + 1

N = 1

x^4+2018x^2+2017x+2018

=(x^4-x)+(2018x^2+2018x+2018)

=x(x^3-1)+2018(x^2+x+1)

=x(x-1)(x^2+x+1)+2018(x^2+x+1)

=(x^2+x+1)(x^2-x+2018)

f(2016)=2016⁸ - 2017*2016⁷ +2017*2016⁶ - 2017*2016⁵ +...+2017*2016² - 2017*2016+ 2018

         =2016⁸ -( 2016⁸ + 2016) + (2016⁷+2016) - (2016⁶ + 2016)+....+2016³+2016 -( 2016² + 2016)+2018

         =2018

Thay x=2016 thì 2017=x+1 và 2018=x+2 Do đó

\(f\left(x\right)=x^8-\left(x+1\right)x^7+\left(x+1\right)x^6-...-\left(x+1\right)x\)\(+x+2\)

           \(=x^8-x^8-x^7+x^7+x^6-...+x^2-x^2-x+x+2\)

            \(=2\)

i do not know

x=2018 nên x-1=2017

\(A=x^{10}-x^9\left(x-1\right)-x^8\left(x-1\right)-...-x^2\left(x-1\right)-x\left(x-1\right)-1\)

\(=x^{10}-x^{10}+x^9-x^9+x^8-...-x^3+x^2-x^2+x-1\)

=x-1=2017

\(A=x^3+2x^2+3x\\ =x\left(x^2+2x+1\right)\\ =x\left(x+1\right)^2\\ =1999.\left(1999+1\right)=1999.2000\\ =3998000\)

\(B=x^4-2017x^3+2017x^2-2017x+2018\\ =x^4-2016x^3-x^3+2016x^2+x^2-2016x-x+2016+2\\ =x^3\left(x-2016\right)-x^3\left(x-2016\right)+x\left(x-2016\right)-\left(x-2016\right)+2\\ =\left(x-2016\right)\left(x^3+x-1\right)+2=0+2=0\)

Bạn xem lại đề câu a nhé , theo mk thì phải là 2 thì tính ms nhanh đc, 3 thì cũng giải đc nhưng ko hợp lí lắm

\(x^{2018}+2x^{2017}+3x^{2016}+...+2017x+2018\)

\(=1+2+3+...+2017+2018\)

\(=\frac{2018.\left(2018+1\right)}{2}=2037171\)