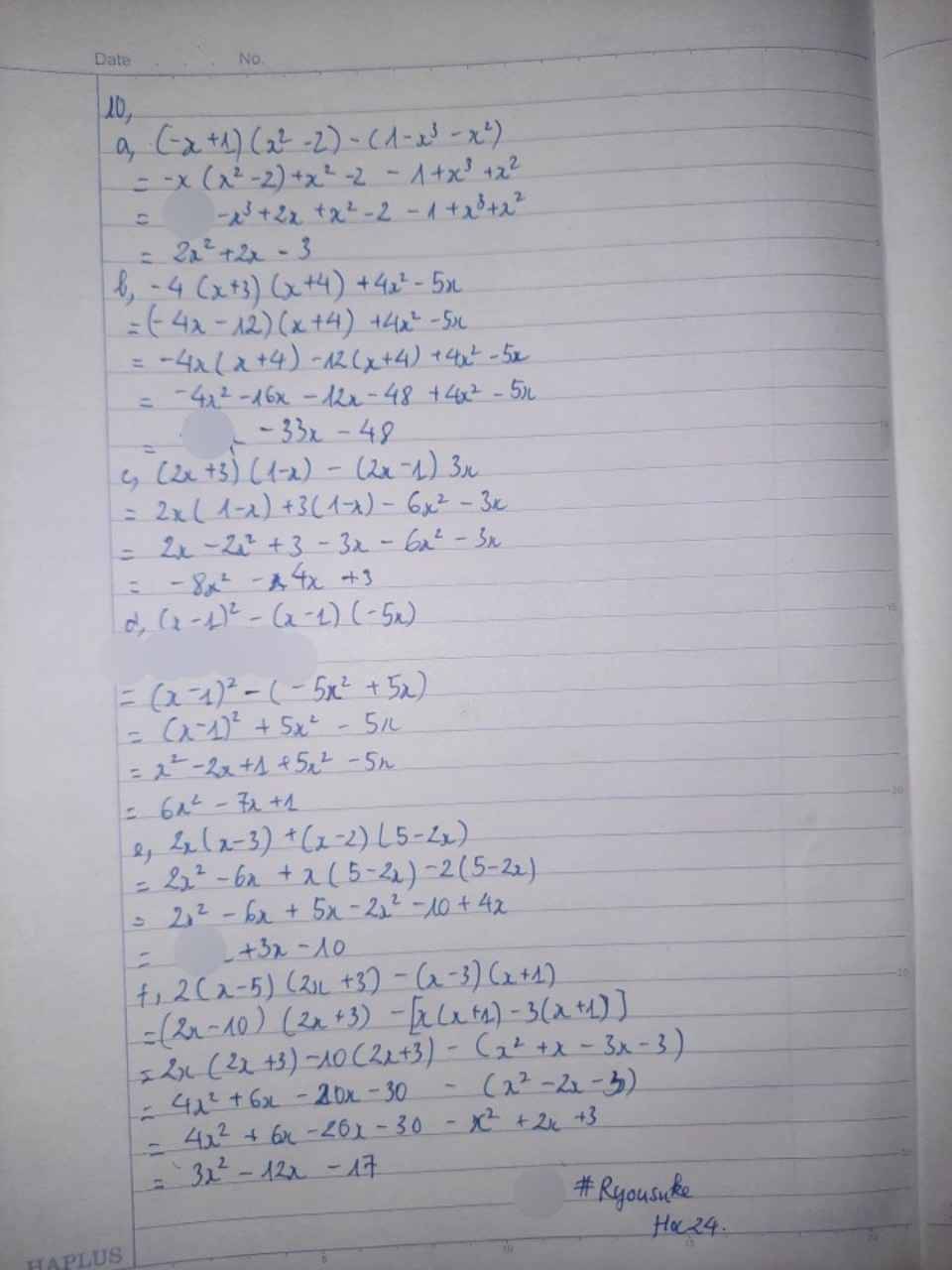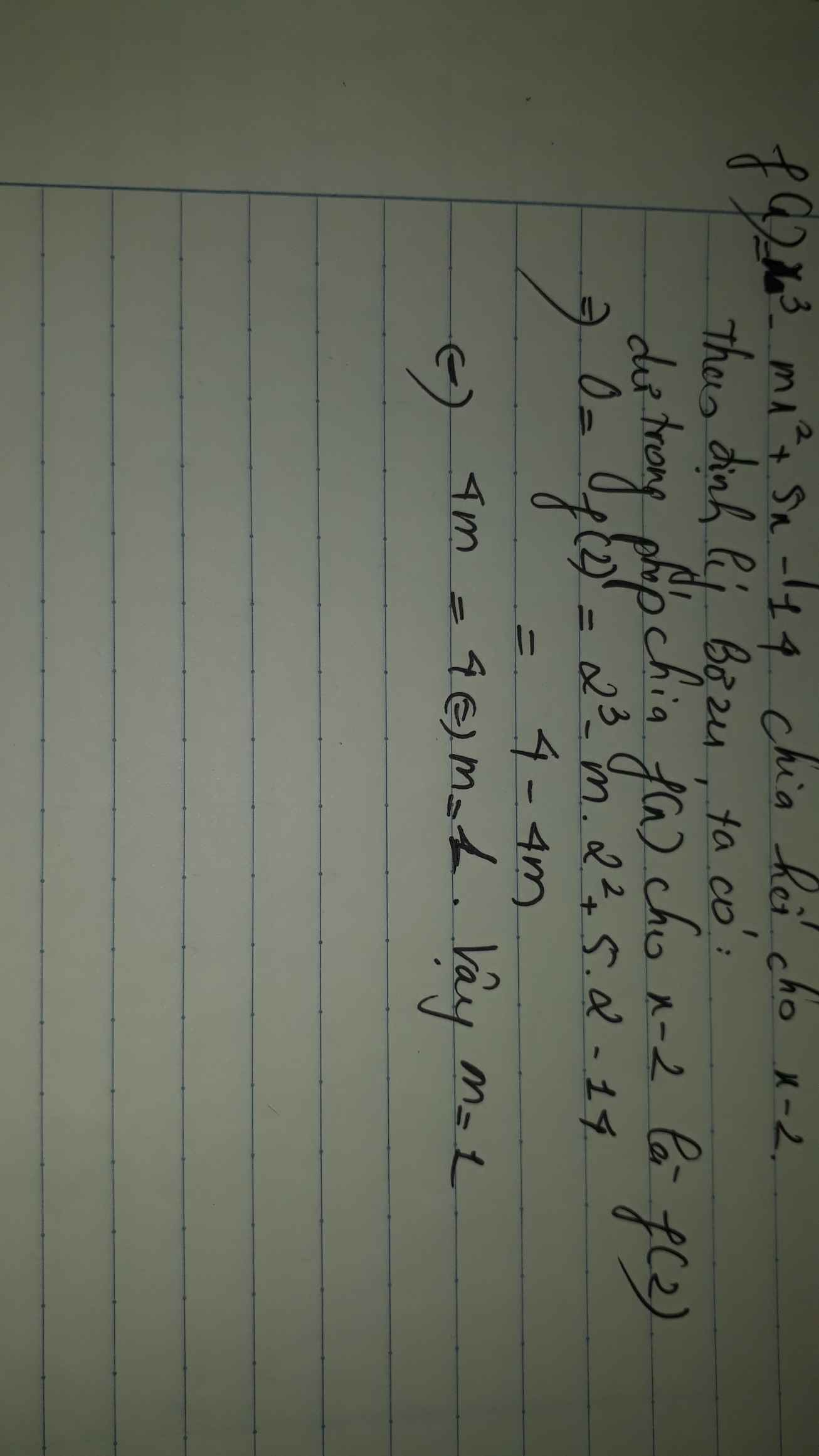Giúp mình với ạ. Cảm ơn ạ
Cho P(x)= x3 - 2x + x3 -x +1 ; Q(x) = 2x2 -8 -4x + 2x3 +x -7
a) Thu gọn mỗi đa thức trên rồi sắp xếp chúng theo lũy thừa giảm dần của biến
b) Tính P(x) + Q(x); c) Tính A(x) = P(x) - Q(x) d) Tính B(x) = Q(x) - P(x)
e) Tìm nghiệm của A(x)? f) Tìm nghiệm của B (x)