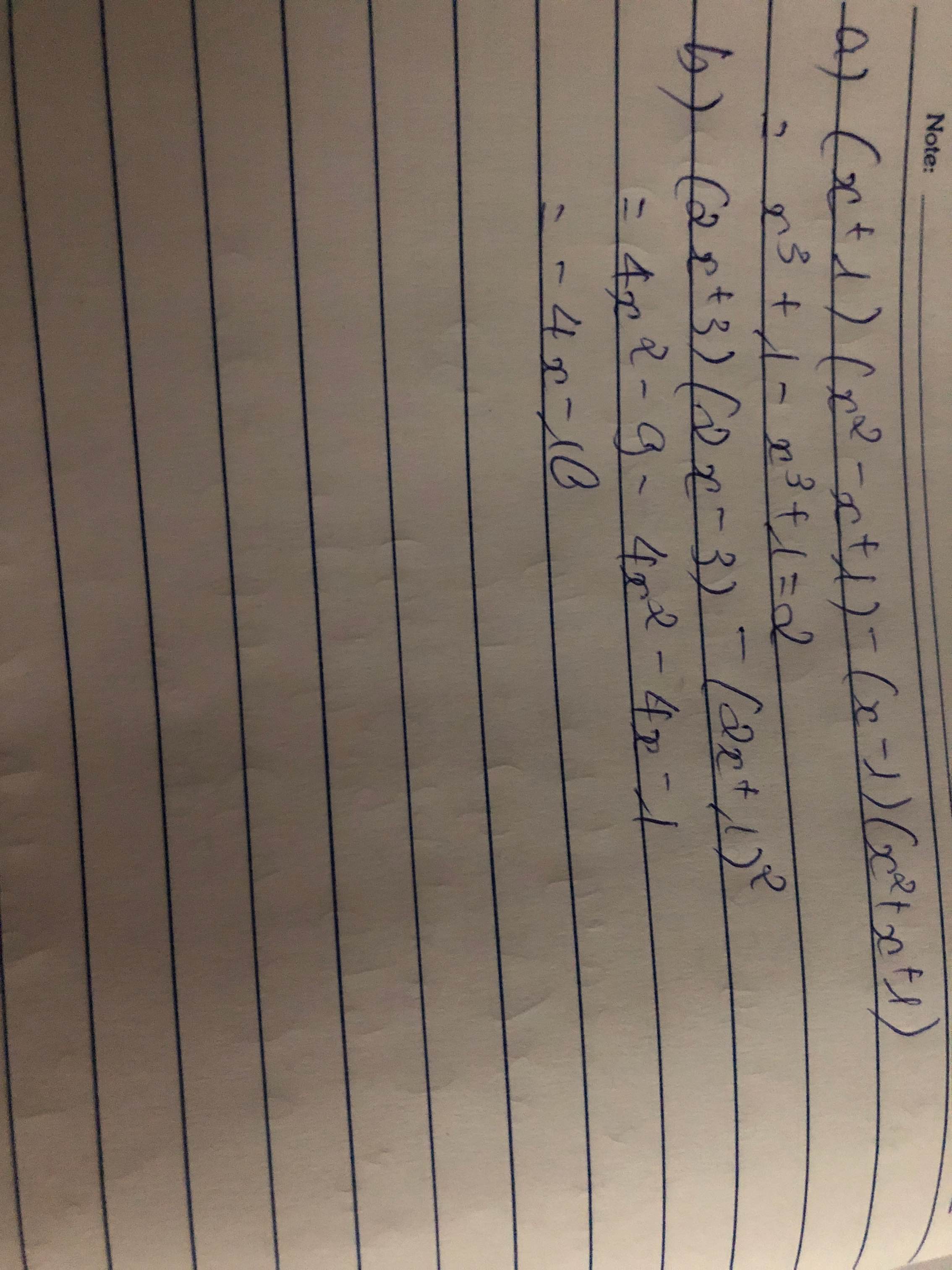a) Ta có: \(\left(x+1\right)\left(x^2-x+1\right)-\left(x-1\right)\left(x^2+x+1\right)\)

\(=x^3+1-x^3+1\)

=2

b) Ta có: \(\left(2x+3\right)\left(2x-3\right)-\left(2x+1\right)^2\)

\(=4x^2-9-4x^2-4x-1\)

\(=-4x-10\)

Ta có: \(2x\left(3x-1\right)-\left(2x+1\right)\left(x-3\right)\)

\(=6x^2-2x-\left(2x^2-6x+x-3\right)\)

\(=6x^2-2x-2x^2+5x+3\)

\(=4x^2+3x+3\)

Ta có: \(3\left(x^2-2x\right)-\left(4x+2\right)\left(x-1\right)\)

\(=3x^2-6x-\left(4x^2-4x+2x-2\right)\)

\(=3x^2-6x-4x^2+2x+2\)

\(=-x^2-4x+2\)

\(2x\left(3x-1\right)-\left(2x+1\right)\left(x-3\right)=6x^2-2x-2x^2+5x+3=4x^2+3x+3\)

\(3\left(x^2-2x\right)-\left(4x+2\right)\left(x-1\right)=3x^2-6x-4x^2+2x-2=-x^2-4x-2\)

cậu xem câu hỏi của Quỳnh Phạm nha

1) `2x(3x-1)-(2x+1)(x-3)`

`=6x^2-2x-2x^2+6x-x+3`

`=4x^2+3x+3`

2) `3(x^2-3x)-(4x+2)(x-1)`

`=3x^2-9x-4x^2+4x-2x+2`

`=-x^2-7x+2`

3) `3x(x-5)-(x-2)^2-(2x+3)(2x-3)`

`=3x^2-15x-(x^2-4x+4)-(4x^2-9)`

`=3x^2-15x-x^2+4x-4-4x^2+9`

`=-2x^2-11x+5`

4) `(2x-3)^2+(2x-1)(x+4)`

`=4x^2-12x+9+2x^2+8x-x-4`

`=6x^2-5x+5`

ĐKXĐ: \(x\notin\left\{1;\dfrac{1}{2}\right\}\)

\(\left(\dfrac{1}{x-1}+2+\dfrac{2x^3+x^2-x}{1-x^3}\right):\dfrac{1-2x}{x^3+x-2}\)

\(=\left(\dfrac{1}{x-1}+2-\dfrac{2x^3+x^2-x}{\left(x-1\right)\left(x^2+x+1\right)}\right)\cdot\dfrac{x^3+x-2}{1-2x}\)

\(=\dfrac{x^2+x+1+2\left(x^3-1\right)-2x^3-x^2+x}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{x^3-x^2+x^2-x+2x-2}{-\left(2x-1\right)}\)

\(=\dfrac{2x+1+2x^3-2-2x^3}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{\left(x-1\right)\left(x^2+x+2\right)}{-\left(2x-1\right)}\)

\(=\dfrac{2x-1}{x^2+x+1}\cdot\dfrac{-\left(x^2+x+2\right)}{2x-1}=\dfrac{-x^2-x-2}{x^2+x+1}\)

\(a,=x^2-6x+9-x^2+6x=9\\ b,=4x^2+4x+1-4x^2+9-4x-8=2\\ c,=\left(2x^2-2x-x+1\right):\left(x-1\right)\\ =\left(x-1\right)\left(2x-1\right):\left(x-1\right)=2x-1\)

`a)(x-3)^2-x(x-6)`

`=x^2-6x+9-x^2+6x=9`

`b)(2x+1)^2-(3+2x)(2x-3)-4(x+2)`

`=4x^2+4x+1-(4x^2-9)-4x-8`

`=2`

`c)(2x^2-3x+1):(x-1)`

`=(2x^2-2x-x+1):(x-1)`

`=[2x(x-1)-(x-1)]:(x-1)`

`=2x-1`

a) \(\left(x-3\right)^2-x\left(x-6\right)=x^2-6x+9-x^2+6x=9\)

b) \(\left(2x+1\right)^2-\left(3+2x\right)\left(2x-3\right)-4\left(x+2\right)=4x^2+4x+1-4x^2+9-4x-8=2\)

c) \(\left(2x^2-3x+1\right):\left(x-1\right)=\left[2x\left(x-1\right)-\left(x-1\right)\right]:\left(x-1\right)=\left[\left(x-1\right)\left(2x-1\right)\right]:\left(x-1\right)=2x-1\)

Answer:

\(\left(2x+1\right)^2+\left(2x-1\right)^2-2\left(1+2x\right)\left(2x-1\right)\)

\(=(4x^2+4x+1)+(4x^2-4x+1)-2(4x^2-1)\)

\(=4x^2+4x+1+4x^2-4x+1-8x^2+2\)

\(=(4x^2+4x^2-8x^2)+(4x-4x)+(1+1+2)\)

\(=4\)

\((x-1)^3-(x+2)(x^2-2x+4)+3(x-1)(x+1)\)

\(=(x^3-3x^2+3x-1)-(x^3+8)+3(x^2-1)\)

\(=x^3-3x^2+3x-1-x^3-8+3x^2-3\)

\(=(x^3-x^3)+(-3x^2+3x^2)+3x+(-1-8-3)\)

\(=3x-12\)

a: Ta có: \(\left(x+5\right)^2-4x\left(2x+3\right)^2-\left(2x-1\right)\left(x+3\right)\left(x-3\right)\)

\(=x^2+10x+25-4x\left(4x^2+12x+9\right)-\left(2x-1\right)\left(x^2-9\right)\)

\(=x^2+10x+25-16x^3-48x^2-36x-2x^3+18x+x^2-9\)

\(=-18x^3-46x^2-8x+16\)

\(a,=x^2-4-x^2+2x+3=2x-1\\ b,=x^3+3x^2-5x-15+x^2-x^3+4x-4x^2=-x-15\\ c,=2x^2+3x-10x-15-2x^2+6x+x+7=-8\\ d,=\left(2x+1+3x-1\right)^2=25x^2\)

a: \(=\dfrac{\left(x+1\right)\left[\left(3x-2\right)-\left(2x+5\right)\left(x-1\right)\right]}{x+1}\)

=3x-2-2x^2+2x-5x+5

=-2x^2+3

b: \(=\left(2x+1-3+x\right)^2=\left(3x-2\right)^2=9x^2-12x+4\)

c: =x^3-3x^2+3x-1-x^3-1+9x^2-1

=6x^2+3x-3

\(a,\left[\left(3x-2\right)\left(x+1\right)-\left(2x+5\right)\left(x^2-1\right)\right]:\left(x+1\right)\)

\(=\left[\left(3x-2\right)\left(x+1\right)-\left(2x+5\right)\left(x-1\right)\left(x+1\right)\right]:\left(x+1\right)\)

\(=\left[\left(x+1\right)\left(3x-2-\left(2x+5\left(x-1\right)\right)\right)\right]:\left(x+1\right)\)

\(=\left[\left(x+1\right)\left(3x-2-2x^2+2x-5x+5\right)\right]:\left(x+1\right)\)
\(=\left[\left(x+1\right)\left(-2x^2+3\right)\right].\dfrac{1}{x+1}\)

\(=-2x^2+3\)

\(b,\left(2x+1\right)^2-2\left(2x+1\right)\left(3-x\right)\)

\(=\left(2x+1\right)\left[\left(2x+1\right)-2\left(3-x\right)\right]\)

\(=\left(2x+1\right)\left(2x+1-6+2x\right)\)

\(=\left(2x+1\right)\left(4x-5\right)\)

\(c,\left(x-1\right)^3-\left(x+1\right)\left(x^2-x+1\right)-\left(3x+1\right)\left(1-3x\right)\)

\(=x^3-3x^2+3x-1-x^3-1-\left(3x-9x^2+1-3x\right)\)

\(=-3x^2+3x-2-3x+9x^2-1+3x\)

\(=6x^2+3x-3\)