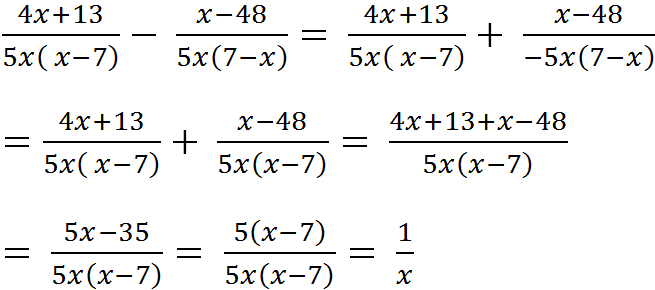5. a) \(\dfrac{4x+13}{5x\left(x-7\right)}-\dfrac{x-48}{5x\left(7-x\right)};\) b) \(\dfrac{1}{x-5x^2}-\dfrac{25x-15}{25x^2-1}\)

Những câu hỏi liên quan
Dùng quy tắc đổi dấu rồi thực hiện các phép tính :
a) \(\dfrac{4x+13}{5x\left(x-7\right)}-\dfrac{x-48}{5x\left(7-x\right)}\)
b) \(\dfrac{1}{x-5x^2}-\dfrac{25x-15}{25x^2-1}\)
Giải phương trình
a) \(\dfrac{3}{5x-1}\)+ \(\dfrac{2}{3-5x}\)=\(\dfrac{4}{\left(1-5x\right)\left(x-3\right)}\)
b) \(\dfrac{5-x}{4x^2-8x}\)+\(\dfrac{7}{8x}\)=\(\dfrac{x-1}{2x\left(x-2\right)}\)+\(\dfrac{1}{8x-16}\)
a:Sửa đề: \(\dfrac{3}{5x-1}+\dfrac{2}{3-x}=\dfrac{4}{\left(1-5x\right)\left(x-3\right)}\)
=>3x-9-10x+2=-4
=>-7x-7=-4
=>-7x=3
=>x=-3/7
b: =>\(\dfrac{5-x}{4x\left(x-2\right)}+\dfrac{7}{8x}=\dfrac{x-1}{2x\left(x-2\right)}+\dfrac{1}{8\left(x-2\right)}\)
=>\(2\left(5-x\right)+7\left(x-2\right)=4\left(x-1\right)+x\)
=>10-2x+7x-14=4x-4+x
=>5x-4=5x-4
=>0x=0(luôn đúng)
Vậy: S=R\{0;2}
Đúng 1
Bình luận (0)
Giải phương trình sau :
a,\(\dfrac{7-3x}{12}+\dfrac{5x+2}{7}=x+13\)
b,\(\dfrac{3\left(x+3\right)}{4}-\dfrac{1}{2}=\dfrac{5x+9}{7}-\dfrac{7x-9}{4}\)
c,\(\dfrac{2x+1}{3}-\dfrac{5x+2}{7}=x+3\)
d,\(\dfrac{2x-3}{3}-\dfrac{2x+3}{7}=\dfrac{4x+3}{5}-17\)
a: \(\Leftrightarrow7\left(7-3x\right)+12\left(5x+2\right)=84\left(x+13\right)\)
\(\Leftrightarrow49-21x+60x+24=84x+1092\)
\(\Leftrightarrow39x-84x=1092-73\)
=>-45x=1019
hay x=-1019/45
b: \(\Leftrightarrow21\left(x+3\right)-14=4\left(5x+9\right)-7\left(7x-9\right)\)
=>21x+63-14=20x+36-49x+63
=>21x+49=-29x+99
=>50x=50
hay x=1
c: \(\Leftrightarrow7\left(2x+1\right)-3\left(5x+2\right)=21x+63\)
=>14x+7-15x-6-21x-63=0
=>-22x-64=0
hay x=-32/11
d: \(\Leftrightarrow35\left(2x-3\right)-15\left(2x+3\right)=21\left(4x+3\right)-17\cdot105\)
=>70x-105-30x-45=84x+63-1785
=>40x-150-84x+1722=0
=>-44x+1572=0
hay x=393/11
Đúng 0
Bình luận (0)
a, msc 12.7=84
Chuyển vế về =0 rồi làm
b,msc 28
c,làm tương tự
Đúng 0
Bình luận (0)
a, \(\Rightarrow49-21x+60x+24=84x+1092\)
\(\Leftrightarrow-45x=1019\Leftrightarrow x=-\dfrac{1019}{45}\)
b, \(\Rightarrow21\left(x+3\right)-14=4\left(5x+9\right)-7\left(7x-9\right)\)
\(\Leftrightarrow21x+63-14=20x+36-49x+63\)
\(\Leftrightarrow50x=50\Leftrightarrow x=1\)
c, \(\Rightarrow14x+7-15x-6=21x+63\Leftrightarrow-22x=62\Leftrightarrow x=-\dfrac{31}{11}\)
d, \(\Rightarrow35\left(2x-3\right)-15\left(2x+3\right)=21\left(4x+3\right)-105.17\)
\(\Leftrightarrow70x-105-30x-45=84x+63-1785\)
\(\Leftrightarrow-44x=-1572\Leftrightarrow x=\dfrac{393}{11}\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Tìm x,y biết
a.\(\left(x-7\right)^{x+1}-\left(x-7\right)^{x+1}=0\)
b.\(\dfrac{5x-1}{3}=\dfrac{7y-6}{5}=\dfrac{5x+7y-7}{4x}\)
c.\(\left|x+5\right|+\left(3y-4\right)^{2017}=0\)
Giúp mk nha
Giải các phương trình
1/ dfrac{1}{x^2+3x+2}+dfrac{1}{x^2+5x+6}+dfrac{1}{x^2+7x+12}dfrac{1}{6}
2/ dfrac{1}{x^2-6x+8}+dfrac{1}{x^2-10x+24}+dfrac{1}{x^2-14x+48}dfrac{1}{9}
3/ dfrac{1}{x^2-3x+3}+dfrac{2}{x^2-3x+4}dfrac{6}{x^2-3x+5}
4/ dfrac{6}{left(x+1right)left(x+2right)}+dfrac{8}{left(x-1right)left(x+4right)}1
5/ 4left(x^3+dfrac{1}{x^3}right)13left(x+dfrac{1}{x}right)
6/ dfrac{4x}{4x^2-8x+7}+dfrac{3x}{4x^2-10x+7}1
7/ dfrac{x^2-3x+5}{x^2-4x+5}-dfrac{x^2-5x+5}{x^2-6x+5}-dfrac{1}{...
Đọc tiếp
Giúp mk nha
Giải các phương trình
1/ \(\dfrac{1}{x^2+3x+2}+\dfrac{1}{x^2+5x+6}+\dfrac{1}{x^2+7x+12}=\dfrac{1}{6}\)
2/ \(\dfrac{1}{x^2-6x+8}+\dfrac{1}{x^2-10x+24}+\dfrac{1}{x^2-14x+48}=\dfrac{1}{9}\)
3/ \(\dfrac{1}{x^2-3x+3}+\dfrac{2}{x^2-3x+4}=\dfrac{6}{x^2-3x+5}\)
4/ \(\dfrac{6}{\left(x+1\right)\left(x+2\right)}+\dfrac{8}{\left(x-1\right)\left(x+4\right)}=1\)
5/ \(4\left(x^3+\dfrac{1}{x^3}\right)=13\left(x+\dfrac{1}{x}\right)\)
6/ \(\dfrac{4x}{4x^2-8x+7}+\dfrac{3x}{4x^2-10x+7}=1\)
7/ \(\dfrac{x^2-3x+5}{x^2-4x+5}-\dfrac{x^2-5x+5}{x^2-6x+5}=-\dfrac{1}{4}\)
8/ \(x.\dfrac{8-x}{x-1}\left(x-\dfrac{8-x}{x-1}\right)=15\)
1) điều kiện xác định : \(x\notin\left\{-1;-2;-3;-4\right\}\)
ta có : \(\dfrac{1}{x^2+3x+2}+\dfrac{1}{x^2+5x+6}+\dfrac{1}{x^2+7x+12}=\dfrac{1}{6}\)
\(\Leftrightarrow\dfrac{1}{\left(x+1\right)\left(x+2\right)}+\dfrac{1}{\left(x+2\right)\left(x+3\right)}+\dfrac{1}{\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\) \(\Leftrightarrow\dfrac{\left(x+3\right)\left(x+4\right)+\left(x+1\right)\left(x+4\right)+\left(x+1\right)\left(x+2\right)}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)\(\Leftrightarrow\dfrac{x^2+7x+12+x^2+5x+4+x^2+3x+2}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)
\(\Leftrightarrow\dfrac{3x^2+15x+18}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)
\(\Leftrightarrow6\left(3x^2+15x+18\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18\left(x^2+5x+6\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18\left(x+2\right)\left(x+3\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18=\left(x+1\right)\left(x+4\right)\) ( vì điều kiện xác định )
\(\Leftrightarrow18=x^2+5x+4\Leftrightarrow x^2+5x-14=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+7\right)=0\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x+7=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-7\end{matrix}\right.\left(tmđk\right)\)
vậy \(x=2\) hoặc \(x=-7\) mấy câu kia lm tương tự nha bn
Đúng 0
Bình luận (0)
1.\(\left(x-5\right)^2+3\left(x-5\right)=0\)
\(2,\dfrac{2x-1}{3}-\dfrac{5x+2}{7}=x+13\)
\(3,\dfrac{x-1}{x+2}-\dfrac{x}{x-2}=\dfrac{7x-6}{4-x^2}\)
\(1\text{)}\left(x-5\right)^2+3\left(x-5\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(x-5+3\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(x-2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=2\\x=5\end{matrix}\right.\)
\(2\text{)}\dfrac{2x-1}{3}-\dfrac{5x+2}{7}=x+13\)
\(\Leftrightarrow\dfrac{7\left(2x-1\right)-3\left(5x+2\right)}{21}=\dfrac{21\left(x+13\right)}{21}\)
\(\Leftrightarrow14x-7-15x-6=21x+273\)
\(\Leftrightarrow-x-13=21x+273\)
\(\Leftrightarrow-22x=286\)
\(\Rightarrow x=-\dfrac{286}{22}=-\dfrac{143}{11}\)
\(3\text{)}\dfrac{x-1}{x+2}-\dfrac{x}{x-2}=\dfrac{7x-6}{4-x^2}\left(đk:x\ne\pm2\right)\)
\(\Leftrightarrow\dfrac{x-1}{2+x}+\dfrac{x}{2-x}=\dfrac{7x-6}{4-x^2}\)
\(\Leftrightarrow\dfrac{\left(x-1\right)\left(2-x\right)+x\left(2+x\right)}{4-x^2}=\dfrac{7x-6}{4-x^2}\)
\(\Leftrightarrow2x-x^2-2+x+2x+x^2=7x-6\)
\(\Leftrightarrow x-2=7x-6\)
\(\Leftrightarrow-6x=-4\)
\(\Rightarrow x=\dfrac{2}{3}\)
Đúng 2
Bình luận (0)
1.(x−5)2+3(x−5)=0
=>(x-5)(x-5)+3.(x-5)=0
=>(x-5).(x-5+3)=0
=>x-5=0 hoặc x-2=0
=>x=5 hoặc x=2
Đúng 0
Bình luận (2)
2)\(\dfrac{2x-1}{3}\)-\(\dfrac{5x+2}{7}\)=x+13
=>\(\dfrac{7.\left(2x-1\right)}{7.3}\)-\(\dfrac{3.\left(5x+2\right)}{3.7}\)=\(\dfrac{21.\left(x+13\right)}{21}\)
=>khử mẫu:
=>7.(2x-1)-3.(5x+2)=21.(x+13)
=>14x-7-15x-6=21x+273
=>14-7-15x-6-21x-273=0
=>-36x-272=0
=>-36x=272
=>x=...
Đúng 0
Bình luận (0)
rút gọn phân thức
a.\(\dfrac{5x-15}{4x+4}:\dfrac{x^2-9}{x^2+2x+1}\)
b.\(\dfrac{6x+48}{7x-7}:\dfrac{x^2-64}{x^2-2x+1}\)
c.\(\dfrac{4x-24}{5x+5}:\dfrac{x^2-36}{x^2+2x+1}\)
d.\(\left(\dfrac{1}{x^2+x}-\dfrac{2-x}{x+1}\right):\left(\dfrac{1}{x}+x-2\right)\)
a.\(\dfrac{5\left(x-3\right)}{4\left(x+1\right)}\) : \(\dfrac{\left(x-3\right)\left(x+3\right)}{\left(x+1\right)^2}\)
= \(\dfrac{5\left(x-3\right)}{4\left(x+1\right)}\). \(\dfrac{\left(x+1\right)^2}{\left(x-3\right)\left(x+3\right)}\)
= \(\dfrac{5\left(x+1\right)}{4\left(x+3\right)}\)
b. \(\dfrac{6\left(x+8\right)}{7\left(x-1\right)}\). \(\dfrac{\left(x-1\right)^2}{\left(x-8\right)\left(x+8\right)}\)
= \(\dfrac{6\left(x-1\right)}{7\left(x-8\right)}\)
c.Tương tự hai câu trên nka!!
d. (\(\dfrac{1}{x\left(x+1\right)}\)-\(\dfrac{2-x}{x+1}\)).(\(\dfrac{x}{x-1}\))
=( \(\dfrac{1}{x\left(x+1\right)}\)-\(\dfrac{2x-x^2}{x\left(x+1\right)}\)). ....
= \(\dfrac{\left(1-x\right)^2}{x\left(x+1\right)}\). ...
= \(\dfrac{x-1}{x+1}\)
Đúng 1
Bình luận (0)
Lê Cẩm TúThiên ThảoPhạm Thái DươnMai LinhgGuyoSky SơnTùngKhôi Bùi Mysterious PersonPhong ThầnPhùng Khánh Linhtran nguyen baNguyễn Xuân Sángo quanDƯƠNG PHAN KHDũng NguyễnÁNH DƯƠNGlê thị hương giang
Đúng 0
Bình luận (0)
c: \(=\dfrac{4\left(x-6\right)}{5\left(x+1\right)}\cdot\dfrac{\left(x+1\right)^2}{\left(x-6\right)\left(x+6\right)}=\dfrac{4\left(x+1\right)}{5\left(x+6\right)}\)
a: \(=\dfrac{5\left(x-3\right)}{4\left(x+1\right)}\cdot\dfrac{\left(x+1\right)^2}{\left(x-3\right)\left(x+3\right)}=\dfrac{5\left(x+1\right)}{4\left(x+3\right)}\)
b: \(=\dfrac{6\left(x+8\right)}{7\left(x-1\right)}\cdot\dfrac{\left(x-1\right)^2}{\left(x-8\right)\left(x+8\right)}=\dfrac{6\left(x-1\right)}{7\left(x-8\right)}\)
Đúng 0
Bình luận (0)
Giải các phương trình sau:a.dfrac{5x-2}{3}dfrac{5-3x}{2}b.dfrac{10x+3}{12}1+dfrac{6+8x}{9}c.2left(x+dfrac{3}{5}right)5-left(dfrac{13}{5}+xright)d.dfrac{7}{8}x-5left(x-9right)dfrac{20x+1,5}{6}e.dfrac{7x-1}{6}+2xdfrac{16-x}{5}f.dfrac{x+4}{5}-x+4dfrac{x}{3}-dfrac{x-2}{2}
Đọc tiếp
Giải các phương trình sau:
\(a.\dfrac{5x-2}{3}=\dfrac{5-3x}{2}\)
\(b.\dfrac{10x+3}{12}=1+\dfrac{6+8x}{9}\)
\(c.2\left(x+\dfrac{3}{5}\right)=5-\left(\dfrac{13}{5}+x\right)\)
\(d.\dfrac{7}{8}x-5\left(x-9\right)=\dfrac{20x+1,5}{6}\)
\(e.\dfrac{7x-1}{6}+2x=\dfrac{16-x}{5}\)
\(f.\dfrac{x+4}{5}-x+4=\dfrac{x}{3}-\dfrac{x-2}{2}\)
a: =>10x-4=15-9x
=>19x=19
hay x=1
b: \(\Leftrightarrow3\left(10x+3\right)=36+4\left(8x+6\right)\)
=>30x+9=36+32x+24
=>30x-32x=60-9
=>-2x=51
hay x=-51/2
c: \(\Leftrightarrow2x+\dfrac{6}{5}=5-\dfrac{13}{5}-x\)
=>3x=6/5
hay x=2/5
d: \(\Leftrightarrow\dfrac{7x}{8}-\dfrac{5\left(x-9\right)}{1}=\dfrac{20x+1.5}{6}\)
\(\Leftrightarrow21x-120\left(x-9\right)=4\left(20x+1.5\right)\)
=>21x-120x+1080=80x+60
=>-179x=-1020
hay x=1020/179
e: \(\Leftrightarrow5\left(7x-1\right)+60x=6\left(16-x\right)\)
=>35x-5+60x=96-6x
=>95x+6x=96+5
=>x=1
f: \(\Leftrightarrow6\left(x+4\right)+30\left(-x+4\right)=10x-15\left(x-2\right)\)
=>6x+24-30x+120=10x-15x+30
=>-24x+96=-5x+30
=>-19x=-66
hay x=66/19
Đúng 3
Bình luận (0)
Giải các hệ phương trình sau
f.{ (2x - y) (x + 3y) = 4
{ (5x + y) (x + 3y) = 24
g.{ \(\dfrac{8x-5y-3}{7}+\dfrac{11y-4x-7}{5}=12\)
{ \(\dfrac{9x+4y-13}{5}+\dfrac{3\left(x-2\right)}{4}=15\)
h.{\(\dfrac{1}{x}+\dfrac{1}{y}=2\)
{\(\dfrac{3}{x}-\dfrac{4}{y}=-1\)
h) \(\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=2\\\dfrac{3}{x}-\dfrac{4}{y}=-1\end{matrix}\right.\)\(\left(1\right)\)\(\left(đk:x,y\ne0\right)\)
Đặt \(a=\dfrac{1}{x},b=\dfrac{1}{y}\)
\(\left(1\right)\Leftrightarrow\) \(\left\{{}\begin{matrix}a+b=2\\3a-4b=-1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}3a+3b=6\\3a-4b=-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+b=2\\7b=7\end{matrix}\right.\)\(\Leftrightarrow a=b=1\)
Thay a,b:
\(\Leftrightarrow\dfrac{1}{x}=\dfrac{1}{y}=1\Leftrightarrow x=y=1\left(tm\right)\)
Đúng 1
Bình luận (0)