Tìm GTLN : \(\sqrt{x+1}-\sqrt{x-8}\)

Những câu hỏi liên quan
A=\(\dfrac{\sqrt{x}+1}{2\sqrt{x}-1}+\dfrac{\sqrt{x}}{\sqrt{x}+3}-\dfrac{x+6\sqrt{x}+2}{2x+5\sqrt{x}-3}\) B=\(\dfrac{\sqrt{x}+3}{x+8}\) Tìm GTLN: P=AB
Cho biểu thức A =\(\left(\frac{x+2}{x\sqrt{x}-1}+\frac{\sqrt{x}}{x+\sqrt{x}+1}+\frac{1}{1-\sqrt{x}}\right):\frac{\sqrt{x}-1}{2}\)
Tìm x để A đạt GTLN, tìm GTLN đó
1tìm x để \(\frac{2-5\sqrt{x}}{\sqrt{x}+3}=\frac{-8\sqrt{x}+5}{3\sqrt{x}+1}\)
2 tìm GTLN của P=\(\frac{2-5\sqrt{x}}{\sqrt{x}+3}\)
2/ \(P=\frac{2-5\sqrt{x}}{\sqrt{x}+3}=-5+\frac{17}{\sqrt{x}+3}\)
Ta thấy rằng mẫu là số dương nên để P lớn nhất thì mẫu bé nhất hay x = 0
\(P=\frac{2}{3}\)
Đúng 0
Bình luận (0)
1/ Đặt \(\sqrt{x}=a\:voi\:a\ge0\) thì pt thành
\(\frac{2-5a}{a+3}=\frac{5-8a}{3a+1}\)
\(\Leftrightarrow7a^2-20a+13=0\)
<=> (a - 1)(7a - 13) = 0
Đúng 0
Bình luận (0)
Giải tiếp câu 1/
\(\Leftrightarrow\orbr{\begin{cases}x=1\\x=\frac{13}{7}\end{cases}}\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Tìm GTNN của:
a)\(\dfrac{\sqrt{x}-1}{\sqrt{x}+1}\)
b)\(\dfrac{x+\sqrt{x}+1}{\sqrt{x}}\)
Tìm GTLN của:
\(\dfrac{1}{\dfrac{\left(\sqrt{x}+1\right)^2}{\sqrt{x}}}\)
1:
a: \(A=\dfrac{\sqrt{x}+1-2}{\sqrt{x}+1}=1-\dfrac{2}{\sqrt{x}+1}\)
căn x+1>=1
=>2/căn x+1<=2
=>-2/căn x+1>=-2
=>A>=-2+1=-1
Dấu = xảy ra khi x=0
b: 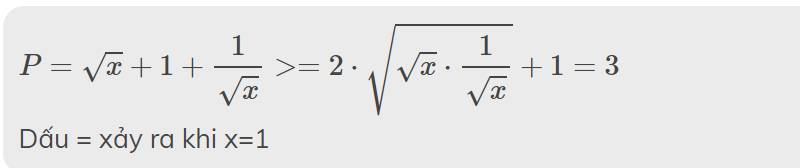
Đúng 0
Bình luận (0)
1) giải phương trình
a)\(\sqrt{x+3-4\sqrt{x-1}}+\sqrt{x+8-6\sqrt{x-1}=1}\)
b)\(\sqrt{x+\sqrt{x-11}}+\sqrt{x-\sqrt{x-11}}=4\)
2) Tìm GTLN của biểu thúc
M=\(\sqrt{x-2}+\sqrt{4-x}\)
a, \(\left(\sqrt{x-1}-2\right)^2+\)\(\left(\sqrt{x-1}-3\right)^2\)
xog xét 2 TH
b, bình phương
2
GTLN : 2 dấu = xra \(2\le x\le4\)
Đúng 0
Bình luận (0)
Hà Thị Thế pạn làm ra lun giúp mjk dx k ạ
Đúng 0
Bình luận (0)
Bài 1:
A=\(\left(\dfrac{x+2}{x\sqrt{x}-1}+\dfrac{\sqrt{x}}{x+\sqrt{x}+1}+\dfrac{1}{1-\sqrt{x}}\right):\dfrac{\sqrt{x}-1}{2}\)
a) Tìm tập xác định của biểu thức A
b) Rút gọn biểu thức A
c) Chứng minh rằng A>0 với mọi x≠1
d) Tìm x để A đạt GTLN, tìm GTLN đó
a: ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne1\end{matrix}\right.\)
b: Ta có: \(A=\left(\dfrac{x+2}{x\sqrt{x}-1}+\dfrac{\sqrt{x}}{x+\sqrt{x}+1}+\dfrac{1}{1-\sqrt{x}}\right):\dfrac{\sqrt{x}-1}{2}\)
\(=\dfrac{x+2+x-\sqrt{x}-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\dfrac{2}{\sqrt{x}-1}\)
\(=\dfrac{2}{x+\sqrt{x}+1}\)
Đúng 0
Bình luận (0)
c: Ta có: \(x+\sqrt{x}+1>0\forall x\) thỏa mãn ĐKXĐ
\(\Leftrightarrow\dfrac{2}{x+\sqrt{x}+1}>0\forall x\)
Đúng 0
Bình luận (0)
Cho 2 biểu thức A= \(\dfrac{7}{\sqrt{x}+8}\) và B=\(\dfrac{\sqrt{x}}{\sqrt{x}-3}+\dfrac{2\sqrt{x}-24}{x-9}\)
a) Chứng minh B= \(\dfrac{\sqrt{x}+8}{\sqrt{x}+3}\)
b) Tìm GTLN của B
c) Tìm số nguyên x để biểu thức P = A.B có giá trị là số nguyên.
giúp mk vs mk like cho
tìm GTLN \(A=\sqrt{x+1}-\sqrt{x-8}\)
Trục căn thức: (điều kiện: \(x\ge8\))
\(A=\frac{x+1-\left(x-8\right)}{\sqrt{x+1}+\sqrt{x-8}}=\frac{9}{\sqrt{x+1}+\sqrt{x-8}}\le\frac{9}{\sqrt{8+1}+0}=\frac{9}{3}=3\)
Đúng 0
Bình luận (0)
\(A=\sqrt{x+1}+\sqrt{8-x}\)
Xét \(A^2=x+1+2\sqrt{\left(x+1\right)\left(8-x\right)}+8-x\)
\(\Leftrightarrow A^2=9+2\sqrt{\left(x+1\right)\left(8-x\right)}\)
Áp dụng bất đẳng thức \(2\sqrt{ab}\le a+b\)ta có
\(A^2\le9+\left(x+1\right)+\left(8-x\right)=18\)
\(\Rightarrow\)max \(A=3\sqrt{2}\)\(\Leftrightarrow x+1=8-x\Leftrightarrow x=3,5\)
Đúng 0
Bình luận (0)
Tìm GTLN của \(\dfrac{\sqrt{x}-1}{x+\sqrt{x}+2}\)
Đặt A=\(\dfrac{\sqrt{x}-1}{x+\sqrt{x}+2}\)\(\Rightarrow Ax+A\sqrt{x}+2A-\sqrt{x}+1=0\)
\(\Leftrightarrow Ax+\sqrt{x}\left(A-1\right)+2A+1=0\)
\(\Delta=\left(A-1\right)^2-4A\left(2A+1\right)=A^2-2A+1-8A^2-4A\)\(=-7A^2-6A+1\ge0\)
\(\Rightarrow-1\le A\le\dfrac{1}{7}\)
Vậy Max A là \(\dfrac{1}{7}\)
Dâu"=" xảy ra \(\Leftrightarrow A=\dfrac{1}{7}\)
\(\Leftrightarrow7\sqrt{x}-7=x+\sqrt{x}+2\)
\(\Leftrightarrow x-6\sqrt{x}+9=0\)
\(\Leftrightarrow\left(\sqrt{x}-3\right)^2=0\Leftrightarrow x=9\)
Đúng 0
Bình luận (0)
Tìm GTLN :
\(M=\dfrac{\sqrt{x}+1}{x\sqrt{x}+7}\)
ĐK: \(x\ge0\)
Mà: \(\left\{{}\begin{matrix}\sqrt{x}\ge0\forall x\left(xđ\right)\\x\sqrt{x}\ge0\forall x\left(xđ\right)\end{matrix}\right.\)
Nên: \(M=\dfrac{\sqrt{x}+1}{x\sqrt{x}+7}\le\dfrac{1}{7}\forall x\left(xđ\right)\)
Dấu "=" xảy ra:
\(\dfrac{\sqrt{x}+1}{x\sqrt{x}+7}=\dfrac{1}{7}\)
\(\Leftrightarrow7\sqrt{x}+7=x\sqrt{x}+7\)
\(\Leftrightarrow x\sqrt{x}-7\sqrt{x}=0\)
\(\Leftrightarrow\sqrt{x}\left(x-7\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=7\end{matrix}\right.\left(tm\right)\)
Vậy: \(M_{max}=\dfrac{1}{7}\) khi \(\left[{}\begin{matrix}x=0\\x=7\end{matrix}\right.\)
Đúng 1
Bình luận (0)

























