A = cos2x. cot2x + 3cos2x - cot2x - 2sin2x
Chứng minh biểu thức sau không phụ thuộc vào x.
Rút gọn biểu thức A = cos2x.cot2x + 3cos2x - cot2x + 2sin2x không phụ thuộc vào x và bằng
A. 2.
B. -2.
C. 1.
D. -1.
Chọn A.
Ta có: A = cos2x.cot2x + 3cos2x - cot2x + 2sin2x
=( cos2x.cot2x - cot2x) + (2sin2x + 2cos2x) + cos2x
= cot2x( cos2x - 1) + 2 + cos2x
= - cot2x. sin2x + 2 + cos2x
= -cos2x + 2 + cos2x = 2
Chứng minh biểu thức sau không phụ thuộc vào x:
A = 1 sin 4 x + 1 sin 8 x + 1 sin 16 x - c o t 2 x + c o t 16 x
chứng minh
a> cot2x/1+cot2x . 1+tan2x/tan2x = tan2x+cot2x/1=tan4x
b>tan2x-cos2x/sin2x + cot2x-sin2x/cos2x = 2
a: \(VT=\dfrac{cot^2x}{1+cot^2x}\cdot\dfrac{1+tan^2x}{tan^2x}\)
\(=\dfrac{cot^2x}{\dfrac{1}{sin^2x}}\cdot\dfrac{\dfrac{1}{cos^2x}}{tan^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{1}{cos^2x}:\dfrac{1}{sin^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{sin^2x}{cos^2x}\)
\(=cot^2x\)
\(VP=\dfrac{tan^2x+cot^2x}{1+tan^4x}=\dfrac{\dfrac{sin^2x}{cos^2x}+\dfrac{cos^2x}{sin^2x}}{1+\dfrac{sin^4x}{cos^4x}}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}:\dfrac{cos^4x+sin^4x}{cos^4x}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}\cdot\dfrac{cos^4x}{cos^4x+sin^4x}=\dfrac{cos^2x}{sin^2x}=cot^2x\)
=>VT=VP
b:
\(\dfrac{tan^2x-cos^2x}{sin^2x}+\dfrac{cot^2x-sin^2x}{cos^2x}\)
\(=\dfrac{\left(\dfrac{sinx}{cosx}\right)^2-cos^2x}{sin^2x}+\dfrac{\left(\dfrac{cosx}{sinx}\right)^2-sin^2x}{cos^2x}\)
\(=\dfrac{sin^2x-cos^4x}{cos^2x\cdot sin^2x}+\dfrac{cos^2x-sin^4x}{sin^2x\cdot cos^2x}\)
\(=\dfrac{sin^2x+cos^2x-cos^4x-sin^4x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{1-\left(cos^2x+sin^2x\right)^2+2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}=2\)
Chứng minh biểu thức sau không phụ thuộc vào x:
A = cos 6 x + 3 sin 2 x . cos 2 x + 2 sin 4 α . cos 2 x + sin 4 α
A = cos 6 x + 3 sin 2 x . cos 2 x + 2 sin 4 α . cos 2 x + sin 4 α
= cos 6 x + 3.(1 - cos 2 x ) cos 4 x + 2 sin 4 α . cos 2 x + sin 4 α
= cos 6 x + 3 cos 4 x - 3 cos 6 x + 2. sin 4 α .(1 - sin 2 x ) + sin 4 α= cos 6 x + 3 cos 4 x - 3 cos 6 x + 2 sin 4 α - 2 sin 6 x + sin 4 α
= -2.( cos 6 x + sin 6 x ) + 3 cos 4 x + 3 sin 4 α
= -2.( cos 6 x + sin 6 x ) + 3.( cos 4 x + sin 4 α ) = 1
Vậy biểu thức A không phụ thuộc vào x.
Chứng minh rằng giá trị của biểu thức sau không phụ thuộc vào x.
A = 2( sin 4 x + cos 4 x + sin 2 x . cos 2 x ) 2 - ( sin 8 x + cos 8 x )
Ta có:
![]()
![]()
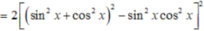

![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Vậy giá trị của biểu thức  không phụ thuộc vào x.
không phụ thuộc vào x.
chứng minh biểu thức sau đây không phụ thuộc vào y:
A= \(\frac{1}{sin2y}+\frac{1}{sin4y}+\frac{1}{sin8y}-coty+cot8y\)
B= \(\frac{1}{sin4x}+\frac{1}{sin8x}+\frac{1}{sin16x}-cot2x+cot16x\)
\(\frac{1}{sin2a}=\frac{sina}{sina.sin2a}=\frac{sin\left(2a-a\right)}{sina.sin2a}=\frac{sin2a.cosa-cos2a.sina}{sina.sin2a}\)
\(=\frac{sin2a.cosa}{sina.sin2a}-\frac{cos2a.sina}{sina.cos2a}=\frac{cosa}{sina}-\frac{cos2a}{sin2a}=cota-cot2a\)
Áp dụng vào bài toán:
\(A=\frac{1}{sin2y}+\frac{1}{sin2\left(2y\right)}+\frac{1}{sin2\left(4y\right)}-coty+cot8y\)
\(=coty-cot2y+cot2y-cot4y+cot4y-cot8y-coty+cot8y\)
\(=0\)
\(B=\frac{1}{sin2\left(2x\right)}+\frac{1}{sin2\left(2x\right)}+\frac{1}{sin2\left(8x\right)}-cot2x+cot16x\)
\(=cot2x-cot4x+cot4x-cot8x+cot8x-cot16x-cot2x+cot16x\)
\(=0\)
Đơn giản biểu thức A = (1 - sin 2 x ) c o t 2 x + (1 - c o t 2 x ), ta có:
A. A = - cos 2 x
B. A = cos 2 x
C. A = sin 2 x
D. A = - sin 2 x
Đáp án: C
Ta có:
A = (1 - sin 2 x ) c o t 2 x + (1 - c o t 2 x ) = c o t 2 x - sin 2 x . c o t 2 x + 1 - c o t 2 x
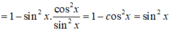
Đơn giản biểu thức A = (1 - sin2x) .cot2x + (1 - cot2x) ta được :
A. sin2x
B. 2
C. 1
D. cot2x
Rút gọn biểu thức sau A = c o t 2 x - cos 2 x c o t 2 x + sin x . cos x c o t x
A. A = 1
B. A = -1
C. A = -2
D. A = 2
Đơn giản biểu thức C = (1- sin2x) cot2x + 1 - cot2x.
A. sin2x
B. tan2x
C. cot2x
D. cos2x
Chọn A.
Ta có C = (1-sin2x) cot2x + 1 - cot2x.
= (1 - sin2x - 1) cot2x + 1
= -sin2x.cot2x + 1 = -cos2x + 1 = sin2x.