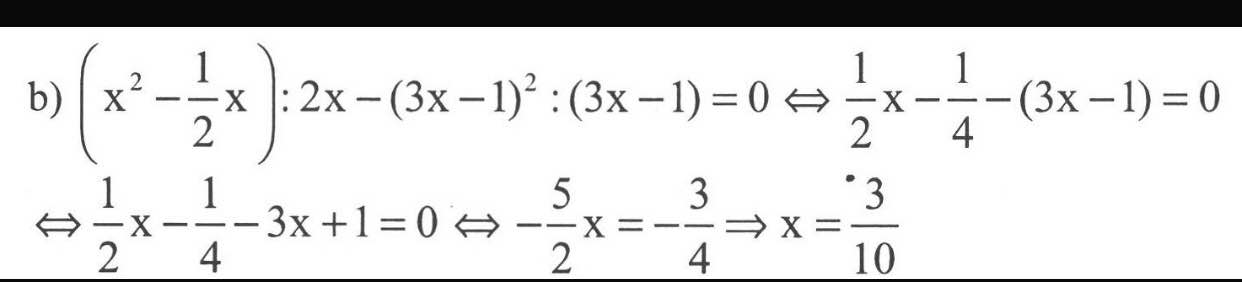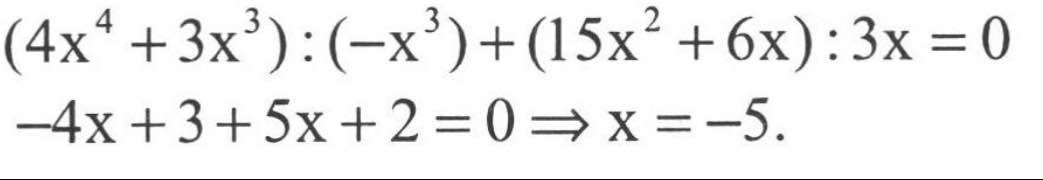a) Ta có: \(3x^2+2x-1=0\)

\(\Leftrightarrow3x^2+3x-x-1=0\)

\(\Leftrightarrow3x\left(x+1\right)-\left(x+1\right)=0\)

\(\Leftrightarrow\left(x+1\right)\left(3x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x+1=0\\3x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\3x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=\dfrac{1}{3}\end{matrix}\right.\)

Vậy: \(S=\left\{-1;\dfrac{1}{3}\right\}\)

b) Ta có: \(x^2-5x+6=0\)

\(\Leftrightarrow x^2-2x-3x+6=0\)

\(\Leftrightarrow x\left(x-2\right)-3\left(x-2\right)=0\)

\(\Leftrightarrow\left(x-2\right)\left(x-3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\)

Vậy: S={2;3}

c) Ta có: \(x^2-3x+2=0\)

\(\Leftrightarrow x^2-x-2x+2=0\)

\(\Leftrightarrow x\left(x-1\right)-2\left(x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(x-2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)

Vậy: S={1;2}

d) Ta có: \(2x^2-6x+1=0\)

\(\Leftrightarrow2\left(x^2-3x+\dfrac{1}{3}\right)=0\)

mà \(2\ne0\)

nên \(x^2-3x+\dfrac{1}{3}=0\)

\(\Leftrightarrow x^2-2\cdot x\cdot\dfrac{3}{2}+\dfrac{9}{4}-\dfrac{23}{12}=0\)

\(\Leftrightarrow\left(x-\dfrac{3}{2}\right)^2=\dfrac{23}{12}\)

\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{3}{2}=\dfrac{\sqrt{69}}{6}\\x-\dfrac{3}{2}=\dfrac{-\sqrt{69}}{6}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{9+\sqrt{69}}{6}\\x=\dfrac{9-\sqrt{69}}{6}\end{matrix}\right.\)

Vậy: \(S=\left\{\dfrac{9+\sqrt{69}}{6};\dfrac{9-\sqrt{69}}{6}\right\}\)

e) Ta có: \(4x^2-12x+5=0\)

\(\Leftrightarrow4x^2-10x-2x+5=0\)

\(\Leftrightarrow2x\left(2x-5\right)-\left(2x-5\right)=0\)

\(\Leftrightarrow\left(2x-5\right)\left(2x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}2x-5=0\\2x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=5\\2x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=\dfrac{1}{2}\end{matrix}\right.\)

Vậy: \(S=\left\{\dfrac{5}{2};\dfrac{1}{2}\right\}\)

cho vào máy tính là ra hết

Đây nhé! Tích giúp mình nha

Ta có: \(\dfrac{4x^4+3x^3}{-x^3}+\dfrac{15x^2+6x}{3x}=0\)

\(\Leftrightarrow-4x-3+5x+2=0\)

\(\Leftrightarrow x-1=0\)

hay x=1

Giải như sau.

(1)+(2)⇔x2−2x+1+√x2−2x+5=y2+√y2+4⇔(x2−2x+5)+√x2−2x+5=y2+4+√y2+4⇔√y2+4=√x2−2x+5⇒x=3y(1)+(2)⇔x2−2x+1+x2−2x+5=y2+y2+4⇔(x2−2x+5)+x2−2x+5=y2+4+y2+4⇔y2+4=x2−2x+5⇒x=3y

⇔√y2+4=√x2−2x+5⇔y2+4=x2−2x+5, chỗ này do hàm số f(x)=t2+tf(x)=t2+t đồng biến ∀t≥0∀t≥0
Công việc còn lại là của bạn ! 

\(\left(x+6\right)\left(2x+1\right)=0\)

<=>  \(\orbr{\begin{cases}x+6=0\\2x+1=0\end{cases}}\)

<=>  \(\orbr{\begin{cases}x=-6\\x=-\frac{1}{2}\end{cases}}\)

Vậy....

hk tốt

^^

c, |2\(x\) + 1| + |3\(x\) - 1| = 0

   vì |2\(x\) + 1| ≥ 0; |3\(x\) - 1| = 0

  ⇒ |2\(x\) + 1| + |3\(x\) - 1| = 0

   ⇔ \(\left\{{}\begin{matrix}2x+1=0\\3x-1=0\end{matrix}\right.\)

   ⇔ \(\left\{{}\begin{matrix}2x=-1\\3x=1\end{matrix}\right.\)

   \(\Rightarrow\) \(\left\{{}\begin{matrix}x=-\dfrac{1}{2}\\x=\dfrac{1}{3}\end{matrix}\right.\)

       \(-\dfrac{1}{2}\) < \(\dfrac{1}{3}\) 

Vậy \(x\) \(\in\) \(\varnothing\)

a, Nếu 4.|3\(x\) - 1| = |6\(x\) - 2| + |-1,5|

             4.|3\(x\) -1| - 2.|3\(x\) - 1|  = 1,5

           Nếu 3\(x\) - 1 ≥ 0 ⇒ \(x\) ≥ \(\dfrac{1}{3}\)

Ta có: 4.(3\(x\) - 1) - 2.(3\(x\) - 1) = 1,5

           12\(x\) - 4 - 6\(x\) + 2 = 1,5

            6\(x\) - 2  = 1,5

            6\(x\)        = 1,5 + 2

            6\(x\)       = 3,5

               \(x\)      = 3,5: 6

                \(x\)    = \(\dfrac{7}{12}\)

Nếu 3\(x\) - 1 < 0 ⇒ \(x\) < \(\dfrac{1}{3}\)

Ta có: - 4.(3\(x\) - 1) = - (6\(x\) - 2) + 1,5

           -12\(x\) + 4 + 6\(x\) - 2 = 1,5

             -6\(x\) + 2 = 1,5

              6\(x\)         = 2- 1,5

              6\(x\)          = 0,5

                 \(x\)         = 0,5 : 6

                 \(x\)        = \(\dfrac{1}{12}\)

Vậy \(x\) \(\in\) {\(\dfrac{1}{12}\); \(\dfrac{7}{12}\)}

b, 2024.|2\(x\) - 1| = 2025.|1 - 2\(x\)| - |-2|

    2025.|1 - 2\(x\)| - 2024.|1 - 2\(x\)| = |-2|

             |1 - 2\(x\)| = 2 

             Nếu 1 - 2\(x\) ≥ 0 ⇒ \(x\) ≥ \(\dfrac{1}{2}\)

       với \(x\)    ≥ \(\dfrac{1}{2}\)  ta có: 1 - 2\(x\) = 2 ⇒ 2\(x\) = -1 ⇒ \(x\) = - \(\dfrac{1}{2}\) (1)

      Nếu \(1-2x\) < 0 ⇒ 2\(x\) ≤ 1 ⇒ \(x\) < \(\dfrac{1}{2}\) 

Với \(x\) < \(\dfrac{1}{2}\) ta có: -1 + 2\(x\) = 2 ⇒ 2\(x\) = 3 ⇒ \(x\) = \(\dfrac{3}{2}\) (2)

Kết hợp(1) và (2) ta có: \(x\) \(\in\) { - \(\dfrac{1}{2}\); \(\dfrac{3}{2}\)}

x4−3x3−2x2+6x+4=0x4−3x3−2x2+6x+4=0

⇔x4−2x3−2x2−x3+2x2+2x−2x2+4x+4=0⇔x4−2x3−2x2−x3+2x2+2x−2x2+4x+4=0

⇔x2(x2−2x−2)−x(x2−2x−2)−2(x2−2x−2)=0⇔x2(x2−2x−2)−x(x2−2x−2)−2(x2−2x−2)=0

⇔(x2−x−2)(x2−2x−2)=0⇔(x2−x−2)(x2−2x−2)=0

⇔(x+1)(x−2)(x−1−√3)(x−1+√3)=0⇔(x+1)(x−2)(x−1−3)(x−1+3)=0

⇔⎡⎢ ⎢ ⎢ ⎢⎣x=−1x=2x=1+√3x=1−√3

tl

x4−3x3−2x2+6x+4=0x4−3x3−2x2+6x+4=0

⇔x4−2x3−2x2−x3+2x2+2x−2x2+4x+4=0⇔x4−2x3−2x2−x3+2x2+2x−2x2+4x+4=0

⇔x2(x2−2x−2)−x(x2−2x−2)−2(x2−2x−2)=0⇔x2(x2−2x−2)−x(x2−2x−2)−2(x2−2x−2)=0

⇔(x2−x−2)(x2−2x−2)=0⇔(x2−x−2)(x2−2x−2)=0

⇔(x+1)(x−2)(x−1−√3)(x−1+√3)=0⇔(x+1)(x−2)(x−1−3)(x−1+3)=0

⇔⎡⎢ ⎢ ⎢ ⎢⎣x=−1x=2x=1+√3x=1−√3

^HT^

Ta thấy x=0 không là nghiệm của phương trình

chia cả 2 vế cho x^2 ta được:

PT <=> x^2-3x-6+3/x+1/(x^2)=0

       <=> (x^2-2+1/(x^2))-3(x-1/x)-4=0

      <=> (x-1/x)^2-3(x-1/x)-4=0

Đặt x-1/x=y

PT <=> y^2-3y-4=0

     <=> y=-4 hoặc y=1

Tại y=-4 , ta có x+1/x+4=0

                       <=> x^2+4x+1=0

                       <=> x=-2+ √3 hoăc x=-2-  √ 3

Tại y=1 ta có x^2-x-1=0

                 <=> x=(1- √  5)/2 hoặc x=(1+  √5)/2

de qua

x.(2.x-1)+1/3-2/3.x=0

c.

ĐLXĐ: \(x\ge-\dfrac{1}{3}\)

\(-\left(3x+1\right)+\sqrt{3x+1}+4x^2-10x+6=0\)

Đặt \(\sqrt{3x+1}=t\ge0\)

\(\Rightarrow-t^2+t+4x^2-10x+6=0\)

\(\Delta=1+4\left(4x^2-10x+6\right)=\left(4x-5\right)^2\)

\(\Rightarrow\left[{}\begin{matrix}t=\dfrac{-1+4x-5}{-2}=3-2x\\t=\dfrac{-1-4x+5}{-2}=2x-2\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{3x+1}=3-2x\left(x\le\dfrac{3}{2}\right)\\\sqrt{3x-1}=2x-2\left(x\ge1\right)\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}3x+1=4x^2-12x+9\left(x\le\dfrac{3}{2}\right)\\3x-1=4x^2-8x+4\left(x\ge1\right)\end{matrix}\right.\)

\(\Leftrightarrow...\)

b.

ĐKXĐ: \(x\ge-\dfrac{61}{12}\)

\(\Leftrightarrow36x^2+12x-58-2\sqrt{12x+61}=0\)

\(\Leftrightarrow\left(36x^2+24x+4\right)-\left(12x+61+2\sqrt{12x+61}+1\right)=0\)

\(\Leftrightarrow\left(6x+2\right)^2-\left(\sqrt{12x+61}+1\right)^2=0\)

\(\Leftrightarrow\left(6x+1-\sqrt{12x+61}\right)\left(6x+3+\sqrt{12x+61}\right)=0\)

\(\Leftrightarrow...\) tương tự câu a

a.

ĐKXĐ: \(x\ge-\dfrac{5}{4}\)

\(\Leftrightarrow4x^2-12x-2-2\sqrt{4x+5}=0\)

\(\Leftrightarrow\left(4x^2-8x+4\right)-\left(4x+5+2\sqrt{4x+5}+1\right)=0\)

\(\Leftrightarrow\left(2x-2\right)^2-\left(\sqrt{4x+5}+1\right)^2=0\)

\(\Leftrightarrow\left(2x-2-\sqrt{4x+5}-1\right)\left(2x-2+\sqrt{4x+5}+1\right)=0\)

\(\Leftrightarrow\left(2x-3-\sqrt{4x+5}\right)\left(2x-1+\sqrt{4x+5}\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{4x+5}=2x-3\left(x\ge\dfrac{3}{2}\right)\\\sqrt{4x+5}=1-2x\left(x\le\dfrac{1}{2}\right)\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}4x+5=4x^2-12x+9\left(x\ge\dfrac{3}{2}\right)\\4x+5=4x^2-4x+1\left(x\le\dfrac{1}{2}\right)\end{matrix}\right.\)

\(\Leftrightarrow...\)