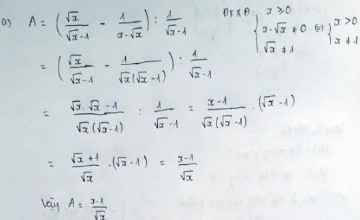1. c

2. b

3. d

4. c

5. a

6. b

7. c

8. a

9. d

10. b

a: ĐKXĐ x>0; x<>1

\(A=\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\sqrt{x}-1}{1}=\dfrac{x-1}{\sqrt{x}}\)

b: A<0

=>x-1<0

=>0<x<1

Gọi số người mua là x(người), doanh thu là y(đồng)

(Điều kiện: \(x\in Z^+;y>0\))

Vì doanh thu bằng số người mua nhân với lại giá của bộ quần áo nên y=320000x(đồng)

=>\(320000=\dfrac{y}{x}\)

Số người mua tăng lên 60% và doanh thu cũng tăng thêm 30% nên giá mới sẽ là:

\(\dfrac{y\cdot\left(1+30\%\right)}{x\left(1+60\%\right)}=\dfrac{y}{x}\cdot\dfrac{13}{16}=320000\cdot\dfrac{13}{16}=260000\left(đồng\right)\)

\(9,PT\Leftrightarrow x-6=3x-7\left(x\ge6\right)\\ \Leftrightarrow x=\dfrac{1}{2}\left(ktm\right)\\ \Leftrightarrow x\in\varnothing\\ 10,PT\Leftrightarrow3x-2=4x^2-4x+1\left(x\le\dfrac{1}{2}\right)\\ \Leftrightarrow4x^2-7x+3=0\\ \Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{3}{4}\end{matrix}\right.\left(ktm\right)\Leftrightarrow x\in\varnothing\\ 11,PT\Leftrightarrow\sqrt{x^2+x-1}=2-x\left(x\le2\right)\\ \Leftrightarrow x^2+x-1=x^2-4x+4\\ \Leftrightarrow5x=5\Leftrightarrow x=1\left(tm\right)\\ 12,PT\Leftrightarrow\left(\sqrt{20-x}-4\right)+\left(\sqrt{x+5}-3\right)=0\left(5\le x\le20\right)\\ \Leftrightarrow\dfrac{4-x}{\sqrt{20-x}+4}+\dfrac{x-4}{\sqrt{x+5}+3}=0\\ \Leftrightarrow\left(x-4\right)\left(\dfrac{1}{\sqrt{x+5}+3}-\dfrac{1}{\sqrt{20-x}+4}\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=4\left(tm\right)\\\dfrac{1}{\sqrt{x+5}+3}=\dfrac{1}{\sqrt{20-x}+4}\left(1\right)\end{matrix}\right.\\ \left(1\right)\Leftrightarrow\sqrt{x+5}+3=\sqrt{20-x}+4\\ \Leftrightarrow\left(\sqrt{x+5}-4\right)-\left(\sqrt{20-x}-3\right)=0\\ \Leftrightarrow\dfrac{x-11}{\sqrt{x+5}+4}+\dfrac{x-11}{\sqrt{20-x}+3}=0\\ \Leftrightarrow\left(x-11\right)\left(\dfrac{1}{\sqrt{x+5}+4}+\dfrac{1}{\sqrt{20-x}+3}\right)=0\\ \Leftrightarrow x=11\left(\dfrac{1}{\sqrt{x+5}+4}+\dfrac{1}{\sqrt{20-x}+3}>0\right)\\ \text{Vậy PT có nghiệm }x\in\left\{4;11\right\}\)

\(13,PT\Leftrightarrow\sqrt{x-1}+\sqrt{3x-2}=\sqrt{5x+1}\left(x\ge-\dfrac{1}{5}\right)\\ \Leftrightarrow4x-3+2\sqrt{\left(x-1\right)\left(3x-2\right)}=5x+1\\ \Leftrightarrow x+4=2\sqrt{3x^2-5x+2}\\ \Leftrightarrow x^2+8x+16=12x^2-20x+8\\ \Leftrightarrow11x^2-28x-8=0\\ \Delta'=14^2+8\cdot11=284\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{14-2\sqrt{71}}{11}\\x=\dfrac{14+2\sqrt{71}}{11}\end{matrix}\right.\)

\(14,ĐK:x\ge-1\)

Đặt \(\sqrt{x+1}=a\ge0\)

\(PT\Leftrightarrow2\sqrt{a^2-1+2a}-a=4\\ \Leftrightarrow2\sqrt{a^2+2a-1}=a+4\\ \Leftrightarrow4a^2+8a-4=a^2+8a+16\\ \Leftrightarrow3a^2-20=0\\ \Leftrightarrow a^2=\dfrac{20}{3}\Leftrightarrow x+1=\dfrac{20}{3}\Leftrightarrow x=\dfrac{17}{3}\left(tm\right)\)

\(15,ĐK:-3\le x\le6\)

Đặt \(\sqrt{x+3}+\sqrt{6-x}=a\ge0\)

\(\Leftrightarrow\dfrac{a^2-9}{2}=\sqrt{\left(x+3\right)\left(6-x\right)}\\ PT\Leftrightarrow a-\dfrac{a^2-9}{2}=3\\ \Leftrightarrow2a-a^2+9=6\\ \Leftrightarrow a^2-2a-3=0\\ \Leftrightarrow a=3\left(a\ge0\right)\\ \Leftrightarrow\sqrt{x+3}+\sqrt{6-x}=3\\ \Leftrightarrow\sqrt{x+3}-3+\sqrt{6-x}=0\\ \Leftrightarrow\dfrac{x-6}{\sqrt{x+3}+3}-\dfrac{x-6}{\sqrt{6-x}}=0\\ \Leftrightarrow\left[{}\begin{matrix}x=6\left(tm\right)\\\dfrac{1}{\sqrt{x+3}+3}=\dfrac{1}{\sqrt{6-x}}\left(1\right)\end{matrix}\right.\\ \left(1\right)\Leftrightarrow\sqrt{x+3}+3=\sqrt{6-x}\\ \Leftrightarrow\sqrt{x+3}-\left(\sqrt{6-x}-3\right)=0\\ \Leftrightarrow\dfrac{x+3}{\sqrt{x+3}}+\dfrac{x+3}{\sqrt{6-x}+3}=0\\ \Leftrightarrow x=-3\left(\dfrac{1}{\sqrt{x+3}}+\dfrac{1}{\sqrt{6-x}+3}>0\right)\\ \text{Vậy PT có nghiệm }x\in\left\{6;-3\right\}\) 

\(16,\) Đặt \(\sqrt{x^2-6x+6}=a\ge0\)

\(PT\Leftrightarrow a^2+3=4a\\ \Leftrightarrow a^2-4a+3=0\\ \Leftrightarrow\left[{}\begin{matrix}a=1\\a=3\end{matrix}\right.\)

Với \(a=1\Leftrightarrow x^2-6x+5=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=5\end{matrix}\right.\)

Với \(a=3\Leftrightarrow x^2-6x-3=0\Leftrightarrow\left[{}\begin{matrix}x=3+2\sqrt{3}\\x=3-2\sqrt{3}\end{matrix}\right.\)

Vậy PT có nghiệm \(x\in\left\{1;5;3+2\sqrt{3};3-2\sqrt{3}\right\}\)

Các bài thơ xoay quanh chủ đề quê hương:

"Quê hương đẹp mãi trong tôi

Dòng sông bên lở bên bồi uốn quanh

Cánh cò bay lượn chòng chành

Đàn bò gặm cỏ đồng xanh mượt mà

Sáo diều trong gió ngân nga

Bình yên thanh đạm chan hòa yêu thương

Bức tranh đẹp tựa thiên đường

Hồn thơ trỗi dậy nặng vương nghĩa tình."

( Bức tranh quê - Hà Thu )

"Bút chì xanh đỏ

Em gọt hai đầu

Em thử hai màu

Xanh tươi, đỏ thắm

Em vẽ làng xóm

Tre xanh, lúa xanh

Sông máng lượn quanh

Một dòng xanh mát

Trời mây bát ngát

Xanh ngắt mùa thu

Xanh màu ước mơ…

Em quay đầu đỏ

Vẽ nhà em ở

Ngói mới đỏ tươi

Trường học trên đồi

Em tô đỏ thắm

Cây gạo đầu xóm

Hoa nở chói ngời

A, nắng lên rồi

Lá cờ Tổ quốc

Bay giữa trời xanh…

Chị ơi bức tranh

Quê ta đẹp quá!"

( Vẽ quê hương - Định Hải )

\(\frac{-3}{7}\).\(^{\left(-3\right)^2}\)-\(\sqrt{\frac{4}{49}}\)

= \(\frac{-3}{7}.9-\sqrt{\frac{4}{49}}\)

=\(\frac{-27}{7}-\sqrt{\frac{4}{49}}\)

=\(\frac{-27}{7}-\frac{2}{7}\)

=\(\frac{-29}{7}\)

Chúc bạn học tốt

\(\left|-\frac{3}{7}\right|\cdot(-3)^2-\sqrt{\frac{4}{49}}\)

\(=\frac{3}{7}\cdot9-\frac{2}{7}\)

\(=\frac{27}{7}-\frac{2}{7}=\frac{25}{7}\)

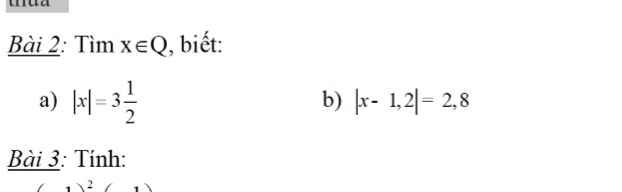
a) \(\left|x\right|=3\dfrac{1}{2}\)

\(\Rightarrow\left|x\right|=\dfrac{7}{2}\)

\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=-\dfrac{7}{2}\end{matrix}\right.\)

b) \(\left|x-1,2\right|=2,8\)

\(\Rightarrow\left[{}\begin{matrix}x-1,2=2,8\\x-1,2=-2,8\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}x=4\\x=-1,6\end{matrix}\right.\)

\(a,\left|x\right|=3\dfrac{1}{2}\)

\(\Rightarrow x=\left[{}\begin{matrix}3\dfrac{1}{2}\\-3\dfrac{1}{2}\end{matrix}\right.\)

\(b,\left|x-1,2\right|=2,8\\ \Rightarrow\left[{}\begin{matrix}x-1,2=2,8\\x-1,2=-2,8\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=2,8+1,2=4\\x=-2,8+1,2=-1,6\end{matrix}\right.\)

Vậy \(x\in\left\{4;-1,6\right\}\)

\(R1//R2\)

\(\Rightarrow\left\{{}\begin{matrix}Rtd=\dfrac{R1R2}{R1+R2}=8\Omega\\U2=U1=Ia1.R1=12V\Rightarrow Ia2=\dfrac{U2}{R2}=\dfrac{12}{40}=0,3A\\Ia=Ia1+Ia2=0,3+1,2=1,5A\\Uv=U2=12V\end{matrix}\right.\)

yêu cầu tính nhanh nha

Ta có: \(\dfrac{3}{n\left(n+3\right)}=\dfrac{\left(n+3\right)-n}{n\left(n+3\right)}=\dfrac{n+3}{n\left(n+3\right)}-\dfrac{n}{n\left(n+3\right)}=\dfrac{1}{n}-\dfrac{1}{n+3}\)

Do đó:

\(C=\dfrac{1}{1}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{7}+..+\dfrac{1}{37}-\dfrac{1}{40}\)

\(=1-\dfrac{1}{40}=\dfrac{39}{40}\)