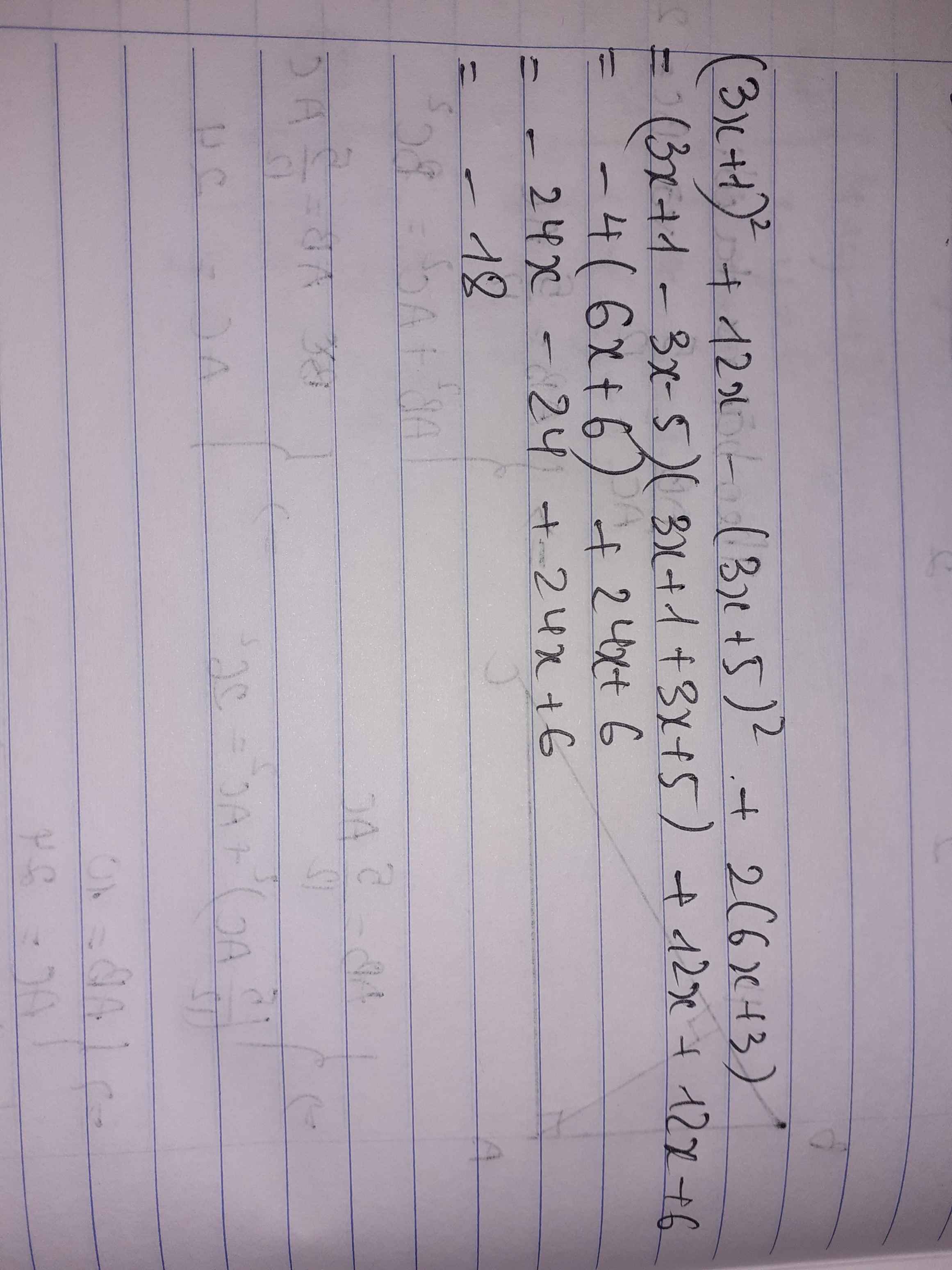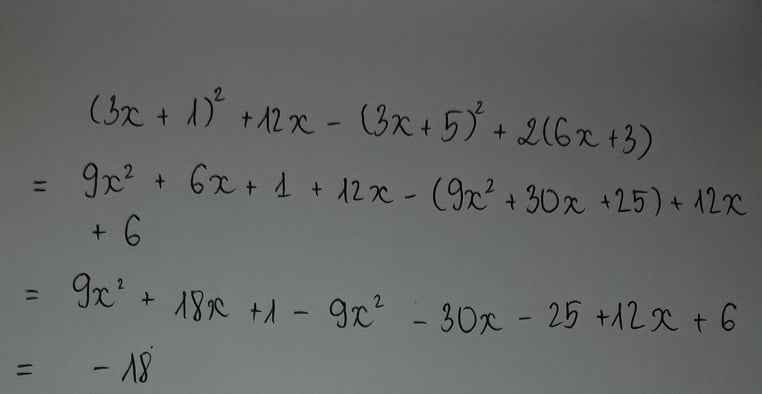tìm nghiệm nha mọi người quên chưa nói

a) \(x^2+2x+3=0\)

\(\Rightarrow x^2+2x+3-3=0-3\)

\(\Rightarrow x^2+2x=-3\)

\(\Rightarrow x^2+2x+1=-3+1\)

\(\Rightarrow\left(x+1\right)^2=-2\)

Điều này là vô lý vì bình phương của 1 số luôn lớn hơn hoặc bằng 0 mà -2 < 0.

Vậy đa thức vô nghiệm.

b) \(x^2+6x+5=0\)

\(x^2+x+5x+5=0\)

\(x\left(x+1\right)+5\left(x+1\right)=0\)

\(\left(x+5\right)\left(x+1\right)=0\)

\(\Rightarrow\orbr{\begin{cases}x+5=0\\x+1=0\end{cases}}\)

\(\Rightarrow\orbr{\begin{cases}x=-5\\x=-1\end{cases}}\)

Ta có: \(\left(3x+1\right)^2+12x-\left(3x+5\right)^2+2\left(6x+3\right)\)

\(=9x^2+6x+1+12x-9x^2-30x-25+12x+6\)

=-18

chuyển vế sang r phân tích thành nhân tử, có thể dùng máy tính bỏ túi nhé bạn

câu 1: 9\(x^2\) + 12\(x\) + 5  =11

           (3\(x\))² + 2.3.\(x\) .2 + 2² + 1 = 11

           (3\(x\) + 2)²      =  11 - 1

             (3\(x\) + 2)²    = 10

               \(\left[{}\begin{matrix}3x+2=\sqrt{10}\\3x+2=-\sqrt{10}\end{matrix}\right.\)

                \(\left[{}\begin{matrix}3x=\sqrt{10}-2\\3x=-\sqrt{10}-2\end{matrix}\right.\)

                  \(\left[{}\begin{matrix}x=\dfrac{\sqrt{10}-2}{3}\\x=\dfrac{-\sqrt{10}-2}{3}\end{matrix}\right.\)

                 Vậy S = {\(\dfrac{-\sqrt{10}-2}{3}\); \(\dfrac{\sqrt{10}-2}{3}\)} 

  Câu 2: 6\(x^2\) + 16\(x\) + 12 = 2\(x^2\)

              6\(x^2\) + 16\(x\) + 12 - 2\(x^2\) = 0

              4\(x^2\) + 16\(x\) + 12 = 0

              (2\(x\))² + 2.2.\(x\).4 + 16 - 4 = 0

               (2\(x\) + 4)²   = 4

               \(\left[{}\begin{matrix}2x+4=2\\2x+4=-2\end{matrix}\right.\) 

                \(\left[{}\begin{matrix}2x=-2\\2x=-6\end{matrix}\right.\)

                 \(\left[{}\begin{matrix}x=-1\\x=-3\end{matrix}\right.\)

              S = { -3; -1}

3, 16\(x^2\) + 22\(x\) + 11 = 6\(x\) + 5

    16\(x^2\) + 22\(x\) - 6\(x\)  + 11 - 5 = 0

     16\(x^2\) + 16\(x\) + 6 = 0

      (4\(x\))² + 2.4.\(x\) . 2 + 2² + 2 = 0

       (4\(x\) + 2)² + 2 = 0 (1) 

Vì (4\(x\)+ 2)² ≥ 0 ∀ ⇒ (4\(x\) + 2)² + 2 > 0 ∀ \(x\) vậy (1) Vô nghiệm

             S = \(\varnothing\)

Câu 4. 12\(x^2\) + 20\(x\) + 10 = 3\(x^2\) - 4\(x\) 

            12\(x^2\) + 20\(x\) + 10 - 3\(x^2\) + 4\(x\) = 0

            9\(x^2\) + 24\(x\) + 10 = 0

           (3\(x\))² + 2.3.\(x\).4 + 16 - 6 = 0

          (3\(x\) + 4)² = 6

            \(\left[{}\begin{matrix}3x+4=\sqrt{6}\\3x+4=-\sqrt{6}\end{matrix}\right.\)

              \(\left[{}\begin{matrix}3x=-4+\sqrt{6}\\3x=-4-\sqrt{6}\end{matrix}\right.\)

              \(\left[{}\begin{matrix}x=\dfrac{\sqrt{6}-4}{3}\\x=-\dfrac{\sqrt{6}+4}{3}\end{matrix}\right.\)

                    S = {\(\dfrac{-\sqrt{6}-4}{3}\); \(\dfrac{\sqrt{6}-4}{3}\)}

\(\left(3x+1\right)^2+12x-\left(3x+5\right)^2+2\left(6x+3\right)\)

\(=9x^2+6x+1+12x-9x^2-30x-25+12x+6\)

\(=-18\)

Vậy đa thức trên ko phụ thuộc vào biến 

a: ĐKXD: x<>0

\(\dfrac{14x^3+12x^2-14x}{2x}=\left(x+2\right)\left(3x-4\right)\)

=>\(\dfrac{2x\left(7x^2+6x-7\right)}{2x}=\left(x+2\right)\left(3x-4\right)\)

=>\(7x^2+6x-7=3x^2-4x+6x-8\)

=>\(7x^2+6x-7=3x^2+2x-8\)

=>\(4x^2+4x+1=0\)

=>\(\left(2x+1\right)^2=0\)

=>2x+1=0

=>x=-1/2(nhận)

b: \(\left(4x-5\right)\left(6x+1\right)-\left(8x+3\right)\left(3x-4\right)=15\)

=>\(24x^2+4x-30x-5-\left(24x^2-32x+9x-12\right)=15\)

=>\(24x^2-26x-5-24x^2+23x+12=15\)

=>-3x+7=15

=>-3x=8

=>\(x=-\dfrac{8}{3}\)

A = ( 3x + 1 ) ² + 12x - ( 3x + 5 ) ²+ 2( 6x + 3 )

=9x²+6x+1+12x-9x²-30x-25+12x+6

=(9x²-9x²)+(6x+12x-30x+12x)+1-25+6

=0+0+(-18)

=-18

A=(3x+1)²+12x-(3x+5)²+2(6x+3)

=9x²+6x+1+12x-(9x²+30x+25)+12x+6

=9x²+6x+1+12x-9x²-30x-25+12x+6

=60x-18

\(\Rightarrow\)đề sai 100%

\(A=9x^2+6x+1+12x-9x^2-30x-25+12x+6=0x-18=-18\)