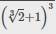 mn giải chi tiết giúp mình bước tách ra được ko ạ tại mình ko hiểu cách tách lắm. cảm ơn mn !
mn giải chi tiết giúp mình bước tách ra được ko ạ tại mình ko hiểu cách tách lắm. cảm ơn mn !

Những câu hỏi liên quan
(2+2)^2+?
Mn giúp mk với ạ, mình học lopw 8 nhưng mất gốc nên học hằng đẳng thức ko hiểu lắm. Giải chi tiết hộ mình nhé. Mình cảm ơn!
Trả lời :
\(\left(2+2\right)^2\)
\(=4^2\)
\(=16\)
~HT~
trả lời
(2+2)2
=4^2
=16
 Mn giúp có thể giúp mình câu C bài 4 và bài 5 được ko ạ, giải chi tiết 1 chút với ạ. Mình cảm ơn
Mn giúp có thể giúp mình câu C bài 4 và bài 5 được ko ạ, giải chi tiết 1 chút với ạ. Mình cảm ơn
Bài 4:
b: Xét ΔABK vuông tại A có AD là đường cao ứng với cạnh huyền BK
nên \(BD\cdot BK=BA^2\left(1\right)\)
Xét ΔABC vuông tại A có AH là đường cao ứng với cạnh huyền BC
nên \(BH\cdot BC=AB^2\left(2\right)\)
Từ (1) và (2) suy ra \(BD\cdot BK=BH\cdot BC\)
Đúng 1
Bình luận (1)
Tìm GTNN
E= 2x2+3x+8
được thì mn giải thích chi tiết chỗ phần tách ra giúp mình với ạ
E = 2x2 + 3x + 8
= 2( x2 + 3/2x + 9/16 ) + 55/8
= 2( x + 3/4 )2 + 55/8 ≥ 55/8 ∀ x
Dấu "=" xảy ra khi x = -3/4
=> MinE = 55/8 <=> x = -3/4
Giúp e bài 4 vs ạ(có vẽ hình),e cần cách lm dễ hiểu chi tiết,bài này e cần lắm luôn đó ạ nên giúp e lm đúng vs(nếu mn có thời gian thì giúp e luôn bài 2 vs,e ko đc chắc chắn bài này e lm có đúng ko)e cảm ơn nhìu lắm ạ!!!
Bài 2: Chọn C
Bài 4:
a: \(\widehat{C}=180^0-80^0-50^0=50^0\)
Xét ΔABC có \(\widehat{A}=\widehat{C}< \widehat{B}\)
nên BC=AB<AC
b: Xét ΔABC có AB<BC<AC
nên \(\widehat{C}< \widehat{A}< \widehat{B}\)
Đúng 1
Bình luận (0)
mn giải giúp mik vs ạ, giải chi tiết ra luôn, mình phải nộp trong hôm nay rùi ạ, cảm ơn mn nhìu
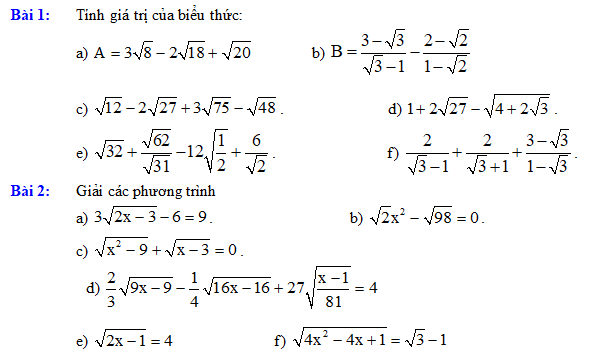

Bài 1:
\(a,A=6\sqrt{2}-6\sqrt{2}+2\sqrt{5}=2\sqrt{5}\\ b,B=\dfrac{\sqrt{3}\left(\sqrt{3}-1\right)}{\sqrt{3}-1}+\dfrac{\sqrt{2}\left(\sqrt{2}-1\right)}{\sqrt{2}-1}=\sqrt{3}+\sqrt{2}\\ c,=2\sqrt{3}-6\sqrt{3}+15\sqrt{3}-4\sqrt{3}=7\sqrt{3}\\ d,=1+6\sqrt{3}-\sqrt{3}-1=5\sqrt{3}\\ e,=4\sqrt{2}+\sqrt{2}-6\sqrt{2}+3\sqrt{2}=2\sqrt{2}\)
Bài 2:
\(a,ĐK:x\ge\dfrac{3}{2}\\ PT\Leftrightarrow\sqrt{2x-3}=5\Leftrightarrow2x-3=25\Leftrightarrow x=14\\ b,PT\Leftrightarrow x^2=\sqrt{\dfrac{98}{2}}=\sqrt{49}=7\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{7}\\x=-\sqrt{7}\end{matrix}\right.\\ c,ĐK:x\ge3\\ PT\Leftrightarrow\sqrt{x-3}\left(\sqrt{x+3}+1\right)=0\\ \Leftrightarrow\sqrt{x-3}=0\left(\sqrt{x+3}+1>0\right)\\ \Leftrightarrow x=3\\ d,ĐK:x\ge1\\ PT\Leftrightarrow2\sqrt{x-1}-\sqrt{x-1}+3\sqrt{x-1}=4\\ \Leftrightarrow\sqrt{x-1}=1\Leftrightarrow x=2\left(tm\right)\\ e,PT\Leftrightarrow2x-1=16\Leftrightarrow x=\dfrac{17}{2}\\ f,PT\Leftrightarrow\left|2x-1\right|=\sqrt{3}-1\Leftrightarrow\left[{}\begin{matrix}2x-1=\sqrt{3}-1\\2x-1=1-\sqrt{3}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\sqrt{3}}{2}\\x=\dfrac{2-\sqrt{3}}{2}\end{matrix}\right.\)
Đúng 2
Bình luận (0)
Bài 3:
\(a,Q=\dfrac{1+5}{3-1}=3\\ b,P=\dfrac{x+\sqrt{x}-6+x-2\sqrt{x}-3-x+4\sqrt{x}+9}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\\ P=\dfrac{\sqrt{x}\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}=\dfrac{\sqrt{x}}{\sqrt{x}-3}\\ c,M=\dfrac{\sqrt{x}}{\sqrt{x}-3}\cdot\dfrac{3-\sqrt{x}}{\sqrt{x}+5}=\dfrac{-\sqrt{x}}{\sqrt{x}+5}\)
Vì \(-\sqrt{x}\le0;\sqrt{x}+5>0\) nên \(M< 0\)
Do đó \(\left|M\right|>\dfrac{1}{2}\Leftrightarrow M< -\dfrac{1}{2}\Leftrightarrow-\dfrac{\sqrt{x}}{\sqrt{x}+5}+\dfrac{1}{2}< 0\)
\(\Leftrightarrow\dfrac{2\sqrt{x}-\sqrt{x}-5}{2\left(\sqrt{x}+5\right)}< 0\Leftrightarrow\sqrt{x}-5< 0\left(\sqrt{x}+5>0\right)\\ \Leftrightarrow0\le x< 25\)
Bài 4:
\(a,A=\dfrac{16+2\cdot4+5}{4-3}=29\\ b,B=\dfrac{2\sqrt{x}-9-x+9+2x-3\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\\ B=\dfrac{x-\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\sqrt{x}+1}{\sqrt{x}-3}\\ c,P=\dfrac{x+2\sqrt{x}+5}{\sqrt{x}-3}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}+1}=\dfrac{x+2\sqrt{x}+5}{\sqrt{x}+1}\\ P=\dfrac{\left(\sqrt{x}+1\right)^2+4}{\sqrt{x}+1}=\sqrt{x}+1+\dfrac{4}{\sqrt{x}+1}\\ P\ge2\sqrt{\left(\sqrt{x}+1\right)\cdot\dfrac{4}{\sqrt{x}+1}}=2\sqrt{4}=4\\ P_{min}=4\Leftrightarrow\left(\sqrt{x}+1\right)^2=4\Leftrightarrow\sqrt{x}+1=2\Leftrightarrow x=1\left(tm\right)\)
Đúng 1
Bình luận (0)
giúp mình với mn ai hiểu thì ghi chi tiết cho mình cũng đc! ạ cảm ơn mn nhiều
\(∘ backwin\)
\(CD:1 × 4 = 4 ( c m )\)
\(CR:1 × 3 = 3 ( c m )\)
\(Chiều\) \(cao:1 × 4 = 4 ( c m )\)
\(V: 4 × 3 × 4 = 48 ( c m ^3 )\)
\(Đ/s:48cm^3\)
Đúng 0
Bình luận (1)
Mn ơi giúp mình câu tự luận này được ko ạ? Hicc hicc mình cảm ơn nhiều lắm :((
Okie, xinh nên giúp :3 Đùa thui
a/ 5 nguồn mắc nối tiếp \(\left\{{}\begin{matrix}\xi_b=5.\xi=5.4=20\left(V\right)\\r_b=5r=5.0,2=1\left(\Omega\right)\end{matrix}\right.\)
b/ \(R_D=\dfrac{U^2_{dm}}{P_{dm}}=\dfrac{36}{6}=6\left(\Omega\right);I_{dm}=\dfrac{P_{dm}}{U_{dm}}=\dfrac{6}{6}=1\left(A\right)\)
Đèn sáng bình thường \(\Rightarrow I_2=I_D=I_{dm}=1\left(A\right)\)
\(\left(R_1ntR_B\right)//\left(R_2ntR_D\right)\Rightarrow R_{td}=\dfrac{\left(R_1+R_B\right)\left(R_2+R_D\right)}{R_1+R_B+R_2+R_D}=\dfrac{\left(2+4\right)\left(6+6\right)}{2+4+6+6}=4\left(\Omega\right)\)
c/ \(I=\dfrac{\xi_b}{r_b+R_{td}}=\dfrac{20}{1+4}=4\left(A\right)\)
\(I=I_1+I_2\Rightarrow I_1=I-I_2=4-1=3\left(A\right)\Rightarrow P_1=I_1^2.R_1=3^2.2=18\left(W\right)\)
\(m_{Cu}=\dfrac{A_{Cu}.I_B.t}{F.n}=\dfrac{64.3.\left(32.60+10\right)}{96500.2}=...\left(g\right)\)
Đúng 1
Bình luận (2)
Toán lớp 7 này e ko bt lm ạ,mà e đang cần,ai giúp e giải chi tiết dễ hiểu đc ko ạ,e cảm ơn nhiều lắm ạ!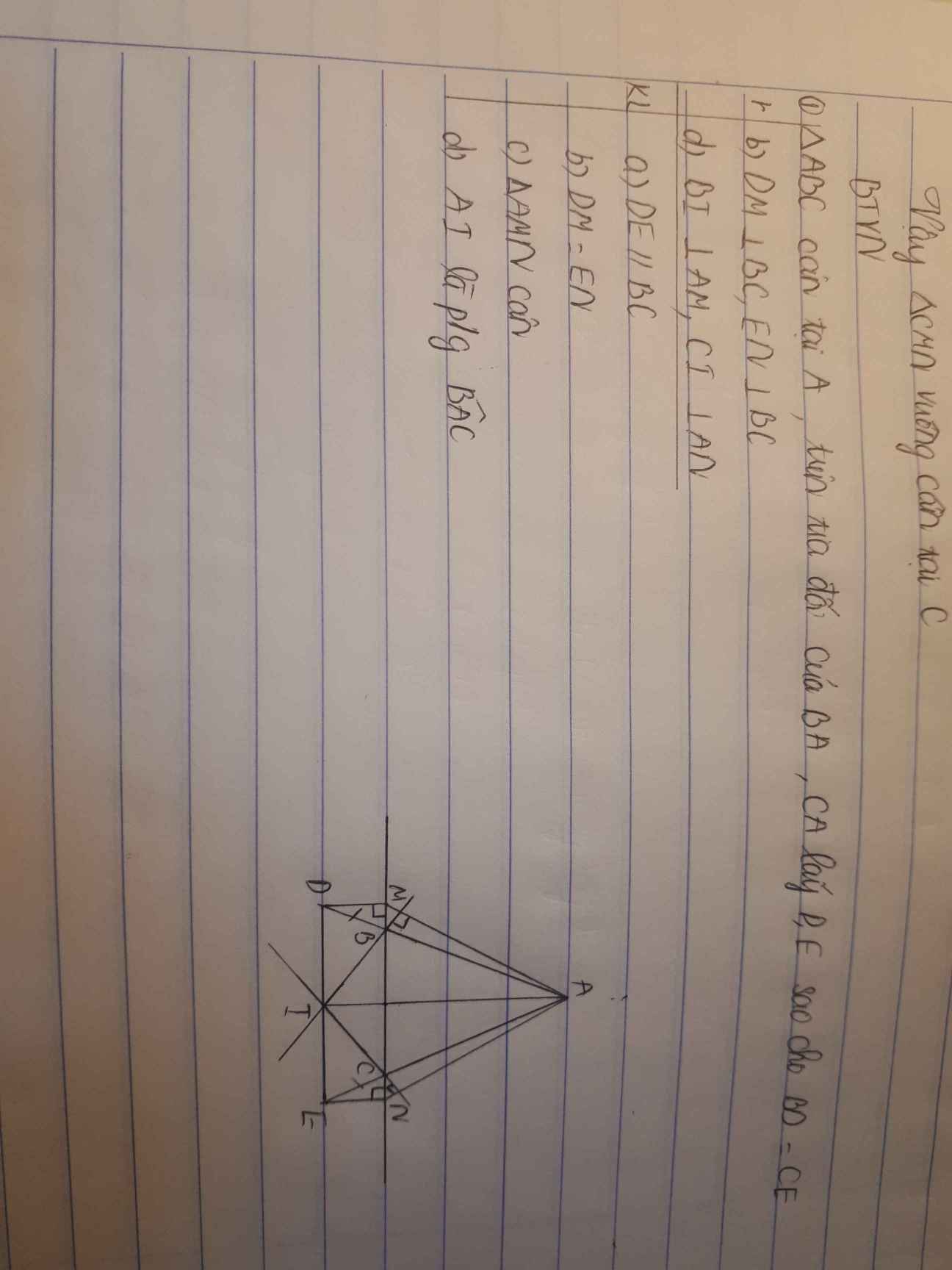
Mn giải chi tiết giúp mình với. Mình cảm ơn ạ
a: Khi m=2 thì (1) sẽ là:
2x+y=2 và 4x+3y=10
=>x=-2 và y=6
b: 2x+y=m và 4x+3y=10
=>4x+2y=2m và 4x+3y=10
=>4x+3y=10 và 4x+2y=2m
=>y=10-2m và 2x=m-10+2m=3m-10
=>y=10-2m và x=3/2m-5
x>0 và y>0
=>10-2m>0 và 3/2m-5>0
=>m>5:3/2=10/3 và m<5
=>10/3<m<5
Đúng 0
Bình luận (0)



















