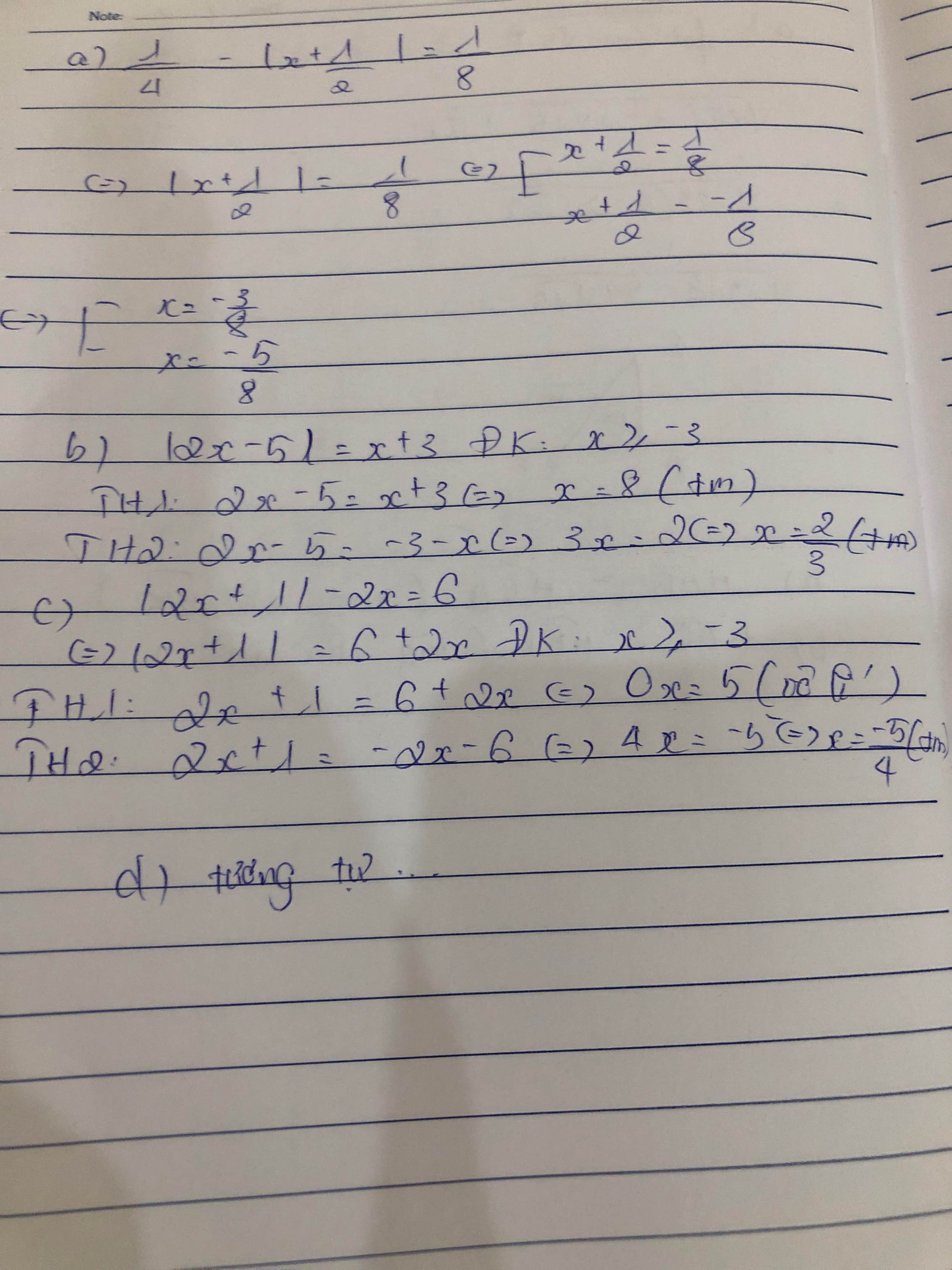a: ta có: \(\left(2x-5\right)\left(x+2\right)-2x\left(x-1\right)=15\)

\(\Leftrightarrow2x^2+4x-5x-10-2x^2+2x=15\)

\(\Leftrightarrow x=25\)

b: Ta có: \(\left(5-2x\right)\left(2x+7\right)=4x^2-25\)

\(\Leftrightarrow4x^2-25+\left(2x-5\right)\left(2x+7\right)=0\)

\(\Leftrightarrow\left(2x-5\right)\left(2x+5+2x+7\right)=0\)

\(\Leftrightarrow\left(2x-5\right)\left(x+3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-3\end{matrix}\right.\)

c: Ta có: \(x\left(4x-5\right)-\left(2x+1\right)^2=0\)

\(\Leftrightarrow4x^2-5x-4x^2-4x-1=0\)

\(\Leftrightarrow-9x=1\)

hay \(x=-\dfrac{1}{9}\)

a: ta có: $\left(2x-5\right)\left(x+2\right)-2x\left(x-1\right)=15$

$\iff 2{}^{}+4x-5x-10-2{}^{}+2x=15$

$\iff x=25$

b: Ta có: $\left(5-2x\right)\left(2x+7\right)=4{}^{}-25$

$\iff 4{}^{}-25+\left(2x-5\right)\left(2x+7\right)=0$

$\iff \left(2x-5\right)(2x+5+2x+$

a) Ta có: \(6x\left(x-5\right)+3x\left(7-2x\right)=18\)

\(\Leftrightarrow6x^2-30x+21x-6x^2=18\)

\(\Leftrightarrow-9x=18\)

hay x=-2

Vậy: S={-2}

b) Ta có: \(2x\left(3x+1\right)+\left(4-2x\right)\cdot3x=7\)

\(\Leftrightarrow6x^2+2x+12x-6x^2=7\)

\(\Leftrightarrow14x=7\)

hay \(x=\dfrac{1}{2}\)

Vậy: \(S=\left\{\dfrac{1}{2}\right\}\)

c) Ta có: \(0.5x\left(0.4-4x\right)+\left(2x+5\right)\cdot x=-6.5\)

\(\Leftrightarrow0.2x-2x^2+2x^2+5x=-6.5\)

\(\Leftrightarrow5.2x=-6.5\)

hay \(x=-\dfrac{5}{4}\)

Vậy: \(S=\left\{-\dfrac{5}{4}\right\}\)

d) Ta có: \(\left(x+3\right)\left(x+2\right)-\left(x-2\right)\left(x+5\right)=6\)

\(\Leftrightarrow x^2+5x+6-\left(x^2+3x-10\right)=6\)

\(\Leftrightarrow x^2+5x+6-x^2-3x+10=6\)

\(\Leftrightarrow2x+16=6\)

\(\Leftrightarrow2x=-10\)

hay x=-5

Vậy: S={-5}

e) Ta có: \(3\left(2x-1\right)\left(3x-1\right)-\left(2x-3\right)\left(9x-1\right)=0\)

\(\Leftrightarrow3\left(6x^2-5x+1\right)-\left(18x^2-29x+3\right)=0\)

\(\Leftrightarrow18x^2-15x+3-18x^2+29x-3=0\)

\(\Leftrightarrow14x=0\)

hay x=0

Vậy: S={0}

\(\frac{7^x\left(7^2+7+1\right)}{57}=\frac{5^{2x}\left(1+5+5^3\right)}{131}\)

\(\frac{7^x.57}{57}=\frac{5^{2x}.131}{131}\)

\(7^x=5^{2x}\)khi và chỉ khi x = 0.

\(\frac{7^{x+2}+7^{x+1}+7^x}{57}=\frac{7^x.7^2+7^x.7+7^x}{57}=\frac{7^x.\left(7^2+7+1\right)}{57}=7^x\)

\(\frac{5^{2x}+5^{2x+1}+5^{2x+3}}{131}=\frac{5^{2x}+5^{2x}.5+5^{2x}.5^3}{131}=\frac{5^{2x}\left(1+5+5^3\right)}{131}=\frac{25^x.131}{131}=25^x\)

\(\Rightarrow7^x=25^x\Rightarrow x=0\)

ai tích mình mình tích lại cho

ai tích mình mình tích lại cho

\(\frac{7^{x+2}+7^{x+1}+7^x}{57}=\frac{5^{2x}+5^{2x+1}+5^{2x+3}}{131}\)

\(\Rightarrow\frac{7^x.7^2+7^x.7^1+7^x}{57}=\frac{5^{2x}+5^{2x}.5+5^{2x}.5^3}{131}\)

\(\Rightarrow\frac{7^x.\left(7^2+7+1\right)}{57}=\frac{5^{2x}.\left(1+5+5^3\right)}{131}\)

\(\Rightarrow\frac{7^x.57}{57}=\frac{5^{2x}.131}{131}\)

\(\Rightarrow7^x=5^{2x}\)

Bạn tự làm phần còn lại nhé

Biến đổi vế trái, ta được : \(\frac{7^{x+2}+7^{x+1}+7^x}{57}=\frac{7^x.7^2+7^x.7+7^x}{57}=\frac{7^x\left(7^2+7+1\right)}{57}=\frac{7^x.57}{57}=7^x\)\(=7^x\)

Biến đổi vế phải, ta được : \(\frac{5^{2x}+5^{2x+1}+5^{2x+3}}{131}=\frac{5^{2x}+5^{2x}.5+5^{2x}.5^3}{131}=\frac{5^{2x}.\left(1+5+5^3\right)}{131}=\frac{5^{2x}.131}{131}=5^{2x}=25^x\)

\(\Rightarrow7^x=25^x\)

Vì \(\left(7,25\right)=1\)

\(\Rightarrow7^x=25^x=1\)

\(\Rightarrow x=0\)

Vậy \(x=0\)

a) Ta có: \(\dfrac{1}{4}-\left|x+\dfrac{1}{2}\right|=\dfrac{1}{8}\)

\(\Leftrightarrow\left|x+\dfrac{1}{2}\right|=\dfrac{1}{8}\)

\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{1}{2}=\dfrac{1}{8}\\x+\dfrac{1}{2}=-\dfrac{1}{8}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-3}{8}\\x=\dfrac{-5}{8}\end{matrix}\right.\)

b) Ta có: \(\left|2x-5\right|=x+3\)

\(\Leftrightarrow\left[{}\begin{matrix}2x-5=x+3\left(x\ge\dfrac{5}{2}\right)\\2x-5=-x-3\left(x< \dfrac{5}{2}\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x-x=3+5\\2x+x=-3+5\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=8\left(nhận\right)\\x=\dfrac{2}{3}\left(nhận\right)\end{matrix}\right.\)