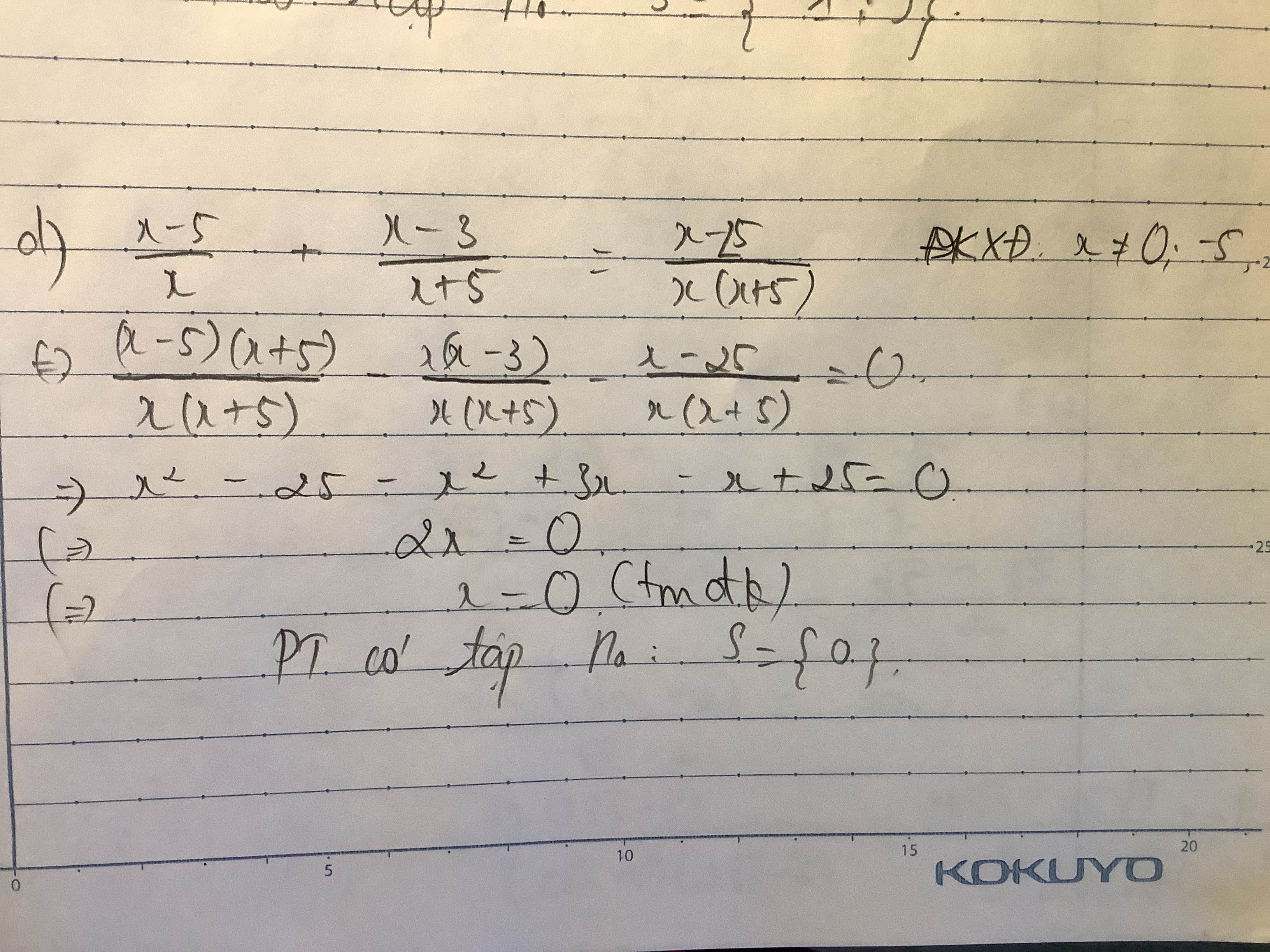1, \(\left(\dfrac{1}{3}-x\right)^2=\dfrac{9}{25}\)
2, \(\left(5-x\right)^2=25\)

Những câu hỏi liên quan
Tìm x liên quan đến lũy thừa:
1, \(\left(3x-\dfrac{1}{5}\right)^2=\left(\dfrac{-3}{25}\right)^2\)
2, \(\left(2x-\dfrac{1}{3}\right)^2=\left(\dfrac{-2}{9}\right)^2\)
3, \(\left(\dfrac{1}{3}-x\right)^2=\dfrac{9}{25}\)
4, \(\left(5-x\right)^2=25\)
1: \(\left(3x-\dfrac{1}{5}\right)^2=\left(-\dfrac{3}{25}\right)^2\)
=>3x-1/5=3/25 hoặc 3x-1/5=-3/25
=>3x=8/25 hoặc 3x=2/25
=>x=8/75 hoặc x=2/75
2: \(\left(2x-\dfrac{1}{3}\right)^2=\left(-\dfrac{2}{9}\right)^2\)
=>2x-1/3=2/9 hoặc 2x-1/3=-2/9
=>2x=5/9 hoặc 2x=1/9
=>x=5/18 hoặc x=1/18
Đúng 2
Bình luận (0)
Câu 1: Thực hiện phép tính
a, \(40\dfrac{1}{4}:\dfrac{5}{7}-25\dfrac{1}{4}:\dfrac{5}{7}-\dfrac{1}{2021}\)
b, \(\left|\dfrac{-5}{9}\right|.\sqrt{81}-2021^0.\dfrac{16}{25}\)
Câu 2: Tìm x
\(3\left(x-\dfrac{1}{3}\right)-7\left(x+\dfrac{3}{7}\right)=-2x+\dfrac{1}{3}\)
1:
a: =7/5(40+1/4-25-1/4)-1/2021
=21-1/2021=42440/2021
b: =5/9*9-1*16/25=5-16/25=109/25
Đúng 0
Bình luận (0)
giải các phương trình sau
a, 4x- 2(1-x)= 5(x-4)
b, \(\dfrac{x}{6}+\dfrac{1-3x}{9}=\dfrac{-x+1}{12}\)
c, \(\left(x+2\right)^2-3\left(x+2\right)=0\)
d,\(\dfrac{x-5}{x}+\dfrac{x-3}{x+5}=\dfrac{x-25}{x\left(x+5\right)}\)
a: Ta có: \(4x-2\left(1-x\right)=5\left(x-4\right)\)
\(\Leftrightarrow4x-2+2x=5x-20\)
\(\Leftrightarrow x=-18\)
b: Ta có: \(\dfrac{x}{6}+\dfrac{1-3x}{9}=\dfrac{-x+1}{12}\)
\(\Leftrightarrow6x+4\left(1-3x\right)=3\left(-x+1\right)\)
\(\Leftrightarrow6x+4-12x=-3x+3\)
\(\Leftrightarrow-3x=-1\)
hay \(x=\dfrac{1}{3}\)
c: Ta có: \(\left(x+2\right)^2-3\left(x+2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\)
Đúng 0
Bình luận (0)
d: Ta có: \(\dfrac{x-5}{x}+\dfrac{x-3}{x+5}=\dfrac{x-25}{x\left(x+5\right)}\)
\(\Leftrightarrow x^2-25+x^2-3x=x-25\)
\(\Leftrightarrow2x^2-4x=0\)
\(\Leftrightarrow2x\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(loại\right)\\x=2\left(nhận\right)\end{matrix}\right.\)
Đúng 0
Bình luận (0)
tìm x
\(\dfrac{3-x}{5-x}=\dfrac{6}{11}\) \(\left(1\dfrac{1}{3}-25\%.x-\dfrac{5}{12}\right)-2x=1,6:\dfrac{3}{5}\)
\(\dfrac{1}{2}.\left(x-\dfrac{2}{3}\right)-\dfrac{1}{3}.\left(2x-3\right)=x\)
\(2.\left(\dfrac{1}{2}-x\right)-3\left(x-\dfrac{1}{3}\right)=\dfrac{7}{2}\)
a: =>11(x-3)=6(x-5)
=>11x-33=6x-30
=>5x=3
=>x=3/5
b: =>(4/3-1/4x-5/12)-2x=8/5*5/3=8/3
=>-9/4x+11/12=8/3
=>-9/4x=32/12-11/12=21/12=7/4
=>x=-7/9
c: =>1/2x-1/3-2/3x-1=x
=>-1/6x-4/3=x
=>-7/6x=4/3
=>x=-4/3:7/6=-4/3*6/7=-24/21=-8/7
d: =>1-2x-3x+1=7/2
=>-5x=3/2
=>x=-3/10
Đúng 0
Bình luận (0)
Giải PT:a) dfrac{1}{2}sqrt{x-1}-dfrac{3}{2}sqrt{9x-9}+24sqrt{dfrac{x-1}{64}}-17b) sqrt{18x-9}-0,5sqrt{2x-1}+dfrac{1}{2}sqrt{25left(2x-1right)}+sqrt{49left(2x-1right)}24c) sqrt{36x-72}-15sqrt{dfrac{x-2}{25}}4left(5+sqrt{x-2}right)d) sqrt{dfrac{1}{3x+2}}-dfrac{1}{2}sqrt{dfrac{9}{3x+2}}+sqrt{dfrac{16}{3x+2}}-5sqrt{dfrac{1}{12x+8}}1e) dfrac{1}{2}sqrt{dfrac{49x}{x+2}}-3sqrt{dfrac{x}{4x+8}}-sqrt{dfrac{x}{x+2}}-sqrt{5}0
Đọc tiếp
Giải PT:
a) \(\dfrac{1}{2}\sqrt{x-1}-\dfrac{3}{2}\sqrt{9x-9}+24\sqrt{\dfrac{x-1}{64}}=-17\)
b) \(\sqrt{18x-9}-0,5\sqrt{2x-1}+\dfrac{1}{2}\sqrt{25\left(2x-1\right)}+\sqrt{49\left(2x-1\right)}=24\)
c) \(\sqrt{36x-72}-15\sqrt{\dfrac{x-2}{25}}=4\left(5+\sqrt{x-2}\right)\)
d) \(\sqrt{\dfrac{1}{3x+2}}-\dfrac{1}{2}\sqrt{\dfrac{9}{3x+2}}+\sqrt{\dfrac{16}{3x+2}}-5\sqrt{\dfrac{1}{12x+8}}=1\)
e) \(\dfrac{1}{2}\sqrt{\dfrac{49x}{x+2}}-3\sqrt{\dfrac{x}{4x+8}}-\sqrt{\dfrac{x}{x+2}}-\sqrt{5}=0\)
a. ĐKXĐ: $x\geq 1$
PT $\Leftrightarrow \frac{1}{2}\sqrt{x-1}-\frac{3}{2}.\sqrt{9}.\sqrt{x-1}+24.\sqrt{\frac{1}{64}}.\sqrt{x-1}=-17$
$\Leftrightarrow \frac{1}{2}\sqrt{x-1}-\frac{9}{2}\sqrt{x-1}+3\sqrt{x-1}=-17$
$\Leftrightarrow -\sqrt{x-1}=-17$
$\Leftrightarrow \sqrt{x-1}=17$
$\Leftrightarrow x-1=289$
$\Leftrightarrow x=290$
b. ĐKXĐ: $x\geq \frac{1}{2}$
PT $\Leftrightarrow \sqrt{9}.\sqrt{2x-1}-0,5\sqrt{2x-1}+\frac{1}{2}.\sqrt{25}.\sqrt{2x-1}+\sqrt{49}.\sqrt{2x-1}=24$
$\Leftrightarrow 3\sqrt{2x-1}-0,5\sqrt{2x-1}+2,5\sqrt{2x-1}+7\sqrt{2x-1}=24$
$\Leftrightarrow 12\sqrt{2x-1}=24$
$\Leftrihgtarrow \sqrt{2x-1}=2$
$\Leftrightarrow x=2,5$ (tm)
Đúng 1
Bình luận (0)
c. ĐKXĐ: $x\geq 2$
PT $\Leftrightarrow \sqrt{36}.\sqrt{x-2}-15\sqrt{\frac{1}{25}}\sqrt{x-2}=4(5+\sqrt{x-2})$
$\Leftrightarrow 6\sqrt{x-2}-3\sqrt{x-2}=20+4\sqrt{x-2}$
$\Leftrightarrow \sqrt{x-2}=-20< 0$ (vô lý)
Vậy pt vô nghiệm
Đúng 0
Bình luận (0)
d. ĐKXĐ: $x>\frac{-2}{3}$
PT $\Leftrightarrow \sqrt{\frac{1}{3x+2}}-\frac{1}{2}\sqrt{9}.\sqrt{\frac{1}{3x+2}}+\sqrt{16}.\sqrt{\frac{1}{3x+2}}-5\sqrt{\frac{1}{4}}\sqrt{\frac{1}{3x+2}}=1$
$\Leftrightarrow \sqrt{\frac{1}{3x+2}}-\frac{3}{2}\sqrt{\frac{1}{3x+2}}+4\sqrt{\frac{1}{3x+2}}-\frac{5}{2}\sqrt{\frac{1}{3x+2}}=1$
$\Leftrightarrow \sqrt{\frac{1}{3x+2}}=1$
$\Leftrightarrow \frac{1}{3x+2}=1$
$\Leftrightarrow 3x+2=1$
$\Leftrightarrow x=-\frac{1}{3}$
Đúng 0
Bình luận (0)
Rút gọn:1) Aleft(dfrac{x-5sqrt{x}}{x-25}-1right):left(dfrac{25-x}{x+2sqrt{x}-15}-dfrac{sqrt{x}+3}{sqrt{x}+5}-dfrac{sqrt{x}-5}{sqrt{x}-3}right)2) Aleft(dfrac{1}{sqrt{a}-1}-dfrac{1}{sqrt{a}}right):left(dfrac{sqrt{a}+1}{sqrt{a}-2}-dfrac{sqrt{a}+2}{sqrt{a}-1}right)3) Aleft(dfrac{sqrt{x}+2}{x+2sqrt{x}+1}-dfrac{sqrt{x}-2}{x-1}right).dfrac{sqrt{x}+1}{sqrt{x}}4) Aleft(dfrac{x+1}{x-1}-dfrac{x-1}{x+1}+dfrac{x^2-4x-1}{x^2-1}right).dfrac{x+2003}{x}5) Aleft(dfrac{5sqrt{x}}{x-4}-dfrac{sqrt{x}}{sqrt{x}-2}+dfra...
Đọc tiếp
Rút gọn:
1) \(A=\left(\dfrac{x-5\sqrt{x}}{x-25}-1\right):\left(\dfrac{25-x}{x+2\sqrt{x}-15}-\dfrac{\sqrt{x}+3}{\sqrt{x}+5}-\dfrac{\sqrt{x}-5}{\sqrt{x}-3}\right)\)
2) \(A=\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\left(\dfrac{\sqrt{a}+1}{\sqrt{a}-2}-\dfrac{\sqrt{a}+2}{\sqrt{a}-1}\right)\)
3) \(A=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right).\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
4) \(A=\left(\dfrac{x+1}{x-1}-\dfrac{x-1}{x+1}+\dfrac{x^2-4x-1}{x^2-1}\right).\dfrac{x+2003}{x}\)
5) \(A=\left(\dfrac{5\sqrt{x}}{x-4}-\dfrac{\sqrt{x}}{\sqrt{x}-2}+\dfrac{\sqrt{x}}{\sqrt{x}+2}\right)\left(2-\sqrt{x}\right)\)
6) \(A=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right).\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
Giúp mình với, cần gấp ạ ![]()
2: Ta có: \(A=\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\left(\dfrac{\sqrt{a}+1}{\sqrt{a}-2}-\dfrac{\sqrt{a}+2}{\sqrt{a}-1}\right)\)
\(=\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}:\dfrac{a-1-a+4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}\)
\(=\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}{3}\)
\(=\dfrac{\sqrt{a}-2}{3\sqrt{a}}\)
Đúng 1
Bình luận (1)
1: Ta có: \(A=\left(\dfrac{x-5\sqrt{x}}{x-25}-1\right):\left(\dfrac{25-x}{x+2\sqrt{x}-15}-\dfrac{\sqrt{x}+3}{\sqrt{x}+5}-\dfrac{\sqrt{x}-5}{\sqrt{x}-3}\right)\)
\(=\left(\dfrac{x-5\sqrt{x}-x+25}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\right):\dfrac{25-x-x+9-x+25}{\left(\sqrt{x}+5\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{-5}{\sqrt{x}+5}\cdot\dfrac{\left(\sqrt{x}+5\right)\left(\sqrt{x}-3\right)}{-3x+59}\)
\(=\dfrac{-5\left(\sqrt{x}-3\right)}{-3x+59}\)
\(=\dfrac{5\sqrt{x}-15}{3x-59}\)
Đúng 1
Bình luận (0)
Xem thêm câu trả lời
Rút gọn:1) Aleft(dfrac{x-5sqrt{x}}{x-25}-1right):left(dfrac{25-x}{x+2sqrt{x}-15}-dfrac{sqrt{x}+3}{sqrt{x}+5}+dfrac{sqrt{x}-5}{sqrt{x}-3}right)2) Aleft(dfrac{1}{sqrt{a}-1}-dfrac{1}{sqrt{a}}right):left(dfrac{sqrt{a}+1}{sqrt{a}-2}-dfrac{sqrt{a}+2}{sqrt{a}-1}right)3) Aleft(dfrac{x+1}{x-1}-dfrac{x-1}{x+1}+dfrac{x^2-4x-1}{x^2-1}right).dfrac{x+2003}{x}4) Aleft(dfrac{5sqrt{x}}{x-4}-dfrac{sqrt{x}}{sqrt{x}-2}+dfrac{sqrt{x}}{sqrt{x}+2}right)left(2-sqrt{x}right)5) Aleft(dfrac{sqrt{x}+2}{x+2sqrt{x}+1}-dfrac{...
Đọc tiếp
Rút gọn:
1) \(A=\left(\dfrac{x-5\sqrt{x}}{x-25}-1\right):\left(\dfrac{25-x}{x+2\sqrt{x}-15}-\dfrac{\sqrt{x}+3}{\sqrt{x}+5}+\dfrac{\sqrt{x}-5}{\sqrt{x}-3}\right)\)
2) \(A=\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\left(\dfrac{\sqrt{a}+1}{\sqrt{a}-2}-\dfrac{\sqrt{a}+2}{\sqrt{a}-1}\right)\)
3) \(A=\left(\dfrac{x+1}{x-1}-\dfrac{x-1}{x+1}+\dfrac{x^2-4x-1}{x^2-1}\right).\dfrac{x+2003}{x}\)
4) \(A=\left(\dfrac{5\sqrt{x}}{x-4}-\dfrac{\sqrt{x}}{\sqrt{x}-2}+\dfrac{\sqrt{x}}{\sqrt{x}+2}\right)\left(2-\sqrt{x}\right)\)
5) \(A=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right).\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
Giúp vs ạ ![]()
1: Ta có: \(A=\left(\dfrac{x-5\sqrt{x}}{x-25}-1\right):\left(\dfrac{25-x}{x+2\sqrt{x}-15}-\dfrac{\sqrt{x}+3}{\sqrt{x}+5}-\dfrac{\sqrt{x}-5}{\sqrt{x}-3}\right)\)
\(=\left(\dfrac{x-5\sqrt{x}-x+25}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\right):\dfrac{25-x-x+9-x+25}{\left(\sqrt{x}+5\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{-5}{\sqrt{x}+5}\cdot\dfrac{\left(\sqrt{x}+5\right)\left(\sqrt{x}-3\right)}{-3x+59}\)
\(=\dfrac{-5\left(\sqrt{x}-3\right)}{-3x+59}\)
\(=\dfrac{5\sqrt{x}-15}{3x-59}\)
Đúng 1
Bình luận (0)
2: Ta có: \(A=\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\left(\dfrac{\sqrt{a}+1}{\sqrt{a}-2}-\dfrac{\sqrt{a}+2}{\sqrt{a}-1}\right)\)
\(=\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}:\dfrac{a-1-a+4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}\)
\(=\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}{3}\)
\(=\dfrac{\sqrt{a}-2}{3\sqrt{a}}\)
Đúng 1
Bình luận (1)
3: Ta có: \(A=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right)\cdot\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
\(=\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)-\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+1\right)^2\cdot\left(\sqrt{x}-1\right)}\cdot\dfrac{\sqrt{x}+1}{\sqrt{x}}\)
\(=\dfrac{x+\sqrt{x}-2-x+\sqrt{x}+2}{x-1}\cdot\dfrac{1}{\sqrt{x}}\)
\(=\dfrac{2}{x-1}\)
Đúng 1
Bình luận (0)
Xem thêm câu trả lời
Phân tích đa thức \(18x^3-\dfrac{8}{25}x\) thành nhân tử
a. \(\dfrac{2}{25}x\left(9x^2-4\right)=\dfrac{2}{25}x\left(3x-2\right)\left(3x+2\right)\)
b. \(2x\left(9x^2-\dfrac{4}{25}\right)=2x\left(3x-\dfrac{2}{5}\right)\left(3x+\dfrac{2}{5}\right)\)
Cách phân tích nào đúng, a hay b. Giải thích vì sao?
1/ (left(-dfrac{2}{3}right)^2 x dfrac{-9}{8} - 25% x dfrac{-16}{5} 2/ -1dfrac{2}{5} x 75% + dfrac{-7}{5} x 25% 3/ -2dfrac{3}{7} x (-125%) + dfrac{-17}{7} x 25% 4/ (-2)^3 x (dfrac{3}{4} x 0.25) : (2dfrac{1}{4} - 1dfrac{1}{6})
Đọc tiếp
1/ (\(\left(-\dfrac{2}{3}\right)\)\(^2\) x \(\dfrac{-9}{8}\) - 25% x \(\dfrac{-16}{5}\)
2/ -1\(\dfrac{2}{5}\) x 75% + \(\dfrac{-7}{5}\) x 25%
3/ -2\(\dfrac{3}{7}\) x (-125%) + \(\dfrac{-17}{7}\) x 25%
4/ (-2)\(^3\) x (\(\dfrac{3}{4}\) x 0.25) : (2\(\dfrac{1}{4}\) - 1\(\dfrac{1}{6}\))
1) Ta có: \(\left(-\dfrac{2}{3}\right)^2\cdot\dfrac{-9}{8}-25\%\cdot\dfrac{-16}{5}\)
\(=\dfrac{4}{9}\cdot\dfrac{-9}{8}-\dfrac{1}{4}\cdot\dfrac{-16}{5}\)
\(=\dfrac{-1}{2}+\dfrac{4}{5}\)
\(=\dfrac{-5}{10}+\dfrac{8}{10}=\dfrac{3}{10}\)
2) Ta có: \(-1\dfrac{2}{5}\cdot75\%+\dfrac{-7}{5}\cdot25\%\)
\(=\dfrac{-7}{5}\cdot\dfrac{3}{4}+\dfrac{-7}{5}\cdot\dfrac{1}{4}\)
\(=\dfrac{-7}{5}\left(\dfrac{3}{4}+\dfrac{1}{4}\right)=-\dfrac{7}{5}\)
3) Ta có: \(-2\dfrac{3}{7}\cdot\left(-125\%\right)+\dfrac{-17}{7}\cdot25\%\)
\(=\dfrac{-17}{7}\cdot\dfrac{-5}{4}+\dfrac{-17}{7}\cdot\dfrac{1}{4}\)
\(=\dfrac{-17}{7}\cdot\left(\dfrac{-5}{4}+\dfrac{1}{4}\right)\)
\(=\dfrac{17}{7}\)
4) Ta có: \(\left(-2\right)^3\cdot\left(\dfrac{3}{4}\cdot0.25\right):\left(2\dfrac{1}{4}-1\dfrac{1}{6}\right)\)
\(=\left(-8\right)\cdot\left(\dfrac{3}{4}\cdot\dfrac{1}{4}\right):\left(\dfrac{9}{4}-\dfrac{7}{6}\right)\)
\(=\left(-8\right)\cdot\dfrac{3}{16}:\dfrac{54-28}{24}\)
\(=\dfrac{-3}{2}\cdot\dfrac{24}{26}\)
\(=\dfrac{-72}{52}=\dfrac{-18}{13}\)
Đúng 1
Bình luận (0)