2021/2018 x 2022 ... ... ... 2019/2020 x 2020

Những câu hỏi liên quan
2021/2022 + 2020/2021 + 2019/2020 + 2018/2019 + 2017/2018 rôì so sánh với 5
A = 2021/2022+2020/2021+2019/2020+2018/2019+2017/2018
A<2022/2022+2021/2021+2020/2020+2019/2019+2018/2018
A<1+1+1+1+1
A<5
Đúng 0
Bình luận (0)
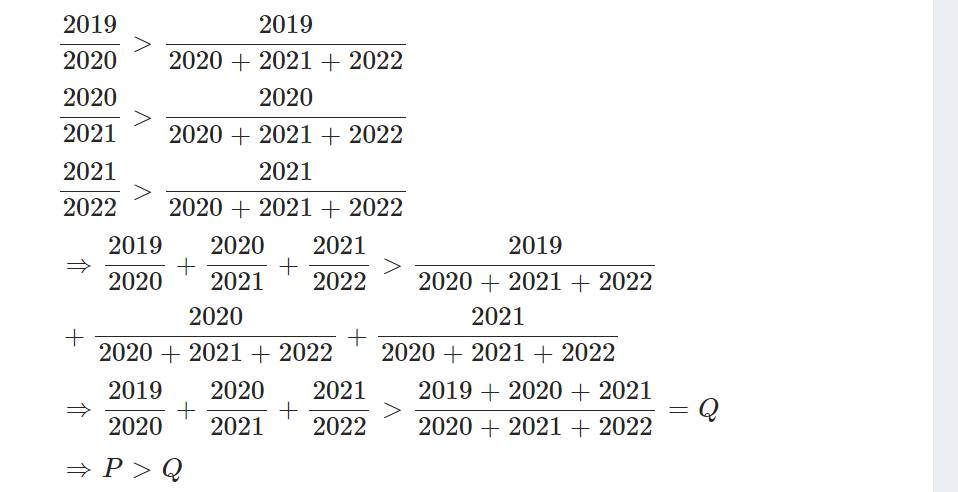
so sánh P=2019/2020+2020/2021+2021/2022 và Q=2019+2020+2021/2020+2021+2022
Tính
(1-1/2018) x (1-1/2019) x (1-1/2020) x (1-1/2021) x (1-1/2022)
\(\left(1-\frac{1}{2018}\right)\times\left(1-\frac{1}{2019}\right)\times\left(1-\frac{1}{2020}\right)\times\left(1-\frac{1}{2021}\right)\times\left(1-\frac{1}{2022}\right)\)
\(=\frac{2017}{2018}\times\frac{2018}{2019}\times\frac{2019}{2020}\times\frac{2020}{2021}\times\frac{2021}{2022}\)
\(=\frac{2017}{2022}\)
1-2-3+4+5-6-7+8+...+2018-2019-2020+2021+2022-2023
Sửa đề: 1-2-3+4+5-6-7+8+...-2018-2019+2020+2021-2022-2023
=(1-2-3+4)+(5-6-7+8)+...+(2017-2018-2019+2020)+(2021-2022-2023)
=0+0+...+0+(-1-2023)
=-2024
Đúng 0
Bình luận (0)
tính
2011 + 2012 + 2013 + 2014 + 2015 + 2016 + 2017 + 2018 + 2019+ 2020 + 2021 +2022 +2023
2011+2012+2013+2014+2015+2016+2017+2018+2019+2020+2021+ 2022+2023 =(2011+2023)+(2013+2022)+...+(2016+2018)+2017 =4034+4034+4034+4034+4034+4034+2017 =4034x6+2017=26221
Đúng 0
Bình luận (0)
2011+2012+2013+2014+2015+2016+2017+2018+2019+2020+2021+2022+2023
=(2011+2023)+(2013+2022)+...+(2016+2018)+2017 =4034+4034+4034+4034+4034+4034+2017 =4034x6+2017=26221
Đúng 0
Bình luận (0)
Cho x = 2020, tính giá trị:
P(x) = x^2021-2021x^2020+2021x^2019-2021x^2018+...+2021x-2020
x=2020 nên x+1=2021
\(P\left(x\right)=x^{2021}-x^{2020}\left(x+1\right)+x^{2019}\left(x+1\right)-....+x\left(x+1\right)-2020\)
\(=x^{2021}-x^{2021}-x^{2020}+x^{2020}-...+x^2+x-2020\)
=x-2020=0
Đúng 0
Bình luận (0)
Ta có: fleft(2019right)20202019+1 fleft(2020right)20212020+1Đặt hleft(xright)-x-1và gleft(xright)fleft(xright)+hleft(xright)Rightarrowhept{begin{cases}gleft(2019right)fleft(2019right)+hleft(2019right)2020-20200gleft(2020right)fleft(2020right)+hleft(2020right)2021-20210end{cases}}Rightarrow x2019;x2020là nghiệm của đa thức g(x) mà g(x) là đa thức bậc 3 , hệ số x^3là số nguyênRightarrow gleft(xright)aleft(x-2019right)left(x-2020right)left(x-x_0right)(ainZ*)Rightarrow fleft(...
Đọc tiếp
Ta có: \(f\left(2019\right)=2020=2019+1\)
\(f\left(2020\right)=2021=2020+1\)
Đặt \(h\left(x\right)=-x-1\)và \(g\left(x\right)=f\left(x\right)+h\left(x\right)\)
\(\Rightarrow\hept{\begin{cases}g\left(2019\right)=f\left(2019\right)+h\left(2019\right)=2020-2020=0\\g\left(2020\right)=f\left(2020\right)+h\left(2020\right)=2021-2021=0\end{cases}}\)
\(\Rightarrow x=2019;x=2020\)là nghiệm của đa thức g(x) mà g(x) là đa thức bậc 3 , hệ số \(x^3\)là số nguyên
\(\Rightarrow g\left(x\right)=a\left(x-2019\right)\left(x-2020\right)\left(x-x_0\right)\)(\(a\in\)Z*)
\(\Rightarrow f\left(x\right)=g\left(x\right)-h\left(x\right)\)
\(=a\left(x-2019\right)\left(x-2020\right)\left(x-x_0\right)+x+1\)
\(f\left(2021\right)=a\left(2021-2019\right)\left(2021-2020\right)\left(2021-x_0\right)+2021+1\)
\(=a.1.2\left(2021-x_0\right)+2022\)
\(f\left(2018\right)=a\left(2018-2019\right)\left(2018-2020\right)\left(2018-x_0\right)+2018+1\)
\(=a.1.2.\left(2018-x_0\right)+2019\)
\(\Rightarrow f\left(2021\right)-f\left(2018\right)=a.1.2\left(2021-2018\right)+3\)
\(=6a+3\)
Làm nốt
Cho đa thức \(f\left(x\right)\)bậc 3 với hệ số \(x^3\)là số nguyên dương thỏa mãn:
\(f\left(2019\right)=2020;f\left(2020\right)=2021\)
CMR \(f\left(2021\right)-f\left(2018\right)\)là hợp số
tính :
A= 1+2-3-4+5+6-7-8+9+...+2018-2019-2020+2021-2022
A = 1 + 2 - 3 - 4 + 5 + 6 - 7 - 8 + ... + 2018 – 2019 - 2020 + 2021
A = (1 + 2 - 3 - 4) + ... + (2017 + 2018 – 2019 - 2020) + 2021
A = (-4) + ... + (-4) + 2021 +
2020 : 4 = 505
A = (-4) . 505 + 2021
A = (-2020) + 2021
A = 1
Vậy A=1
Mình gửi bạn nha !!!!!
Đúng 0
Bình luận (0)
Bài 3: Không quy đồng hãy so sánh các phân số sau: a, 2019/2020 và 2021/2022 b, 2019/2017 và 2021/2019 c, 201/202 và 135/137 d, 2019/2018 và 2021/2019