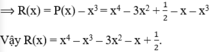Tìm x để biểu thức sau có GTNN
A = x4 + 3x2 + 2
Những câu hỏi liên quan
Cho biểu thức sau :B[(x4−x+x−3x3+1).(x3−2x2+2x−1)(x+1)x9+x7−3x2−3+1−2(x+6)x2+1].4x2+4x+1(x+3)(4−x)[(x4−x+x−3x3+1).(x3−2x2+2x−1)(x+1)x9+x7−3x2−3+1−2(x+6)x2+1].4x2+4x+1(x+3)(4−x)a, Tìm giá trị của x để giá trị của biểu thức B được xác địnhb, Rút gọn Bc, Cmr với các giá trị của x mà giá trị của biểu thức xác định thì −5≤B≤0
Đọc tiếp
Cho biểu thức sau :
B=[(x4−x+x−3x3+1).(x3−2x2+2x−1)(x+1)x9+x7−3x2−3+1−2(x+6)x2+1].4x2+4x+1(x+3)(4−x)[(x4−x+x−3x3+1).(x3−2x2+2x−1)(x+1)x9+x7−3x2−3+1−2(x+6)x2+1].4x2+4x+1(x+3)(4−x)a, Tìm giá trị của x để giá trị của biểu thức B được xác định
b, Rút gọn B
c, Cmr với các giá trị của x mà giá trị của biểu thức xác định thì −5≤B≤0
Cho đa thức
P
(
x
)
3
x
2
-
3
x
-
1
+
x
4
Q
(
x
)
5
x
3
+
2
x
4
-
x
2
-
5
x
3
-
x
4
+
1
+
3...
Đọc tiếp
Cho đa thức
P ( x ) = 3 x 2 - 3 x - 1 + x 4 Q ( x ) = 5 x 3 + 2 x 4 - x 2 - 5 x 3 - x 4 + 1 + 3 x 2 + 5 x 2
Tìm đa thức R(x) sao cho P ( x ) + R ( x ) = Q ( x )
A. 4 x 2 + 3 x + 2
B. 4 x 2 - 3 x + 2
C. - 4 x 2 + 3 x + 2
D. 4 x 2 + 3 x - 2
Thu gọn Q(x) = x4 + 7x2 + 1
Khi đó R(x) = Q(x) - P(x) = 4x2 + 3x + 2. Chọn A
Đúng 0
Bình luận (0)
tìm nghiệm của đa thức C(x) biết C(x)= x4 + 3x2 - 2 + 2
Tìm giá trị nhỏ nhất của các biểu thức sau:
a) A = (x2 - 9)2 + |y - 2| - l; b) B = x4 + 3x2 +2;
a, Có \(\left(x^2-9\right)^2\)≥0 ∀ x ∈ Z
|y-2| ≥0 ∀ y ∈ Z
⇒ Gía trị nhỏ nhất A=-1. Dấu ''='' xảy ra khi:\(\left(x^2-9\right)^2\)+|y-2|=0
⇒ \(x=3\) ; \(y=2\)
Vậy.....
b, Có \(x^4\) ≥ 0 ∀ x ∈ Z
3\(x^2\) ≥ 0 ∀ x ∈ Z
⇒ Giá trị nhỏ nhất của B=2. Dấu ''='' xảy ra khi: \(x^4\)+3\(x^2\)=0
⇒ \(x^2\left(x^2+3\right)\)=0
⇒ \(x^2\) =0
⇒ \(x=0\)
Vậy...
Đúng 2
Bình luận (0)
a)Tìm số nguyên x để A= x4 - 9x4+ 21x2+ x + a B = x-2
b)Tìm số nguyên x để A= x4 - 10x3 + 21x2 + 8x + a B= x+2
c) Tìm số nguyên x để A= 2x3 - 3x2 + ax + b B= x2-2+2
a:
Sửa đề: A=x^4-9x^3+21x^2+x+a
A chia hết cho B
=>x^4-2x^3-7x^3+14x^2+7x^2-14x+15x-30+a+30 chia hết cho x-2
=>a+30=0
=>a=-30
b: A chia hết cho B
=>x^4+2x^3-12x^3-24x^2+45x^2+90x-82x-164+a+164 chia hết cho x+2
=>a+164=0
=>a=-164
Đúng 1
Bình luận (1)
Bài 1 (2điểm)1) Nêu điều kiện để √a có nghĩa ?2) Áp dụng: Tìm x để các căn thức sau có nghĩa:Bài 2: ( 3 điểm ): Rút gọn biểu thứcBài 3 ( 4 điểm ) Cho biểu thức(Với x 0; x 1; x4)a/ Rút gọn P.b/ Với giá trị nào của x thì P có giá trị bằng 1/4c/ Tính giá trị của P tại x 4 + 2√3d/ Tìm số nguyên x để biểu thức P có giá trị là số nguyên ?Bài 4 : ( 1 điểm ): ChoTìm giá trị nhỏ nhất của A, giá trị đó đạt được khi x bằng bao nhiêu?
Đọc tiếp
Bài 1 (2điểm)
1) Nêu điều kiện để √a có nghĩa ?
2) Áp dụng: Tìm x để các căn thức sau có nghĩa:
Bài 2: ( 3 điểm ): Rút gọn biểu thức
Bài 3 ( 4 điểm ) Cho biểu thức
(Với x > 0; x 1; x4)
a/ Rút gọn P.
b/ Với giá trị nào của x thì P có giá trị bằng 1/4
c/ Tính giá trị của P tại x = 4 + 2√3
d/ Tìm số nguyên x để biểu thức P có giá trị là số nguyên ?
Bài 4 : ( 1 điểm ): Cho
Tìm giá trị nhỏ nhất của A, giá trị đó đạt được khi x bằng bao nhiêu?
Bài 1: Cho phân thức: 3x2+6x+12x3−83x2+6x+12x3−8a,Tìm điều kiện của x để phân thức đã cho được xác địnhb, Rút gọn phân thứcc, Tính giá trị của phân thức sau khi rút gọn với x 4001200040012000Bài 2: Cho phân thức: x2−10x+25x2−5xx2−10x+25x2−5xa, Tìm giá trị của x để phân thức bằng 0b, Tìm x để giá trị của phân thức bằng 5252c, Tìm x nguyên để phân thức có giá trị nguyênBài 3: Cho biểu thức: (x+12x−2+3x2−1−x+32x+2)(4x2−45)(x+12x−2+3x2−1−x+32x+2)(4x2−45)a, Tìm điều kiện của x để giá trị của biểu th...
Đọc tiếp
Bài 1: Cho phân thức: 3x2+6x+12x3−83x2+6x+12x3−8
a,Tìm điều kiện của x để phân thức đã cho được xác định
b, Rút gọn phân thức
c, Tính giá trị của phân thức sau khi rút gọn với x = 4001200040012000
Bài 2: Cho phân thức: x2−10x+25x2−5xx2−10x+25x2−5x
a, Tìm giá trị của x để phân thức bằng 0
b, Tìm x để giá trị của phân thức bằng 5252
c, Tìm x nguyên để phân thức có giá trị nguyên
Bài 3: Cho biểu thức: (x+12x−2+3x2−1−x+32x+2)(4x2−45)(x+12x−2+3x2−1−x+32x+2)(4x2−45)
a, Tìm điều kiện của x để giá trị của biểu thức được xác định
b, CMR: Khi giá trị của biểu thức được xác định thì nó không phụ thuộc vào giá trị của biến x
x2−10x+25x2−5x=(x−5)2x(x−5)=x−5x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x2−10x+25x2−5x=(x−5)2x(x−5)=x−5x
x−5x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x−5x phải có giá trị nguyên.
x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)
x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5
(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5
(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
2(x+1)25+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline-table; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2(x+1)25+185−25x2−45x
2(x2+2x+1)5+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2(x2+2x+1)5+185−25x2−45x
2x2+4x+25+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+25+185−25x2−45x
2x2+4x+2+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+2+185−25x2−45x
2x2+4x+205−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+205−25x2−45x
c) tự làm, đkxđ: x≠1;x≠−1
Tìm giá trị nguyên của biến x để tại đó giá trị của mỗi biểu thức sau là một số nguyên: 3 x 2 - x + 1 3 x + 2
Ta có:
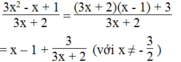
Vì x là số nguyên nên x – 1 là số nguyên.
Để biểu thức đã cho là số nguyên thì 3 ⋮ (3x + 2) và x ≠ -2/3
Suy ra: 3x + 2 ∈ Ư(3) = {-3; -1; 1; 3}
Ta có: 3x + 2 = -3 ⇒ x = -5/3 ∉ Z (loại)
3x + 2 = -1 ⇒ x = - 1
3x + 2 = 1 ⇒ x = -1/3 ∉ Z (loại)
3x + 2 = 3 ⇒ x = 1/3 ∉ Z (loại)
x = -1 khác -3/2
Vậy với x = - 1 thì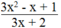
có giá trị nguyên.
Đúng 0
Bình luận (0)
Cho hai đa thức: A(x) = x4 + 2 – 3x2 – x3
và B(x) = 3x2 + x4 + 5
a/ Sắp xếp các hạng tử của đa thức A(x) và B(x) theo lũy thừa giảm dần của biến ?
b/ Tính A(x) + B(x)
c/ Chứng tỏ đa thức B(x) không có nghiệm
a: A(x)=x^4-x^3-3x^2+2
B(x)=x^4+3x^2+5
b: A(x)+B(x)=2x^4-x^3+7
c: B(x)=x^2(x^2+3)+5>0
=>B(x) ko có nghiệm
Đúng 0
Bình luận (0)
Cho đa thức: P(x) = x4 - 3x2 + 1/2 - x.
Tìm các đa thức Q(x), R(x) sao cho:
P(x) - R(x) = x3
