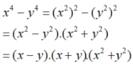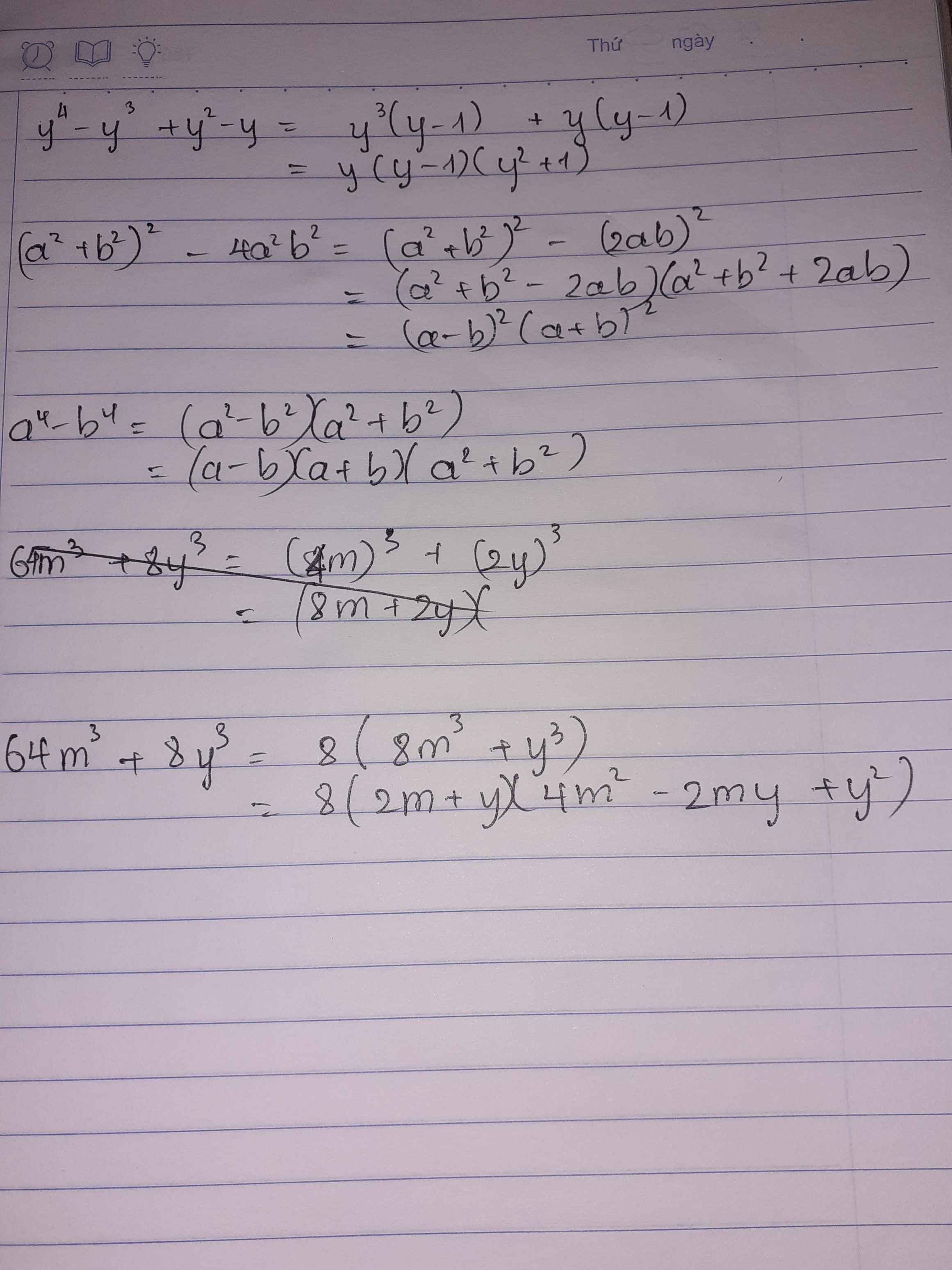Phân tích đa thức thành nhân tử: 64x4 + y4

Những câu hỏi liên quan
64x4+y4 phân tích đa thức thành nhân tử
Đọc tiếp
64x4+y4 phân tích đa thức thành nhân tử
64x^4+y^4
=64x^4+16x^2y^2+y^4-16x^2y^2
=(8x^2+y^2)^2-(4xy)^2
=(8x^2-4xy+y^2)(8x^2+4xy+y^2)
Đúng 0
Bình luận (0)
phân tích đa thức thành nhân tử
a) x4+\(\dfrac{1}{4}\)y8
b) \(\dfrac{1}{4}\)x4+y8
c) 64x4+y4
d) x4+\(\dfrac{1}{64}\)y4
Lời giải:
a.
$=(x^2)^2+(\frac{1}{2}y^4)^2+2.x^2.\frac{1}{2}y^4-x^2y^4$
$=(x^2+\frac{1}{2}y^4)^2-(xy^2)^2$
$=(x^2+\frac{1}{2}y^4-xy^2)(x^2+\frac{1}{2}y^4+xy^2)$
b.
$=(\frac{1}{2}x^2)^2+(y^4)^2+2.\frac{1}{2}x^2.y^4-x^2y^4$
$=(\frac{1}{2}x^2+y^4)^2-(xy^2)^2$
$=(\frac{1}{2}x^2+y^4-xy^2)(\frac{1}{2}x^2+y^4+xy^2)$
c.
$=(8x^2)^2+(y^2)^2+2.8x^2.y^2-16x^2y^2$
$=(8x^2+y^2)^2-(4xy)^2=(8x^2+y^2-4xy)(8x^2+y^2+4xy)$
d.
$=\frac{64x^4+y^4}{64}=\frac{1}{64}(8x^2+y^2-4xy)(8x^2+y^2+4xy)$
Đúng 1
Bình luận (0)
c: \(64x^4+y^4\)
\(=64x^4+16x^2y^2+y^4-16x^2y^2\)
\(=\left(8x^2+y^2\right)^2-\left(4xy\right)^2\)
\(=\left(8x^2+y^2-4xy\right)\left(8x^2+y^2+4xy\right)\)
Đúng 0
Bình luận (0)
BÀi 1: Phân tích đa thức thành nhân tử
a)x3+8x2+17x+10
b)abc+ab+bc+ca+a+b+c+1
c)4x4+81
d)64x4+y4
e)x5+x4+1
f)x+2y-xy-2
g)a2+b2-x2-y2+2ab-2xy
a. = \(\left(x^3+x^2\right)+\left(7x^2+7x\right)+\left(10x+10\right)\)
= \(x^2\left(x+1\right)+7x\left(x+1\right)+10x\left(x+1\right)\)
= \(\left(x+1\right)\left(x^2+7x+10x\right)\)
= \(\left(x+1\right)\left(x+2\right)\left(x+5\right)\)
Đúng 0
Bình luận (0)
phân tích đa thức thành nhân tử: x4 +x2y2+y4
x⁴ + x²y² +y⁴
= (x²)² + x²y² + (y²)²
= (x²)² + x²y² + (y²)² + x²y² - x²y²
= (x²)² + 2 x²y² + (y²)² - x²y²
= (x² + y²)²- (xy)²
=(x² + y² + xy)(x² + y² - xy)
Đúng 2
Bình luận (0)
Phân tích các đa thức sau thành nhân tử: c ) x 4 - y 4
x^4-y^4=(x^2-y^2)(x^2+y^2)=(x-y)(x+y)(x^2+y^2)
phân tích đa thức thành nhân tử
y4-y3+y2-y
(a2+b2)2 - 4a2b2
a4- b4
64m3+8y3
b) \(\left(a^2+b^2\right)^2-4a^2b^2\)
\(=\left(a^2-2ab+b^2\right)\left(a^2+2ab+b^2\right)\)
\(=\left(a-b\right)^2\cdot\left(a+b\right)^2\)
c) \(a^4-b^4=\left(a-b\right)\left(a+b\right)\left(a^2+b^2\right)\)
Đúng 0
Bình luận (0)
a) \(y^4-y^3+y^2-y\)
\(=y^3\left(y-1\right)+y\left(y-1\right)\)
\(=y\left(y-1\right)\left(y^2+1\right)\)
d) \(64m^3+8y^3=\left(4m+2y\right)\left(16m^2-8my+4y^2\right)\)
Đúng 0
Bình luận (0)
Toán vận dụng: phân tích đa thức: x4-y4+2x3y-2xy3 thành nhân tử (x+y).(x2-y2)
\(x^4-y^4+2x^3y-2xy^3\)
\(=\left(x^2+y^2\right)\left(x^2-y^2\right)+2xy\left(x^2-y^2\right)\)
\(=\left(x^2-y^2\right)\left(x^2+y^2+2xy\right)\)
\(=\left(x-y\right)\left(x+y\right)\left(x+y\right)^2\)
\(=\left(x-y\right)\left(x+y\right)^3\)
Đúng 0
Bình luận (1)
\(x^4-y^4+2x^3y-2xy^3\\ =\left(x^2\right)^2-\left(y^2\right)^2+2xy\left(x^2-y^2\right)\\ =\left(x^2-y^2\right)\left(x^2+y^2\right)+2xy\left(x^2-y^2\right)\\ =\left(x^2-y^2\right)\left(x^2+y^2+2xy\right)\\ =\left(x-y\right)\left(x+y\right)\left(x+y\right)^2\\ =\left(x-y\right)\left(x+y\right)^3\)
Đúng 0
Bình luận (2)
Mọi người ơi , giải chi tiết hộ em bài này nha! ( Ai học 3 phương pháp mở rộng của Phân tích đa thức thành nhân tử hoặc học sinh giỏi toán thì giúp em với ạ)
a) 6x2 - 11x + 3
b) 2x2 + 3x - 27
c) ( x2 + x + 2 ) . ( x2 + x +5 ) - 4 ( dấu chấm là dấu nhân ạ )
d) 4x4 + 1
e) 64x4 + y4
f) x2 + 4xy + 3y2
a) \(6x^2-11x+3\)
\(=6x^2-9x-2x+3\)
\(=3x\left(2x-3\right)-\left(2x-3\right)\)
\(=\left(3x-1\right)\left(2x-3\right)\)
Đúng 0
Bình luận (0)
b) \(2x^2+3x-27\)
\(=2x^2-6x+9x-27\)
\(=2x\left(x-3\right)+9\left(x-3\right)\)
\(=\left(2x+9\right)\left(x-3\right)\)
Đúng 0
Bình luận (0)
f) \(x^2+4xy+3y^2\)
\(=x^2+xy+3xy+3y^2\)
\(=x\left(x+y\right)+3y\left(x+y\right)\)
\(=\left(3y+x\right)\left(x+y\right)\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Phân tích các đa thức sau thành nhân tử rồi tính giá trị đa thức:a) A 9x2 + 15x + 6xy + y2 + 5y biết 3x + y 0b) B 25x2 – y4 – 5x + y2
Đọc tiếp
Phân tích các đa thức sau thành nhân tử rồi tính giá trị đa thức:
a) A = 9x2 + 15x + 6xy + y2 + 5y biết 3x + y = 0
b) B = 25x2 – y4 – 5x + y2
Lời giải:
a. $A=9x^2+15x+6xy+y^2+5y=(9x^2+6xy+y^2)+(15x+5y)$
$=(3x+y)^2+5(3x+y)=0^2+5.0=0$
b. $25x^2-y^4-5x+y^2=(25x^2-y^4)-(5x-y^2)=(5x-y^2)(5x+y^2)-(5x-y^2)$
$=(5x-y^2)(5x+y^2-1)$
Đúng 0
Bình luận (0)
phân tích đa thức thành nhân tử
x4+x2y2+y4
`x^4+x^2 y^2+y^4`
`=x^4+2x^2 y^2 +y^4-x^2 y^2`
`=(x^2+y^2)^2-(xy)^2`
`=(x^2-xy+y^2)(x^2+xy+y^2)`
Đúng 1
Bình luận (0)