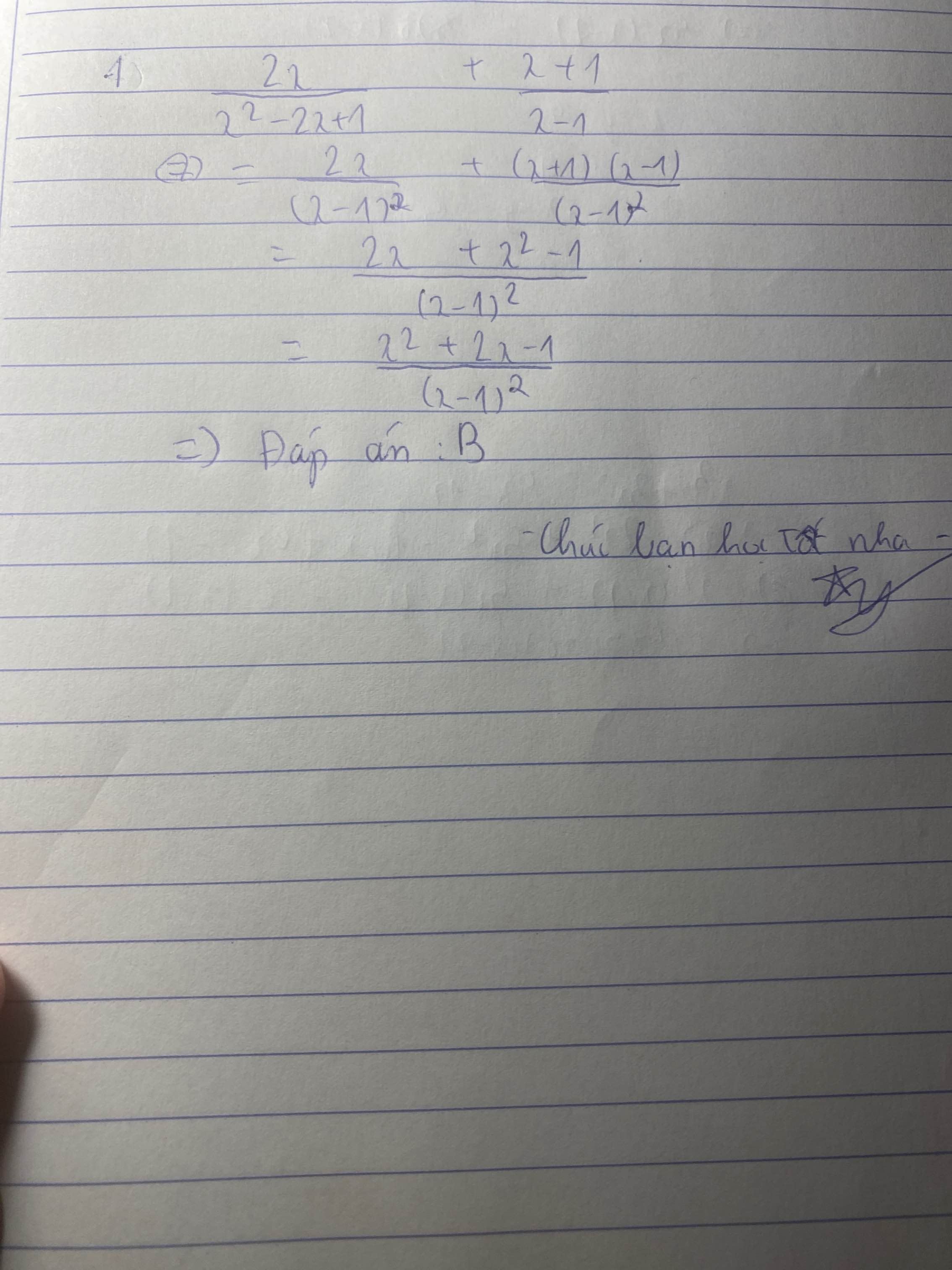\(\dfrac{x}{x-y}-\dfrac{1}{x-y}-\dfrac{1-y}{y-x}\)
cong tru phan thuc

Những câu hỏi liên quan
tim z,y,z thoa
can x cong can tat ca y tru 1 cong can tat ca z tru 2 bang 1 phan 2 ( x cong y cong z)
tim GTN cua B bang 1 phan x tru can x cong 1
tim x thuoc z de can x cong 1 phan can 3 tru 3 la so nguyen
tinh tong T bang 1 phan can 1 cong can 2 cong 1 phan can 2 cong can 3 cong ... cong den 1 phan can 99 cong can 100
bai 1 thuc hien phep tinh a)\(\dfrac{\left(\dfrac{1}{x^2+4x+4}-\dfrac{1}{x^2-4x+4}\right)}{\left(\dfrac{1}{x+2}+\dfrac{1}{x-2}\right)}\)
b)\(\left(\dfrac{5x+y}{x^2-5xy}+\dfrac{5x-y}{x^2+5xy}\right)\cdot\dfrac{x^2-25y^2}{x^2+y^2}\)
\(a,=\dfrac{\left(x-2\right)^2-\left(x+2\right)^2}{\left(x-2\right)^2\left(x+2\right)^2}:\dfrac{x-2+x+2}{\left(x-2\right)\left(x+2\right)}\\ =\dfrac{-8x}{\left(x-2\right)^2\left(x+2\right)^2}\cdot\dfrac{\left(x-2\right)\left(x+2\right)}{2x}=\dfrac{-4}{\left(x-2\right)\left(x+2\right)}\)
\(b,=\dfrac{5x^2+26xy+5y^2+5x^2-26xy+5y^2}{x\left(x-5y\right)\left(x+5y\right)}\cdot\dfrac{\left(x-5y\right)\left(x+5y\right)}{x^2+y^2}\\ =\dfrac{10\left(x^2+y^2\right)}{x\left(x^2+y^2\right)}=\dfrac{10}{x}\)
Đúng 0
Bình luận (0)
1) Thuc hien phep tinh cong 2 phan thuc \(\dfrac{2x}{x^2-2x+1}+\dfrac{x+1}{x-1}\) duoc ket qua la:
A. \(\dfrac{x^2+2x+1}{\left(x-1\right)^2}\) B. \(\dfrac{x^2+2x-1}{\left(x-1\right)^2}\) C. \(\dfrac{x^2-x-1}{\left(x-1\right)^2}\) D. \(\dfrac{x^2-2x-1}{\left(x-1\right)^2}\)
cho x,y là các số thực dương phan biệt thõa mãn \(\dfrac{1}{1+x}\)+ \(\dfrac{1}{1+y}\)=\(\dfrac{2}{1+\sqrt{xy}}\). Tính giá trị biểu thức A= \(\dfrac{1}{1+x}\)+ \(\dfrac{1}{1+y}\)- \(\dfrac{1}{1+\sqrt{xy}}\).
thuc hien phep tinh
a.left(dfrac{2x+1}{2x-1}-dfrac{2x-1}{2x+1}right):dfrac{4x}{10x-5}
b.left(dfrac{1}{x^2+1}-dfrac{2-x}{x+1}right):left(dfrac{1}{x}+1-2right)
c.dfrac{1}{x-1}-dfrac{x^3-x}{x^2+1}.left(dfrac{1}{x^2-2x+1}+dfrac{1}{1-x^2}right)
d.left(dfrac{x^2+xy}{x^3+x^2y+xy^2+y^3}+dfrac{y}{x^2+y^2}right):left(dfrac{1}{x-y}-dfrac{2xy}{x^3-x^2y+xy^2-y^3}right)
Đọc tiếp
thuc hien phep tinh
a.\(\left(\dfrac{2x+1}{2x-1}-\dfrac{2x-1}{2x+1}\right):\dfrac{4x}{10x-5}\)
b.\(\left(\dfrac{1}{x^2+1}-\dfrac{2-x}{x+1}\right):\left(\dfrac{1}{x}+1-2\right)\)
c.\(\dfrac{1}{x-1}-\dfrac{x^3-x}{x^2+1}.\left(\dfrac{1}{x^2-2x+1}+\dfrac{1}{1-x^2}\right)\)
d.\(\left(\dfrac{x^2+xy}{x^3+x^2y+xy^2+y^3}+\dfrac{y}{x^2+y^2}\right):\left(\dfrac{1}{x-y}-\dfrac{2xy}{x^3-x^2y+xy^2-y^3}\right)\)
a: \(=\dfrac{4x^2+4x+1-\left(4x^2-4x+1\right)}{\left(2x-1\right)\left(2x+1\right)}\cdot\dfrac{5\left(2x-1\right)}{4x}\)
\(=\dfrac{8x}{2x+1}\cdot\dfrac{5}{4x}=\dfrac{10}{2x+1}\)
c: \(=\dfrac{1}{x-1}-\dfrac{x\left(x-1\right)\left(x+1\right)}{x^2+1}\cdot\left(\dfrac{x+1-x+1}{\left(x-1\right)^2\cdot\left(x+1\right)}\right)\)
\(=\dfrac{1}{x-1}-\dfrac{x}{x^2+1}\cdot\dfrac{2}{\left(x-1\right)}=\dfrac{x^2+1-2x}{\left(x-1\right)\left(x^2+1\right)}=\dfrac{x-1}{x^2+1}\)
Đúng 0
Bình luận (0)
cho x,y,z la cac so nguyen duong thoa man \(\dfrac{1}{x}+\dfrac{1}{y}+\dfrac{1}{z}=2015\)
tinh gia tri lon nhat cua bieu thuc P=\(\dfrac{xy}{x^3+y^3}+\dfrac{yz}{y^3+z^3}+\dfrac{zx}{z^{3+x^3}}\)
Cho \(\dfrac{x}{y+z+t}=\dfrac{y}{z+t+x}=\dfrac{z}{t+x+y}=\dfrac{t}{x+y+z}\). Tinh gia tri cua bieu thuc :
\(P=\dfrac{x+y}{z+t}+\dfrac{y+z}{t+x}+\dfrac{z+t}{x+y}+\dfrac{t+x}{y+z}\)
Ta có: \(\dfrac{x}{y+z+t}=\dfrac{y}{z+t+x}=\dfrac{z}{t+x+y}=\dfrac{t}{x+y+z}\)
\(\Rightarrow\dfrac{x}{y+z+t}+1=\dfrac{y}{z+t+x}+1=\dfrac{z}{t+x+y}+1=\dfrac{t}{x+y+z}+1\)\(\Rightarrow\dfrac{x+y+z+t}{y+z+t}=\dfrac{x+y+z+t}{z+t+x}=\dfrac{x+y+z+t}{t+x+y}=\dfrac{x+y+z+t}{x+y+z}\) (*)
+) Nếu \(x+y+z+t\ne0\) thì từ (*) suy ra:
\(y+z+t=z+t+x=t+x+y=x+y+z\)
\(\Rightarrow x=y=z=t\)
\(\Rightarrow P=\dfrac{x+x}{x+x}+\dfrac{x+x}{x+x}+\dfrac{x+x}{x+x}+\dfrac{x+x}{x+x}\) \(\Rightarrow P=1+1+1+1=4\)
+) Nếu \(x+y+z+t=0\) thì \(\left\{{}\begin{matrix}x+y=-\left(z+t\right)\\y+z=-\left(t+x\right)\\z+t=-\left(x+y\right)\\t+x=-\left(y+z\right)\end{matrix}\right.\)
\(\Rightarrow P=\dfrac{-\left(z+t\right)}{z+t}+\dfrac{-\left(t+x\right)}{t+x}+\dfrac{-\left(x+y\right)}{x+y}+\dfrac{-\left(y+z\right)}{y+z}\)\(\Rightarrow P=\left(-1\right)+\left(-1\right)+\left(-1\right)+\left(-1\right)=-4\)
Vậy \(P=4\) hoặc \(P=-4\)
Đúng 0
Bình luận (0)
chung to hieu bang mot phan thuc có tử bằng 1
\(\dfrac{1}{xy-x^2}-\dfrac{1}{y^2-xy}\)
\(\dfrac{1}{xy-x^2}-\dfrac{1}{y^2-xy}\)
\(=\dfrac{y}{xy\left(y-x\right)}-\dfrac{x}{xy\left(y-x\right)}\)
\(=\dfrac{y-x}{xy\left(y-x\right)}\)
\(=\dfrac{1}{xy}\)
\(\Rightarrow\) Đpcm.
Đúng 0
Bình luận (0)
BT:Viet phân thức bang moi phân thức sau bang cach doi dau ca tu va mau phan thuc:
a,\(\dfrac{-3}{y-x}\)
b, \(\dfrac{-5}{-x-y}\)
c,\(\dfrac{x\left(x-2\right)}{-x-1}\)
A \(\dfrac{3}{x-y}\)
b \(\dfrac{5}{x+y}\)
c \(\dfrac{2x-x^2}{x+1}\)
Đúng 0
Bình luận (0)