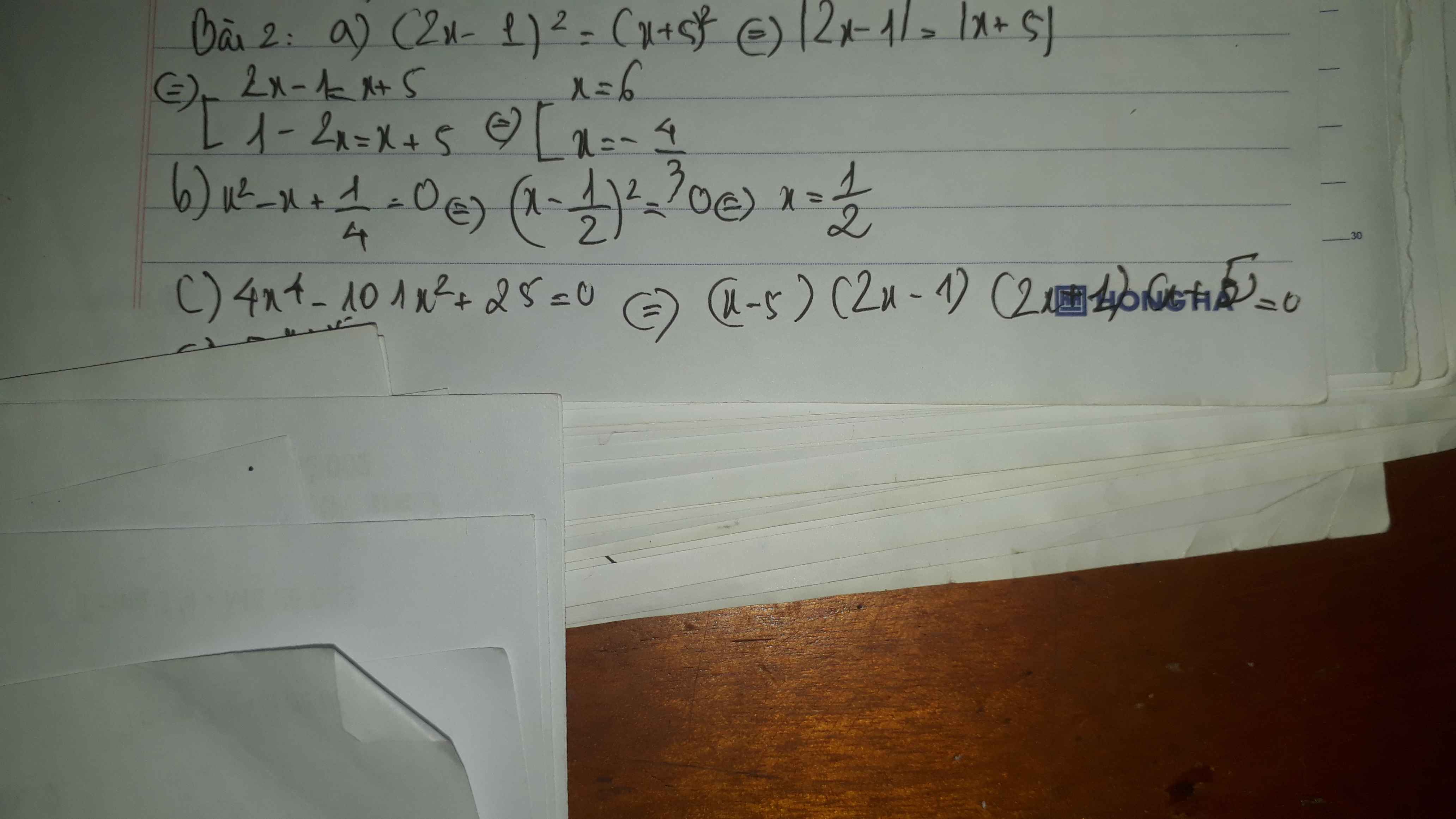Tìm x biết:(3x-5)^2017=(3x-5)^2019

Những câu hỏi liên quan
|5-3x|-|2-x|+3x= 2017 ( tìm x biết)
Tìm giá trị nhỏ nhất của
P=|x+3|+|2x-5|+|x+7|
Q=|x+1|+|3x-a|+|x-1|+5
M=|x+2017|+|x-2018|+|x-2019|
Ai nhanh mình tick
Giải phương trình :
a, x/2017 + x - 1/2018 = x - 2/2019 - 1
b, ( 2x + 1 ) + ( 4x + 3 ) + ( 6x + 5 ) +...+ ( 100x + 99 ) = 7600
c, ( x + 1 ) + ( 2x + 5 ) + ( 3x + 9 ) +...+ ( nx + 101 ) = 975
d, ( x - 1 ) + ( 2x - 3 ) + ( 3x - 5 ) +...+ ( nx - 2019 ) = n
Giải giúp mình nha, cảm ơn nhìu ❤
b. \(\left(2x+1\right)+\left(4x+3\right)+\left(6x+5\right)+...+\left(100x+99\right)=7600\)
\(\rightarrow\left(2x+4x+6x+...+100x\right)+\left(1+3+5+...+99\right)=7600\)
\(\rightarrow\frac{\left(2x+100x\right).50}{2}+\frac{\left(1+99\right).50}{2}=7600\)
\(\rightarrow51x.50+50.50=7600\)
\(\rightarrow51x.50+2500=7600\)
\(\rightarrow51x.50=7600-2500\)
\(\rightarrow51x.50=5100\)
\(\rightarrow50x=100\)
\(\rightarrow x=\frac{100}{50}=2\)
Vậy x = 2
Bt1: Tính
a) 350-[120-7^2:(5^3-59.2)]
Bt2:Tìm x biết:
a) 2x+2^3:2^2=4^3:4^2
b) 405-3x=27:3^2
Bt3:Tính hợp lý:
a) A=1+3+5+....+2021
b) B=2021-2019+2017-2015+.....+5-3
Giải phương trình: \(|x-2017|+|2x-2018|+|3x-2019|=x-2020\)
Nhận thấy vế trái luôn dương nên \(x-2020\ge0\Leftrightarrow x\ge2020\)
Với \(x\ge2020\Rightarrow\left\{{}\begin{matrix}x-2017\ge0\\2x-2018\ge0\\3x-2019\ge0\end{matrix}\right.\)
PT trở thành: \(x-2017+2x-2018+3x-2019=x-2020\)
Hay kết hợp với điều kiện \(x=\dfrac{4034}{5}\) suy ra PT đã cho vô nghiệm
Đúng 2
Bình luận (2)
\(\left|x-2017\right|+\left|2x-2018\right|+\left|3x-2019\right|=x-2020\)
\(ĐK:x\ge2020\)
\(\Leftrightarrow x-2017+2x-2018+3x-2019=x-2020\)
\(\Leftrightarrow5x=4034\)
\(\Leftrightarrow x=806,8\left(tm\right)\)
Vậy \(S=\left\{806,8\right\}\)
Đúng 0
Bình luận (2)
Bài 7. Tìm x,biết:
a) x-3x2=0 e) 5x(3x-1)+x(3x-1)-2(3x-1)=0
b) (x+3)2-x(x-2)=13 c) (x-4)2-36=0
d) x2-7x+12=0 g) x2-2018x-2019=0
Bài 8. Tìm x, biết
a) (2x-1)2=(x+5)2 b) x2-x+1/4
c) 4x4-101x2+25=0 d) x3-3x2+9x-91=0
Tìm x, biết : \(\left(3x-2^4\right).7^5=2.7^6.\frac{1}{2017^0}\)
( 3x - 24 ) . 75 = 2.76 .1/20170
( 3x - 24 ) . 75 =235298
( 3x - 24 ) = 235298 : 75
( 3x - 24 ) =14
3x = 14 + 24
3x = 30
x = 0
dung 100%
Đúng 0
Bình luận (0)
\(\left(3x-2^4\right).7^5=2.7^6.\frac{1}{2017^0}\)
\(\Leftrightarrow\left(3x-16\right).7^5=2.7^6.1\)
\(\Leftrightarrow3x-16=\frac{2.7^6}{7^5}\)
\(\Leftrightarrow3x-16=2.7\)
\(\Leftrightarrow3x-16=14\)
\(\Leftrightarrow3x=30\)
\(\Leftrightarrow x=10\)
Đúng 0
Bình luận (0)
Nhưng mình làm cách này có được không : (Tự nhiên nghĩ được)
(3x-2^4).7^5=2.7^6.1/2017^0
(3x-2^4).7^5=2.7.7^5.1
3x-16=2.7 ( Bớt cả hai vế cho 7^5)
3x=14+16
x=30:3
x=10
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Giải phương trình: |x-2017|+|2x-2018|+|3x-2019|=x-2020
(3x-7)^2019=(3x-7)^2017
\(\left(3x-7\right)^{2019}=\left(3x-7\right)^{2017}\)
\(\Rightarrow\left(3x-7\right)^{2019}-\left(3x-7\right)^{2017}=0\)
\(\Rightarrow\left(3x-7\right)^{2017}\left[\left(3x-7\right)^2-1\right]=0\)
\(\Rightarrow\left(3x-7\right)^{2017}=0\text{ hoặc }\left[\left(3x-7\right)^2-1\right]=0\)
\(\Rightarrow3x-7=0\text{ hoặc }\left(3x-7\right)^2=1\)
\(\Rightarrow3x-7=0\text{ hoặc } \hept{\begin{cases}3x-7=1\\3x-7=-1\end{cases}}\)
\(\Rightarrow3x=7\text{ hoặc }3x=8\text{ hoặc }3x=6\)
\(\Rightarrow x=\frac{7}{3}\text{ hoặc }x=\frac{8}{3}\text{ hoặc }x=2\)
Đúng 0
Bình luận (0)
\(\left(3x-7\right)^{2019}=\left(3x-7\right)^{2017}\)
\(\left(3x-7\right)^{2019}-\left(3x-7\right)^{2017}=0\)
\(\left(3x-7\right)^{2017}\cdot\left[\left(3x-7\right)^2-1\right]=0\)
\(\Rightarrow\orbr{\begin{cases}\left(3x-7\right)^{2017}=0\\\left(3x-7\right)^2-1=0\end{cases}}\) \(\Rightarrow\orbr{\begin{cases}3x-7=0\\\left(3x-7\right)^2=1\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}3x=7\\3x-7=\pm1\end{cases}}\) \(\Rightarrow\orbr{\begin{cases}x=\frac{7}{3}\\3x=6\text{ hoặc }3x=8\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=\frac{7}{3}\\x=2\text{ hoặc }x=\frac{8}{3}\end{cases}}\)
\(\Rightarrow x\in\left\{\frac{7}{3};2;\frac{8}{3}\right\}\)
Đúng 0
Bình luận (0)
Bài giải
\(\left(3x-7\right)^{2019}=\left(3x-7\right)^{2017}\)
\(\left(3x-7\right)^{2019}-\left(3x-7\right)^{2017}=0\)
\(\left(3x-7\right)^{2017}\cdot\left[\left(3x-7\right)^2-1\right]=0\)
\(\Rightarrow\orbr{\begin{cases}\left(3x-7\right)^{2017}=0\\\left(3x-7\right)^2-1=0\end{cases}}\) \(\Rightarrow\orbr{\begin{cases}3x-7=0\\\left(3x-7\right)^2=1\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}3x=7\\3x-7=-1\text{ hoặc }3x-7=1\end{cases}}\) \(\Rightarrow\orbr{\begin{cases}x=\frac{7}{3}\\3x=6\text{ hoặc }3x=8\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=\frac{7}{3}\\x=2\text{ hoặc }x=\frac{8}{3}\end{cases}}\)
\(\Rightarrow\text{ }x\in\left\{\frac{7}{3}\text{ ; }2\text{ ; }\frac{8}{3}\right\}\)
Nếu có điều kiện gì thì bạn chọn x nếu không thì chọn cả 3 đáp án như trên nha !
Đúng 0
Bình luận (0)
Xem thêm câu trả lời










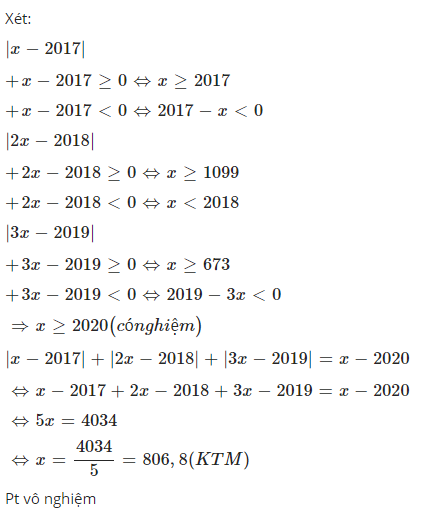







 Bài 1.
Bài 1.