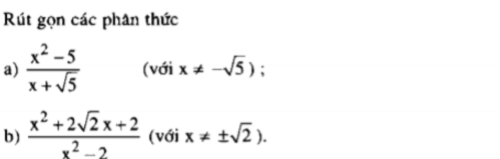e, \(A=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}=\dfrac{\sqrt{x}-1+2}{\sqrt{x}-1}\)
Để A đạt giá trị nguyên thì \(\sqrt{x}-1=\left\{\pm1;\pm2\right\}\)
⇒\(\left[{}\begin{matrix}\sqrt{x}-1=-1\\\sqrt{x}-1=1\\\sqrt{x}-1=2\\\sqrt{x}-1=-2\left(vll\right)\end{matrix}\right.\leftrightarrow\left[{}\begin{matrix}x=0\\x=4\\x=9\end{matrix}\right.\)
*vll là vô lí loại
@hoctot_nha
a: Ta có: \(A=\left(\dfrac{x-\sqrt{x}}{\sqrt{x}-1}+1\right):\left(\dfrac{x+2\sqrt{x}}{\sqrt{x}+2}-1\right)\)
\(=\dfrac{\left(\sqrt{x}+1\right)}{\sqrt{x}-1}\)
b: Thay x=9 vào A, ta được:
\(A=\dfrac{3+1}{3-1}=2\)
c: Để A=5 thì \(\sqrt{x}+1=5\sqrt{x}-5\)
\(\Leftrightarrow-4\sqrt{x}=-6\)
hay \(x=\dfrac{9}{4}\)
a)\(A=\left(\dfrac{x-\sqrt{x}}{\sqrt{x}-1}+1\right):\left(\dfrac{x+2\sqrt{x}}{\sqrt{x}+2}-1\right)=\dfrac{x-\sqrt{x}+\sqrt{x}-1}{\sqrt{x}-1}:\dfrac{x+2\sqrt{x}-\sqrt{x}-2}{\sqrt{x}+2}=\dfrac{x-1}{\sqrt{x}-1}.\dfrac{\sqrt{x}+2}{x+\sqrt{x}-2}=\dfrac{x-1}{\sqrt{x}-1}.\dfrac{1}{\sqrt{x}-1}=\dfrac{x-1}{\left(\sqrt{x}-1\right)^2}\)
b) Thay x=9 vào A ta được: \(A=\dfrac{x-1}{\left(\sqrt{x}-1\right)^2}=\dfrac{9-1}{\left(\sqrt{9}-1\right)^2}=2\)
c) Để A =5 thì \(\dfrac{x-1}{\left(\sqrt{x}-1\right)^2}=5\Leftrightarrow x-1=5\left(x-2\sqrt{x}+1\right)\Leftrightarrow4x-10\sqrt{x}+6=0\Leftrightarrow4\left(\sqrt{x}-\dfrac{3}{2}\right)\left(\sqrt{x}-1\right)=0\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=\dfrac{3}{2}\\\sqrt{x}=1\end{matrix}\right.\Leftrightarrow x=\dfrac{9}{4}\)(do x≠1)
d: Để A<5 thì A-5<0
\(\Leftrightarrow\dfrac{\sqrt{x}+1-5\sqrt{x}+5}{\sqrt{x}-1}< 0\)
\(\Leftrightarrow\dfrac{-4\sqrt{x}+6}{\sqrt{x}-1}< 0\)
\(\Leftrightarrow\dfrac{2\sqrt{x}-3}{\sqrt{x}-1}>0\)
\(\Leftrightarrow\left[{}\begin{matrix}2\sqrt{x}-3>0\\\sqrt{x}-1< 0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x>\dfrac{9}{4}\\0< x< 1\end{matrix}\right.\)
e: Để A nguyên thì \(\sqrt{x}+1⋮\sqrt{x}-1\)
\(\Leftrightarrow\sqrt{x}-1\in\left\{-1;1;2\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{0;2;3\right\}\)
hay \(x\in\left\{4;9\right\}\)