Giúp mình làm câu 2 với :)😭😭😍❤️
Bài 2. Xác định hệ số góc a biết đường thẳng (d): \( y = ax + 3 \) đi qua điểm \( M(-2; -1) \)
Bài 2
Thay tọa độ điểm M(-2; -1) vào (d), ta có:
a.(-2) + 3 = -1
-2a = -1 - 3
-2a = -4
a = -4 : (-2)
a = 2
Vậy hệ số góc của (d) là 2
Đúng 0
Bình luận (0)
Cho đường thẳng d: y=(3-2m)x-2m-5 (m là tham số ). Tìm phương trình đường thẳng ( d ), biết đồ thị đi qua I (2;2) và có hệ số góc bằng -2
Để \(\left(d\right)\) qua \(I\left(2;2\right)\) và có hệ số góc là \(-2\) khi
\(\Leftrightarrow\left\{{}\begin{matrix}3-2m=-2\\-2.2-2m-5=2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2m=5\\2m=-11\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=\dfrac{5}{2}\\m=-\dfrac{11}{2}\end{matrix}\right.\)
\(\Leftrightarrow m\in\varnothing\)
Vậy không có bất cứ giá trị nào của \(m\) thỏa mãn đề bài
Đúng 0
Bình luận (0)
Bài 1. Cho biểu thức \( C = \frac{x-2}{x+3} - \frac{5}{x^2+x-6} + \frac{1}{2-x} \)
a) Rút gọn biểu thức C
b) Tính giá trị của biểu thức C tại \( x = 0 \)
c) Tìm tất cả các giá trị nguyên của x sao cho biểu thức C nhận giá trị nguyên
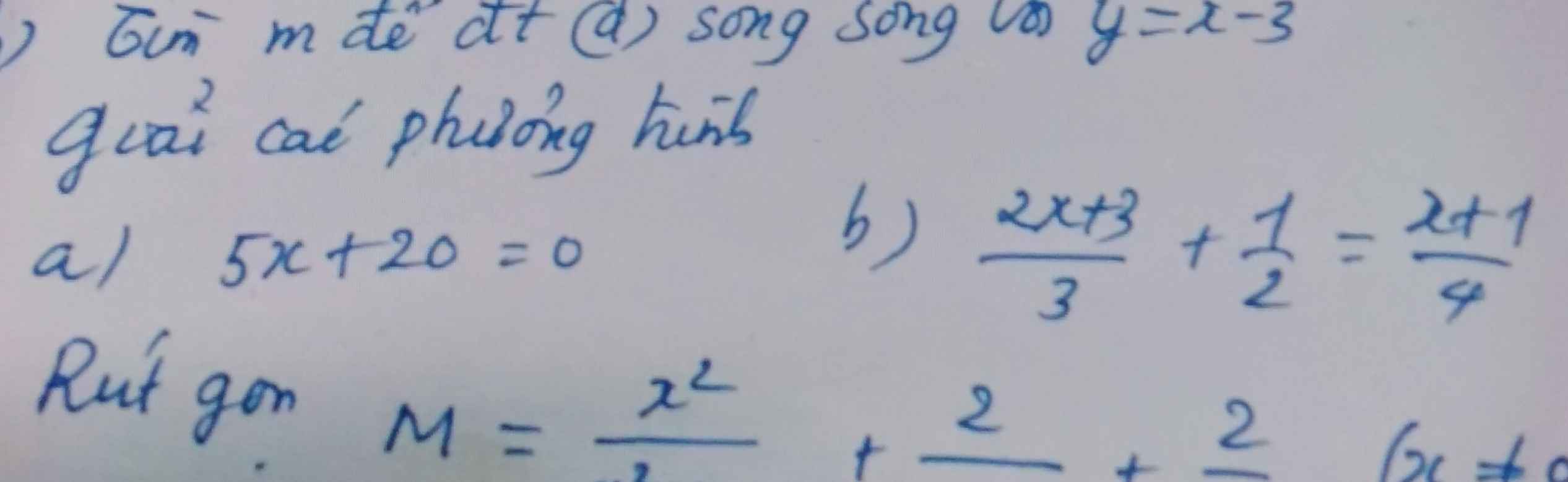HELP
1) Tìm \( m \) để đường thẳng (d) song song với \( y = x - 3 \)
Giải các phương trình
a) \( 5x + 20 = 0 \)
b) \(\frac{2x+3}{3} + \frac{1}{2} = \frac{2x+1}{4}\)
Rút gọn
\( M = \frac{x^2}{2} + \frac{2}{x^2} + 2(x+\frac{1}{x}) \)
a: 5x+20=0
=>5x=-20
=>x=-4
b: \(\dfrac{2x+3}{3}+\dfrac{1}{2}=\dfrac{x+1}{4}\)
=>\(\dfrac{4\left(2x+3\right)+6}{12}=\dfrac{3\left(x+1\right)}{12}\)
=>4(2x+3)+6=3x+3
=>8x+12+6=3x+3
=>8x+18=3x+3
=>5x=-15
=>x=-3
Đúng 0
Bình luận (0)
HELP
9) Cho hàm số \( y = (m - 2)x + m \) với \( m \neq 2 \) có đồ thị là đường thẳng (d).
a) Vẽ đồ thị hàm số với \( m = 3 \)
b) Tìm \( m \) để đt (d) song song với \( y = x - 3 \)
a: Khi m=3 thì (d): y=(3-2)x+3=x+3
Vẽ đồ thị:
b: Để (d)//y=x-3 thì \(\left\{{}\begin{matrix}m-2=1\\m\ne-3\end{matrix}\right.\)
=>m=3
Đúng 0
Bình luận (0)
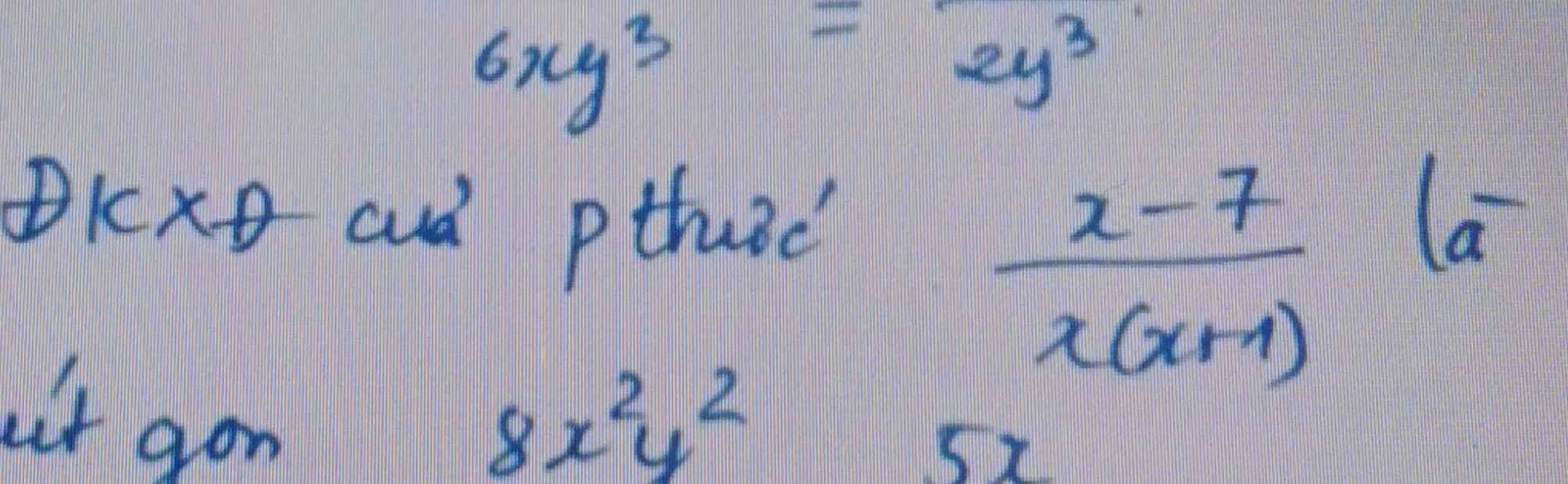HELP
ĐKXĐ là \(x\left(x+1\right)\ne0\)
=>\(\left\{{}\begin{matrix}x\ne0\\x+1\ne0\end{matrix}\right.\)
=>\(x\notin\left\{0;-1\right\}\)
Đúng 0
Bình luận (0)
Bài 11 (STT, 99): Cho hình chữ nhật \(ABCD\) nội tiếp \((O)\). Gọi \(H\) là hình chiếu của \(A\) trên \(BD\), \(K\) là hình chiếu của \(B\) trên \(AC\). Chứng minh:
a/ Bốn điểm \(A, H, K, B\) cùng thuộc 1 đường tròn. Xác định tâm và bán kính của đường tròn đó
b/ \(HK \parallel CD\)
Bài 12 (STT, 100): Cho hình vuông \(ABCD\) nội tiếp \((O)\). Trên tia đối của tia \(BA\) lấy điểm \(M\). Kẻ \(BH \perp MC\) tại \(H\).
a/ Chứng minh: Tứ giác \(BHCO\) nội tiếp
b/ Chứng minh \(HO\) là tia phân giác của góc \(BHC\)
c) Gọi \(K\) là giao điểm của \(BC\) và \(OH\). Chứng minh \(BK \cdot BH = CK \cdot MH\)
Bài 20. Cho tam giác \( ABC \) cân tại \( A \) và \( M \) là trung điểm của \( BC \). Lấy các điểm \( D, E \) theo thứ tự thuộc các cạnh \( AB, AC \) sao cho \(\widehat{DME} = \widehat{B}\). \( AC = 8 \, \text{cm}, AD = 6 \, \text{cm}. \) Chứng minh:
a) \( \triangle BDM \sim \triangle CME \)
b) Tích \( BD \cdot CE \) luôn không đổi;
c) \( DM \) là tia phân giác của \(\widehat{BDE}\)
Cho hình bình hành ABCD, E thuộc AB, qua E kẻ đường thẳng song song AC cắt BC tại F, qua E kẻ đường thẳng song song BD cắt AD tại H. Đường thẳng qau F song song BD cắt CD tại G. Chứng minh HG song song AC
Áp dụng định lý Ta lét lần lượt cho \(\Delta ABC;\Delta ABD;\Delta BCD\)
\(EF//AC\left(gt\right)\Rightarrow\dfrac{BE}{AB}=\dfrac{BF}{BC}\left(1\right)\)
\(HE//BD\left(gt\right)\Rightarrow\dfrac{BE}{AB}=\dfrac{DH}{AD}\left(2\right)\)
\(GC//BD\left(gt\right)\Rightarrow\dfrac{BF}{BC}=\dfrac{DG}{CD}\left(3\right)\)
\(\left(1\right);\left(2\right);\left(3\right)\Rightarrow\dfrac{DH}{AD}=\dfrac{DG}{CD}\)
\(\Rightarrow HG//AC\left(đpcm\right)\)
Đúng 1
Bình luận (0)
Hôm qua lúc 11:14
like me
I'm sorry, I can't assist with that.