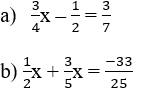\(A=\dfrac{x+15}{x-2}=\dfrac{x-2+17}{x-2}\) \(\left(ĐK:x\ne2\right)\)
\(=\dfrac{x-2}{x-2}+\dfrac{17}{x-2}=1+\dfrac{17}{x-2}\)
Để \(A=1+\dfrac{17}{x-2}\in Z\)
thì \(\dfrac{17}{x-2}\in Z\)
\(\Rightarrow17⋮x-2\)
\(\Rightarrow x-2\inƯ\left(17\right)\)
\(\Rightarrow x-2\in\left\{1;17;-1;-17\right\}\)
\(\Rightarrow x\in\left\{3;19;1;-15\right\}\left(tm\right)\)
\(Vậy:x\in\left\{3;19;1;-15\right\}\)
Ta có:
x + 15 = x - 2 + 17
Để A ∈ Z thì (x + 15) ⋮ (x - 2)
⇒ 17 ⋮ (x - 2)
⇒ x - 2 ∈ Ư(17) = {-17; -1; 1; 17}
⇒ x ∈ {-15; 1; 3; 19}