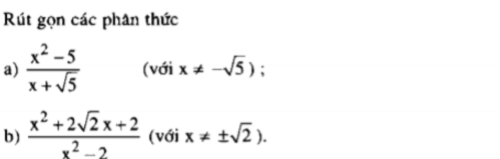Câu 10:
a) \(B=\left(\dfrac{\sqrt{a}-2}{\sqrt{a}+2}+\dfrac{\sqrt{a}+2}{\sqrt{a}-2}-\dfrac{4a}{4-a}\right):\dfrac{3a+4}{\sqrt{a}+2}\)
\(=\dfrac{\left(\sqrt{a}-2\right)^2+\left(\sqrt{a}+2\right)^2+4a}{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}.\dfrac{\sqrt{a}+2}{3a+4}=\dfrac{a-4\sqrt{a}+4+a+4\sqrt{a}+4+4a}{\left(\sqrt{a}-2\right)\left(3a+4\right)}=\dfrac{6a+8}{\left(\sqrt{a}-2\right)\left(3a+4\right)}=\dfrac{2\left(3a+4\right)}{\left(\sqrt{a}-2\right)\left(3a+4\right)}=\dfrac{2}{\sqrt{a}-2}\)
b)Ta có: \(\sqrt{a}-2\ge-2\Rightarrow\dfrac{2}{\sqrt{a}-2}\le-1\)
Để \(B< -1\) thì \(\dfrac{2}{\sqrt{a}-2}\ne-1\Leftrightarrow\sqrt{a}-2\ne-2\Leftrightarrow a\ne0\) và \(a\ge0,a\ne4\)
Câu 11:
a) \(P=\left(\dfrac{\sqrt{x}-4x}{1-4x}-1\right):\left(\dfrac{1+2x}{1-4x}-\dfrac{2\sqrt{x}}{1-2\sqrt{x}}-1\right)=\dfrac{\sqrt{x}-4x-1+4x}{1-4x}:\dfrac{1+2x-2\sqrt{x}\left(1+2\sqrt{x}\right)-1+4x}{1-4x}=\dfrac{\sqrt{x}-1}{1-4x}.\dfrac{1-4x}{2x-2\sqrt{x}}=\dfrac{\sqrt{x}-1}{2\sqrt{x}\left(\sqrt{x}-1\right)}=\dfrac{1}{2\sqrt{x}}\)
b) Thay x=\(3-2\sqrt{2}\) vào P ta được:
\(P=\dfrac{1}{2\sqrt{x}}=\dfrac{1}{2\sqrt{3-2\sqrt{2}}}=\dfrac{1}{2\left(\sqrt{\sqrt{2}-1}\right)^2}=\dfrac{1}{2\left(\sqrt{2}-1\right)}=\dfrac{1+\sqrt{2}}{2}\)
c) \(P=\dfrac{1}{2\sqrt{x}}>\dfrac{1}{2}\Leftrightarrow2\sqrt{x}< 2\Rightarrow0< x< 1\) và \(x\ne\dfrac{1}{4}\)


















 Mọi người giúp mình câu 3, câu 4 với. Mình cảm ơn ạ. Mình đang cần gấp lắm!!!
Mọi người giúp mình câu 3, câu 4 với. Mình cảm ơn ạ. Mình đang cần gấp lắm!!!