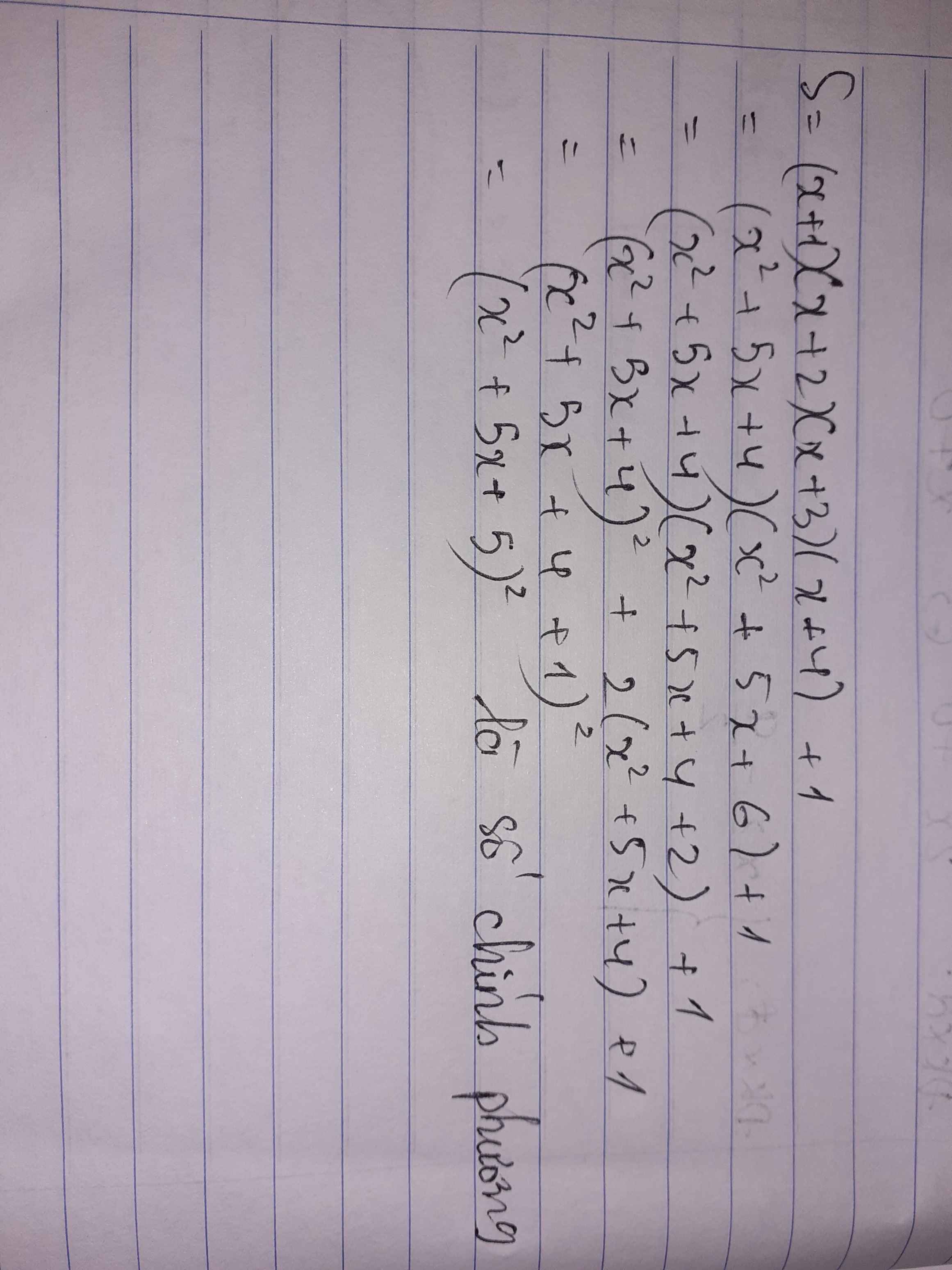Question

CMR:

S=(x+1)(x+2)(x+3)(x+4)+1 luôn luôn là 1 số chính phương ∀ x ∈ Z

Answer 1

Answer 2

\(S=\left(x+1\right)\left(x+4\right)\left(x+2\right)\left(x+3\right)+1\)

\(=\left(x^2+5x+4\right)\left(x^2+5x+6\right)+1\)

\(=\left(x^2+5x+4\right)\left(x^2+5x+4+2\right)+1\)

\(=\left(x^2+5x+4\right)^2+2\left(x^2+5x+4\right)+1\)

\(=\left(x^2+5x+4+1\right)^2\)

\(=\left(x^2+5x+5\right)^2\) là SCP (đpcm)

Answer 3

\(S=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)+1=\left(x+1\right)\left(x+4\right)\left(x+2\right)\left(x+3\right)+1\)

\(=\left(x^2+5x+4\right)\left(x^2+5x+6\right)+1\)

Đặt \(t=x^2+5x+5\Rightarrow\) pt trở thành \(\left(t-1\right)\left(t+1\right)+1=t^2-1+1=t^2\)

\(=\left(x^2+5x+5\right)^2\)

Vì \(x\in Z\Rightarrow x^2+5x+5\in Z\Rightarrow\left(x^2+5x+5\right)^2\) là số chính phương

Answer 4

S = (x + 1)(x + 2)(x + 3)(x + 4) + 1

= [(x + 1)(x + 4)][(x + 2)(x + 3)] + 1

= (x^2 + 5x + 4)(x^2 + 5x +6) + 1

Đặt t = x^2 + 5x + 5 (do x ∈ Z nên t ∈ Z)

⇒ S = (t - 1)(t + 1) + 1

= t^2 - 1 + 1

= t^2 là số chính phương do t ∈ Z

⇒ S là số cp với mọi x ∈ Z (đpcm)

Answer 5

Ta có: \(S=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)+1\)

\(=\left(x^2+5x+4\right)\left(x^2+5x+6\right)+1\)

\(=\left(x^2+5x\right)^2+10\left(x^2+5x\right)+24+1\)

\(=\left(x^2+5x\right)^2+10\left(x^2+5x\right)+25\)

\(=\left(x^2+5x+5\right)^2\)